Fundamentals of Probability: A First Course. Anirban DasGupta
|
|
|
- Βασίλης Κρεστενίτης
- 8 χρόνια πριν
- Προβολές:
Transcript
1 Fundamentals of Probability: A First Course Anirban DasGupta
2 Contents 1 Introducing Probability ExperimentsandSampleSpaces Set Theory Notation and Axioms of Probability How to Interpret a Probability Calculating Probabilities ManualCounting GeneralCountingMethods InclusionExclusionFormula Bounds on the Probability of a Union Synopsis Exercises References The Birthday and the Matching Problem TheBirthdayProblem *Stirling sapproximation TheMatchingProblem Synopsis Exercises References Conditional Probability and Independence BasicFormulasandFirstExamples MoreAdvancedExamples IndependentEvents BayesTheorem Synopsis Exercises Integer Valued and Discrete Random Variables MassFunction CDFandMedianofaRandomVariable FunctionsofaRandomVariable Independence of Random Variables ExpectedValueofaDiscreteRandomVariable I
3 4.4 BasicPropertiesofExpectations Illustrative Examples Using Indicator Variables to Calculate Expectations The Tail Sum Method for Calculating Expectations Variance, Moments, and Basic Inequalities Illustrative Examples Variance of a Sum of Independent Random Variables Utility of µ, σ assummaries Chebyshev s Inequality and Weak Law of Large Numbers *BetterInequalities Other Fundamental Moment Inequalities *ApplyingMomentInequalities TruncatedDistributions Synopsis Exercises References Generating Functions GeneratingFunctions Moment Generating Functions and Cumulants Cumulants Synopsis Exercises References Standard Discrete Distributions IntroductiontoSpecialDistributions DiscreteUniformDistribution BinomialDistribution Geometric and Negative Binomial Distribution HypergeometricDistribution PoissonDistribution Mean Absolute Deviation and the Mode PoissonApproximationtoBinomial MiscellaneousPoissonApproximations Benford slaw II
4 6.10 DistributionofSumsandDifferences *DistributionofDifferences DiscreteDoesNotMeanIntegerValued Synopsis Exercises References Continuous Random Variables TheDensityFunctionandtheCDF Quantiles Generating New Distributions from Old Normal and Other Symmetric Unimodal Densities Functions of a Continuous Random Variable QuantileTransformation Cauchydensity ExpectationofFunctionsandMoments The Tail Probability Method for Calculating Expectations SurvivalandHazardRate *MomentsandtheTail Moment Generating Function and Fundamental Tail Inequalities *Chernoff-BernsteinInequality *Lugosi simprovedinequality Jensen and Other Moment Inequalities and a Paradox Synopsis Exercises References Some Special Continuous Distributions UniformDistribution ExponentialandWeibullDistributions GammaandInverseGammaDistributions BetaDistribution ExtremeValueDistributions * Exponential Density and the Poisson Process Synopsis Exercises III
5 8.9 References Normal Distribution DefinitionandBasicProperties WorkingwithaNormalTable Additional Examples and the Lognormal Density SumsofIndependentNormalVariables Mills Ratio and Approximations for the Standard Normal CDF Synopsis Exercises References Normal Approximations and Central Limit Theorem SomeMotivatingExamples CentralLimitTheorem NormalApproximationtoBinomial ContinuityCorrection ANewRuleofThumb ExamplesoftheGeneralCLT NormalApproximationtoPoissonandGamma Convergence of Densities and Higher Order Approximations *RefinedApproximations Practical Recommendations for Normal Approximations Synopsis Exercises References Multivariate Discrete Distributions Bivariate Joint Distributions and Expectations of Functions Conditional Distributions and Conditional Expectations Examples on Conditional Distributions and Expectations Using Conditioning to Evaluate Mean and Variance CovarianceandCorrelation MultivariateCase JointMGF MultinomialDistribution IV
6 11.6 Synopsis Exercises Multidimensional Densities JointDensityFunctionandItsRole ExpectationofFunctions BivariateNormal Conditional Densities and Expectations Examples on Conditional Densities and Expectations Bivariate Normal Conditional Distributions OrderStatistics BasicDistributionTheory * More Advanced Distribution Theory Synopsis Exercises References Convolutions and Transformations ConvolutionsandExamples Products and Quotients and the t and F Distribution Transformations ApplicationsofJacobianFormula PolarCoordinatesinTwoDimensions Synopsis Exercises References Markov Chains and Applications NotationandBasicDefinitions Chapman-Kolmogorov Equation CommunicatingClasses Gambler sruin FirstPassage,RecurrenceandTransience Long Run Evolution and Stationary Distributions Synopsis Exercises V
7 14.9 References Urn Models in Physics and Genetics Stirling Numbers and Their Basic Properties UrnModelsinQuantumMechanics *PoissonApproximations Pólya surn Pólya-EggenbergerDistribution * de Finetti s Theorem and PólyaUrns UrnModelsinGenetics Wright-FisherModel TimeuntilAlleleUniformity MutationandHoppe surn *TheEwensSamplingFormula Synopsis Exercises References Appendix I: Supplementary Homework and Practice Problems WordProblems True-FalseProblems Appendix II GlossaryofSymbols FormulaSummaries Moments and MGFs of Common Distributions Useful Mathematical Formulas UsefulCalculusFacts Tables NormalTable PoissonTable VI
APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 651 APPENDIX B. BIBLIOGRAPHY 677 APPENDIX C. ANSWERS TO SELECTED EXERCISES 679
 APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 1 Table I Summary of Common Probability Distributions 2 Table II Cumulative Standard Normal Distribution Table III Percentage Points, 2 of the Chi-Squared
APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 1 Table I Summary of Common Probability Distributions 2 Table II Cumulative Standard Normal Distribution Table III Percentage Points, 2 of the Chi-Squared
Statistics 104: Quantitative Methods for Economics Formula and Theorem Review
 Harvard College Statistics 104: Quantitative Methods for Economics Formula and Theorem Review Tommy MacWilliam, 13 tmacwilliam@college.harvard.edu March 10, 2011 Contents 1 Introduction to Data 5 1.1 Sample
Harvard College Statistics 104: Quantitative Methods for Economics Formula and Theorem Review Tommy MacWilliam, 13 tmacwilliam@college.harvard.edu March 10, 2011 Contents 1 Introduction to Data 5 1.1 Sample
Biostatistics for Health Sciences Review Sheet
 Biostatistics for Health Sciences Review Sheet http://mathvault.ca June 1, 2017 Contents 1 Descriptive Statistics 2 1.1 Variables.............................................. 2 1.1.1 Qualitative........................................
Biostatistics for Health Sciences Review Sheet http://mathvault.ca June 1, 2017 Contents 1 Descriptive Statistics 2 1.1 Variables.............................................. 2 1.1.1 Qualitative........................................
5.4 The Poisson Distribution.
 The worst thing you can do about a situation is nothing. Sr. O Shea Jackson 5.4 The Poisson Distribution. Description of the Poisson Distribution Discrete probability distribution. The random variable
The worst thing you can do about a situation is nothing. Sr. O Shea Jackson 5.4 The Poisson Distribution. Description of the Poisson Distribution Discrete probability distribution. The random variable
Έντυπο Καταγραφής Πληροφοριών και Συγκέντρωσης Εκπαιδευτικού Υλικού για τα Ανοικτά Μαθήματα
 Έντυπο Καταγραφής Πληροφοριών και Συγκέντρωσης Εκπαιδευτικού Υλικού για τα Ανοικτά Μαθήματα Έκδοση: 1.02, Απρίλιος 2014 Συντάκτης: Δρ. Παντελής Μπαλαούρας, Καθ. Λάζαρος Μεράκος (ΕΚΠΑ) Προσαρμογή: Αν. Καθ.
Έντυπο Καταγραφής Πληροφοριών και Συγκέντρωσης Εκπαιδευτικού Υλικού για τα Ανοικτά Μαθήματα Έκδοση: 1.02, Απρίλιος 2014 Συντάκτης: Δρ. Παντελής Μπαλαούρας, Καθ. Λάζαρος Μεράκος (ΕΚΠΑ) Προσαρμογή: Αν. Καθ.
Aquinas College. Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET
 Aquinas College Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET Pearson Edexcel Level 3 Advanced Subsidiary and Advanced GCE in Mathematics and Further Mathematics Mathematical
Aquinas College Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET Pearson Edexcel Level 3 Advanced Subsidiary and Advanced GCE in Mathematics and Further Mathematics Mathematical
ON NEGATIVE MOMENTS OF CERTAIN DISCRETE DISTRIBUTIONS
 Pa J Statist 2009 Vol 25(2), 135-140 ON NEGTIVE MOMENTS OF CERTIN DISCRETE DISTRIBUTIONS Masood nwar 1 and Munir hmad 2 1 Department of Maematics, COMSTS Institute of Information Technology, Islamabad,
Pa J Statist 2009 Vol 25(2), 135-140 ON NEGTIVE MOMENTS OF CERTIN DISCRETE DISTRIBUTIONS Masood nwar 1 and Munir hmad 2 1 Department of Maematics, COMSTS Institute of Information Technology, Islamabad,
255 (log-normal distribution) 83, 106, 239 (malus) 26 - (Belgian BMS, Markovian presentation) 32 (median premium calculation principle) 186 À / Á (goo
 (absolute loss function)186 - (posterior structure function)163 - (a priori rating variables)25 (Bayes scale) 178 (bancassurance)233 - (beta distribution)203, 204 (high deductible)218 (bonus)26 ( ) (total
(absolute loss function)186 - (posterior structure function)163 - (a priori rating variables)25 (Bayes scale) 178 (bancassurance)233 - (beta distribution)203, 204 (high deductible)218 (bonus)26 ( ) (total
Bayesian statistics. DS GA 1002 Probability and Statistics for Data Science.
 Bayesian statistics DS GA 1002 Probability and Statistics for Data Science http://www.cims.nyu.edu/~cfgranda/pages/dsga1002_fall17 Carlos Fernandez-Granda Frequentist vs Bayesian statistics In frequentist
Bayesian statistics DS GA 1002 Probability and Statistics for Data Science http://www.cims.nyu.edu/~cfgranda/pages/dsga1002_fall17 Carlos Fernandez-Granda Frequentist vs Bayesian statistics In frequentist
HMY 429: Εισαγωγή στην Επεξεργασία Ψηφιακών. Χρόνου (Ι)
 HMY 429: Εισαγωγή στην Επεξεργασία Ψηφιακών Σημάτων Διάλεξη 5: Στοχαστικά/Τυχαία Σήματα Διακριτού Διάλεξη 5: Στοχαστικά/Τυχαία Σήματα Διακριτού Χρόνου (Ι) Στοχαστικά σήματα Στα προηγούμενα: Ντετερμινιστικά
HMY 429: Εισαγωγή στην Επεξεργασία Ψηφιακών Σημάτων Διάλεξη 5: Στοχαστικά/Τυχαία Σήματα Διακριτού Διάλεξη 5: Στοχαστικά/Τυχαία Σήματα Διακριτού Χρόνου (Ι) Στοχαστικά σήματα Στα προηγούμενα: Ντετερμινιστικά
Probability and Random Processes (Part II)
 Probability and Random Processes (Part II) 1. If the variance σ x of d(n) = x(n) x(n 1) is one-tenth the variance σ x of a stationary zero-mean discrete-time signal x(n), then the normalized autocorrelation
Probability and Random Processes (Part II) 1. If the variance σ x of d(n) = x(n) x(n 1) is one-tenth the variance σ x of a stationary zero-mean discrete-time signal x(n), then the normalized autocorrelation
Harold s Statistics Probability Density Functions Cheat Sheet 30 May PDF Selection Tree to Describe a Single Population
 Harold s Statistics Probability Density Functions Cheat Sheet 30 May 2016 PDF Selection Tree to Describe a Single Population Qualitative Quantitative Copyright 2016 by Harold Toomey, WyzAnt Tutor 1 Discrete
Harold s Statistics Probability Density Functions Cheat Sheet 30 May 2016 PDF Selection Tree to Describe a Single Population Qualitative Quantitative Copyright 2016 by Harold Toomey, WyzAnt Tutor 1 Discrete
Covariance and Pseudo-Covariance of Complex Uncertain Variables
 Covariance and Pseudo-Covariance of Complex Uncertain Variables Rong Gao 1, Hamed Ahmadzade 2, Mojtaba Esfahani 3 1. School of Economics and Management, Hebei University of Technology, Tianjin 341, China
Covariance and Pseudo-Covariance of Complex Uncertain Variables Rong Gao 1, Hamed Ahmadzade 2, Mojtaba Esfahani 3 1. School of Economics and Management, Hebei University of Technology, Tianjin 341, China
Math 6 SL Probability Distributions Practice Test Mark Scheme
 Math 6 SL Probability Distributions Practice Test Mark Scheme. (a) Note: Award A for vertical line to right of mean, A for shading to right of their vertical line. AA N (b) evidence of recognizing symmetry
Math 6 SL Probability Distributions Practice Test Mark Scheme. (a) Note: Award A for vertical line to right of mean, A for shading to right of their vertical line. AA N (b) evidence of recognizing symmetry
ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 4, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 2
 ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 4, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 5.4: Στατιστικοί Μέσοι Όροι 5.5 Στοχαστικές Ανελίξεις (Stochastic Processes)
ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 4, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 5.4: Στατιστικοί Μέσοι Όροι 5.5 Στοχαστικές Ανελίξεις (Stochastic Processes)
Wishart α-determinant, α-hafnian
 Wishart α-determinant, α-hafnian (, JST CREST) (, JST CREST), Wishart,. ( )Wishart,. determinant Hafnian analogue., ( )Wishart,. 1 Introduction, Wishart. p ν M = (µ 1,..., µ ν ) = (µ ij ) i=1,...,p p p
Wishart α-determinant, α-hafnian (, JST CREST) (, JST CREST), Wishart,. ( )Wishart,. determinant Hafnian analogue., ( )Wishart,. 1 Introduction, Wishart. p ν M = (µ 1,..., µ ν ) = (µ ij ) i=1,...,p p p
FORMULAS FOR STATISTICS 1
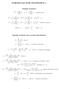 FORMULAS FOR STATISTICS 1 X = 1 n Sample statistics X i or x = 1 n x i (sample mean) S 2 = 1 n 1 s 2 = 1 n 1 (X i X) 2 = 1 n 1 (x i x) 2 = 1 n 1 Xi 2 n n 1 X 2 x 2 i n n 1 x 2 or (sample variance) E(X)
FORMULAS FOR STATISTICS 1 X = 1 n Sample statistics X i or x = 1 n x i (sample mean) S 2 = 1 n 1 s 2 = 1 n 1 (X i X) 2 = 1 n 1 (x i x) 2 = 1 n 1 Xi 2 n n 1 X 2 x 2 i n n 1 x 2 or (sample variance) E(X)
Solution Series 9. i=1 x i and i=1 x i.
 Lecturer: Prof. Dr. Mete SONER Coordinator: Yilin WANG Solution Series 9 Q1. Let α, β >, the p.d.f. of a beta distribution with parameters α and β is { Γ(α+β) Γ(α)Γ(β) f(x α, β) xα 1 (1 x) β 1 for < x
Lecturer: Prof. Dr. Mete SONER Coordinator: Yilin WANG Solution Series 9 Q1. Let α, β >, the p.d.f. of a beta distribution with parameters α and β is { Γ(α+β) Γ(α)Γ(β) f(x α, β) xα 1 (1 x) β 1 for < x
ΕΥΡΕΤΗΡΙΟ ΕΛΛΗΝΙΚΩΝ ΟΡΩΝ
 ΕΥΡΕΤΗΡΙΟ ΕΛΛΗΝΙΚΩΝ ΟΡΩΝ Α Αθροιστική συνάρτηση κατανομής, 70, 317 σχετική συχνότητα, 321 Αθροιστικός κανόνας, 57, 60 Ακολουθία δοκιμών Bernoulli, 118 Ακρίβεια, 501 Αμεροληψία, 252 Αναμενόμενη τιμή, 73
ΕΥΡΕΤΗΡΙΟ ΕΛΛΗΝΙΚΩΝ ΟΡΩΝ Α Αθροιστική συνάρτηση κατανομής, 70, 317 σχετική συχνότητα, 321 Αθροιστικός κανόνας, 57, 60 Ακολουθία δοκιμών Bernoulli, 118 Ακρίβεια, 501 Αμεροληψία, 252 Αναμενόμενη τιμή, 73
BarChart y 1, y 2, makes a bar chart with bar lengths y 1, y 2,.
 In[]:= In[]:= In[3]:= In[4]:= In[5]:= Out[5]= r : Random ri : Random Integer rdice : Random Integer,, 6 disp : Export "t.ps",, "EPS" & list Table rdice, 0 5,, 4, 6,, 3,, 3, 4,, 6, 4, 6,,, 6, 6,, 3, In[6]:=
In[]:= In[]:= In[3]:= In[4]:= In[5]:= Out[5]= r : Random ri : Random Integer rdice : Random Integer,, 6 disp : Export "t.ps",, "EPS" & list Table rdice, 0 5,, 4, 6,, 3,, 3, 4,, 6, 4, 6,,, 6, 6,, 3, In[6]:=
ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 3, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 1
 ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 3, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 1 5.1: Εισαγωγή 5.2: Πιθανότητες 5.3: Τυχαίες Μεταβλητές καθ. Βασίλης Μάγκλαρης
ΣΤΟΧΑΣΤΙΚΑ ΣΥΣΤΗΜΑΤΑ & ΕΠΙΚΟΙΝΩΝΙΕΣ 1o Τμήμα (Α - Κ): Αμφιθέατρο 3, Νέα Κτίρια ΣΗΜΜΥ Θεωρία Πιθανοτήτων & Στοχαστικές Ανελίξεις - 1 5.1: Εισαγωγή 5.2: Πιθανότητες 5.3: Τυχαίες Μεταβλητές καθ. Βασίλης Μάγκλαρης
χ 2 test ανεξαρτησίας
 χ 2 test ανεξαρτησίας Καθηγητής Ι. Κ. ΔΗΜΗΤΡΙΟΥ demetri@econ.uoa.gr 7.2 Το χ 2 Τεστ Ανεξαρτησίας Tο χ 2 τεστ ανεξαρτησίας (όπως και η παλινδρόμηση) είναι στατιστικά εργαλεία για τον εντοπισμό σχέσεων μεταξύ
χ 2 test ανεξαρτησίας Καθηγητής Ι. Κ. ΔΗΜΗΤΡΙΟΥ demetri@econ.uoa.gr 7.2 Το χ 2 Τεστ Ανεξαρτησίας Tο χ 2 τεστ ανεξαρτησίας (όπως και η παλινδρόμηση) είναι στατιστικά εργαλεία για τον εντοπισμό σχέσεων μεταξύ
Anti-Final CS/SE 3341 SOLUTIONS
 CS/SE 3341 SOLUTIONS Anti-Final 1. Users call help desk every 15 minutes, on the average. There is one help desk specialist on duty, and her average service time is 9 minutes. Modeling the help desk as
CS/SE 3341 SOLUTIONS Anti-Final 1. Users call help desk every 15 minutes, on the average. There is one help desk specialist on duty, and her average service time is 9 minutes. Modeling the help desk as
Table A.1 Random numbers (section 1)
 A Tables Table Contents Page A.1 Random numbers 696 A.2 Orthogonal polynomial trend contrast coefficients 702 A.3 Standard normal distribution 703 A.4 Student s t-distribution 704 A.5 Chi-squared distribution
A Tables Table Contents Page A.1 Random numbers 696 A.2 Orthogonal polynomial trend contrast coefficients 702 A.3 Standard normal distribution 703 A.4 Student s t-distribution 704 A.5 Chi-squared distribution
Fundamentals of Signals, Systems and Filtering
 Fundamentals of Signals, Systems and Filtering Brett Ninness c 2000-2005, Brett Ninness, School of Electrical Engineering and Computer Science The University of Newcastle, Australia. 2 c Brett Ninness
Fundamentals of Signals, Systems and Filtering Brett Ninness c 2000-2005, Brett Ninness, School of Electrical Engineering and Computer Science The University of Newcastle, Australia. 2 c Brett Ninness
Wrapped Geometric Stable Distributions
 Wrapped Geometric Stable Distributions Sophy Jacob Study on circular distributions Thesis. Department of Statistics, University of Calicut, 0 Chapter 3 Wrapped Geometric Stable Distributions 3. Introduction
Wrapped Geometric Stable Distributions Sophy Jacob Study on circular distributions Thesis. Department of Statistics, University of Calicut, 0 Chapter 3 Wrapped Geometric Stable Distributions 3. Introduction
List MF20. List of Formulae and Statistical Tables. Cambridge Pre-U Mathematics (9794) and Further Mathematics (9795)
 List MF0 List of Formulae and Statistical Tables Cambridge Pre-U Mathematics (979) and Further Mathematics (979) For use from 07 in all aers for the above syllabuses. CST7 Mensuration Surface area of shere
List MF0 List of Formulae and Statistical Tables Cambridge Pre-U Mathematics (979) and Further Mathematics (979) For use from 07 in all aers for the above syllabuses. CST7 Mensuration Surface area of shere
Limit theorems under sublinear expectations and probabilities
 Limit theorems under sublinear expectations and probabilities Xinpeng LI Shandong University & Université Paris 1 Young Researchers Meeting on BSDEs, Numerics and Finance 4 July, Oxford 1 / 25 Outline
Limit theorems under sublinear expectations and probabilities Xinpeng LI Shandong University & Université Paris 1 Young Researchers Meeting on BSDEs, Numerics and Finance 4 July, Oxford 1 / 25 Outline
Asymptotic distribution of MLE
 Asymptotic distribution of MLE Theorem Let {X t } be a causal and invertible ARMA(p,q) process satisfying Φ(B)X = Θ(B)Z, {Z t } IID(0, σ 2 ). Let ( ˆφ, ˆϑ) the values that minimize LL n (φ, ϑ) among those
Asymptotic distribution of MLE Theorem Let {X t } be a causal and invertible ARMA(p,q) process satisfying Φ(B)X = Θ(B)Z, {Z t } IID(0, σ 2 ). Let ( ˆφ, ˆϑ) the values that minimize LL n (φ, ϑ) among those
lecture 10: the em algorithm (contd)
 lecture 10: the em algorithm (contd) STAT 545: Intro. to Computational Statistics Vinayak Rao Purdue University September 24, 2018 Exponential family models Consider a space X. E.g. R, R d or N. ϕ(x) =
lecture 10: the em algorithm (contd) STAT 545: Intro. to Computational Statistics Vinayak Rao Purdue University September 24, 2018 Exponential family models Consider a space X. E.g. R, R d or N. ϕ(x) =
519.22(07.07) 78 : ( ) /.. ; c (07.07) , , 2008
 .. ( ) 2008 519.22(07.07) 78 : ( ) /.. ;. : -, 2008. 38 c. ( ) STATISTICA.,. STATISTICA.,. 519.22(07.07),.., 2008.., 2008., 2008 2 ... 4 1...5...5 2...14...14 3...27...27 3 ,, -. " ", :,,,... STATISTICA.,,,.
.. ( ) 2008 519.22(07.07) 78 : ( ) /.. ;. : -, 2008. 38 c. ( ) STATISTICA.,. STATISTICA.,. 519.22(07.07),.., 2008.., 2008., 2008 2 ... 4 1...5...5 2...14...14 3...27...27 3 ,, -. " ", :,,,... STATISTICA.,,,.
An Inventory of Continuous Distributions
 Appendi A An Inventory of Continuous Distributions A.1 Introduction The incomplete gamma function is given by Also, define Γ(α; ) = 1 with = G(α; ) = Z 0 Z 0 Z t α 1 e t dt, α > 0, >0 t α 1 e t dt, α >
Appendi A An Inventory of Continuous Distributions A.1 Introduction The incomplete gamma function is given by Also, define Γ(α; ) = 1 with = G(α; ) = Z 0 Z 0 Z t α 1 e t dt, α > 0, >0 t α 1 e t dt, α >
Approximations for the distribution of the discrete scan statistics when the scanning window has arbitrary shape
 Approximations for the distribution of the discrete scan statistics when the scanning window has arbitrary shape Alexandru Am rioarei National Institute of Research and Development for Biological Sciences
Approximations for the distribution of the discrete scan statistics when the scanning window has arbitrary shape Alexandru Am rioarei National Institute of Research and Development for Biological Sciences
Statistical Inference I Locally most powerful tests
 Statistical Inference I Locally most powerful tests Shirsendu Mukherjee Department of Statistics, Asutosh College, Kolkata, India. shirsendu st@yahoo.co.in So far we have treated the testing of one-sided
Statistical Inference I Locally most powerful tests Shirsendu Mukherjee Department of Statistics, Asutosh College, Kolkata, India. shirsendu st@yahoo.co.in So far we have treated the testing of one-sided
HMY 795: Αναγνώριση Προτύπων. Διάλεξη 2
 HMY 795: Αναγνώριση Προτύπων Διάλεξη 2 Επισκόπηση θεωρίας πιθανοτήτων Θεωρία πιθανοτήτων Τυχαία μεταβλητή: Μεταβλητή της οποίας δε γνωρίζουμε με βεβαιότητα την τιμή (αντίθετα με τις ντετερμινιστικές μεταβλητές)
HMY 795: Αναγνώριση Προτύπων Διάλεξη 2 Επισκόπηση θεωρίας πιθανοτήτων Θεωρία πιθανοτήτων Τυχαία μεταβλητή: Μεταβλητή της οποίας δε γνωρίζουμε με βεβαιότητα την τιμή (αντίθετα με τις ντετερμινιστικές μεταβλητές)
Solutions to Exercise Sheet 5
 Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
When using the normal approximation to a discrete distribution, use the continuity correction.
 Tables for Exam C The reading material for Exam C includes a variety of textbooks. Each text has a set of probability distributions that are used in its readings. For those distributions used in more than
Tables for Exam C The reading material for Exam C includes a variety of textbooks. Each text has a set of probability distributions that are used in its readings. For those distributions used in more than
1. A fully continuous 20-payment years, 30-year term life insurance of 2000 is issued to (35). You are given n A 1
 Chapter 7: Exercises 1. A fully continuous 20-payment years, 30-year term life insurance of 2000 is issued to (35). You are given n A 1 35+n:30 n a 35+n:20 n 0 0.068727 11.395336 10 0.097101 7.351745 25
Chapter 7: Exercises 1. A fully continuous 20-payment years, 30-year term life insurance of 2000 is issued to (35). You are given n A 1 35+n:30 n a 35+n:20 n 0 0.068727 11.395336 10 0.097101 7.351745 25
Introduction to the ML Estimation of ARMA processes
 Introduction to the ML Estimation of ARMA processes Eduardo Rossi University of Pavia October 2013 Rossi ARMA Estimation Financial Econometrics - 2013 1 / 1 We consider the AR(p) model: Y t = c + φ 1 Y
Introduction to the ML Estimation of ARMA processes Eduardo Rossi University of Pavia October 2013 Rossi ARMA Estimation Financial Econometrics - 2013 1 / 1 We consider the AR(p) model: Y t = c + φ 1 Y
Supplementary Appendix
 Supplementary Appendix Measuring crisis risk using conditional copulas: An empirical analysis of the 2008 shipping crisis Sebastian Opitz, Henry Seidel and Alexander Szimayer Model specification Table
Supplementary Appendix Measuring crisis risk using conditional copulas: An empirical analysis of the 2008 shipping crisis Sebastian Opitz, Henry Seidel and Alexander Szimayer Model specification Table
Monte Carlo Methods. for Econometric Inference I. Institute on Computational Economics. July 19, John Geweke, University of Iowa
 Monte Carlo Methods for Econometric Inference I Institute on Computational Economics July 19, 2006 John Geweke, University of Iowa Monte Carlo Methods for Econometric Inference I 1 Institute on Computational
Monte Carlo Methods for Econometric Inference I Institute on Computational Economics July 19, 2006 John Geweke, University of Iowa Monte Carlo Methods for Econometric Inference I 1 Institute on Computational
A Bonus-Malus System as a Markov Set-Chain. Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics
 A Bonus-Malus System as a Markov Set-Chain Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics Contents 1. Markov set-chain 2. Model of bonus-malus system 3. Example 4. Conclusions
A Bonus-Malus System as a Markov Set-Chain Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics Contents 1. Markov set-chain 2. Model of bonus-malus system 3. Example 4. Conclusions
Berry-Esseen Theorem. Po-Ning Chen, Professor. Institute of Communications Engineering. National Chiao Tung University. Hsin Chu, Taiwan 30010, R.O.C.
 Berry-Esseen heorem Po-Ning Chen, Professor Institute of Communications Engineering National Chiao ung University Hsin Chu, aiwan 30010, R.O.C. Historical aspects BE- he central limit theorem CL concerns
Berry-Esseen heorem Po-Ning Chen, Professor Institute of Communications Engineering National Chiao ung University Hsin Chu, aiwan 30010, R.O.C. Historical aspects BE- he central limit theorem CL concerns
Problem Set 3: Solutions
 CMPSCI 69GG Applied Information Theory Fall 006 Problem Set 3: Solutions. [Cover and Thomas 7.] a Define the following notation, C I p xx; Y max X; Y C I p xx; Ỹ max I X; Ỹ We would like to show that C
CMPSCI 69GG Applied Information Theory Fall 006 Problem Set 3: Solutions. [Cover and Thomas 7.] a Define the following notation, C I p xx; Y max X; Y C I p xx; Ỹ max I X; Ỹ We would like to show that C
MOTORCAR INSURANCE I
 MOTORCAR INSURANCE I I Acc. II Acc. III Acc. Sex Year Month Day 19970602 0 0 M 1966 4 11 19820101 19840801 0 M 1926 3 25 19820801 19840712 0 F 1952 2 19 19781222 19810507 0 M 1952 3 23 19821110 19870614
MOTORCAR INSURANCE I I Acc. II Acc. III Acc. Sex Year Month Day 19970602 0 0 M 1966 4 11 19820101 19840801 0 M 1926 3 25 19820801 19840712 0 F 1952 2 19 19781222 19810507 0 M 1952 3 23 19821110 19870614
Figure A.2: MPC and MPCP Age Profiles (estimating ρ, ρ = 2, φ = 0.03)..
 Supplemental Material (not for publication) Persistent vs. Permanent Income Shocks in the Buffer-Stock Model Jeppe Druedahl Thomas H. Jørgensen May, A Additional Figures and Tables Figure A.: Wealth and
Supplemental Material (not for publication) Persistent vs. Permanent Income Shocks in the Buffer-Stock Model Jeppe Druedahl Thomas H. Jørgensen May, A Additional Figures and Tables Figure A.: Wealth and
Stationary Stochastic Processes Table of Formulas, 2017
 Stationary Stochastic Processes, 07 Stationary Stochastic Processes Table of Formulas, 07 Basics of probability theory The following is valid for probabilities: P(Ω), where Ω is all possible outcomes 0
Stationary Stochastic Processes, 07 Stationary Stochastic Processes Table of Formulas, 07 Basics of probability theory The following is valid for probabilities: P(Ω), where Ω is all possible outcomes 0
Περιεχόμενα. Πρόλογος 17 ΚΕΦΑΛΑΙΟ 1 23
 Περιεχόμενα Πρόλογος 17 Μέρος A ΚΕΦΑΛΑΙΟ 1 23 ΕΙΣΑΓΩΓΗ ΣΤΗ ΣΤΑΤΙΣΤΙΚΗ 23 1.1 Εισαγωγή 23 1.1.1 Περιγραφική Στατιστική (Descriptive Statistics) 24 1.1.2 Επαγωγική ή Αναλυτική Στατιστική (Inferential or
Περιεχόμενα Πρόλογος 17 Μέρος A ΚΕΦΑΛΑΙΟ 1 23 ΕΙΣΑΓΩΓΗ ΣΤΗ ΣΤΑΤΙΣΤΙΚΗ 23 1.1 Εισαγωγή 23 1.1.1 Περιγραφική Στατιστική (Descriptive Statistics) 24 1.1.2 Επαγωγική ή Αναλυτική Στατιστική (Inferential or
794 Appendix A:Tables
 Appendix A Tables A Table Contents Page A.1 Random numbers 794 A.2 Orthogonal polynomial trend contrast coefficients 800 A.3 Standard normal distribution 801 A.4 Student s t-distribution 802 A.5 Chi-squared
Appendix A Tables A Table Contents Page A.1 Random numbers 794 A.2 Orthogonal polynomial trend contrast coefficients 800 A.3 Standard normal distribution 801 A.4 Student s t-distribution 802 A.5 Chi-squared
Reflecting Brownian motion in two dimensions: Exact asymptotics for the stationary distribution
 Reflecting Brownian motion in two dimensions: Exact asymptotics for the stationary distribution Jim Dai Joint work with Masakiyo Miyazawa July 8, 211 211 INFORMS APS conference at Stockholm Jim Dai (Georgia
Reflecting Brownian motion in two dimensions: Exact asymptotics for the stationary distribution Jim Dai Joint work with Masakiyo Miyazawa July 8, 211 211 INFORMS APS conference at Stockholm Jim Dai (Georgia
Fourier Series. MATH 211, Calculus II. J. Robert Buchanan. Spring Department of Mathematics
 Fourier Series MATH 211, Calculus II J. Robert Buchanan Department of Mathematics Spring 2018 Introduction Not all functions can be represented by Taylor series. f (k) (c) A Taylor series f (x) = (x c)
Fourier Series MATH 211, Calculus II J. Robert Buchanan Department of Mathematics Spring 2018 Introduction Not all functions can be represented by Taylor series. f (k) (c) A Taylor series f (x) = (x c)
ω ω ω ω ω ω+2 ω ω+2 + ω ω ω ω+2 + ω ω+1 ω ω+2 2 ω ω ω ω ω ω ω ω+1 ω ω2 ω ω2 + ω ω ω2 + ω ω ω ω2 + ω ω+1 ω ω2 + ω ω+1 + ω ω ω ω2 + ω
 0 1 2 3 4 5 6 ω ω + 1 ω + 2 ω + 3 ω + 4 ω2 ω2 + 1 ω2 + 2 ω2 + 3 ω3 ω3 + 1 ω3 + 2 ω4 ω4 + 1 ω5 ω 2 ω 2 + 1 ω 2 + 2 ω 2 + ω ω 2 + ω + 1 ω 2 + ω2 ω 2 2 ω 2 2 + 1 ω 2 2 + ω ω 2 3 ω 3 ω 3 + 1 ω 3 + ω ω 3 +
0 1 2 3 4 5 6 ω ω + 1 ω + 2 ω + 3 ω + 4 ω2 ω2 + 1 ω2 + 2 ω2 + 3 ω3 ω3 + 1 ω3 + 2 ω4 ω4 + 1 ω5 ω 2 ω 2 + 1 ω 2 + 2 ω 2 + ω ω 2 + ω + 1 ω 2 + ω2 ω 2 2 ω 2 2 + 1 ω 2 2 + ω ω 2 3 ω 3 ω 3 + 1 ω 3 + ω ω 3 +
ΣΥΣΤΗΜΑΤΑ ΑΝΑΜΟΝΗΣ Queuing Systems Επισκόπηση Γνώσεων Πιθανοτήτων (2/2) Διαδικασία Γεννήσεων Θανάτων Η Ουρά Μ/Μ/1
 ΣΥΣΤΗΜΑΤΑ ΑΝΑΜΟΝΗΣ Queuing Systems Επισκόπηση Γνώσεων Πιθανοτήτων (2/2) Διαδικασία Γεννήσεων Θανάτων Η Ουρά Μ/Μ/1 Βασίλης Μάγκλαρης maglaris@netmode.ntua.gr 15/3/2017 Η ΔΙΑΔΙΚΑΣΙΑ ΚΑΤΑΜΕΤΡΗΣΗΣ ΓΕΓΟΝΟΤΩΝ
ΣΥΣΤΗΜΑΤΑ ΑΝΑΜΟΝΗΣ Queuing Systems Επισκόπηση Γνώσεων Πιθανοτήτων (2/2) Διαδικασία Γεννήσεων Θανάτων Η Ουρά Μ/Μ/1 Βασίλης Μάγκλαρης maglaris@netmode.ntua.gr 15/3/2017 Η ΔΙΑΔΙΚΑΣΙΑ ΚΑΤΑΜΕΤΡΗΣΗΣ ΓΕΓΟΝΟΤΩΝ
Homework 3 Solutions
 Homework 3 Solutions Igor Yanovsky (Math 151A TA) Problem 1: Compute the absolute error and relative error in approximations of p by p. (Use calculator!) a) p π, p 22/7; b) p π, p 3.141. Solution: For
Homework 3 Solutions Igor Yanovsky (Math 151A TA) Problem 1: Compute the absolute error and relative error in approximations of p by p. (Use calculator!) a) p π, p 22/7; b) p π, p 3.141. Solution: For
ESTIMATION OF SYSTEM RELIABILITY IN A TWO COMPONENT STRESS-STRENGTH MODELS DAVID D. HANAGAL
 ESTIMATION OF SYSTEM RELIABILITY IN A TWO COMPONENT STRESS-STRENGTH MODELS DAVID D. HANAGAL Department of Statistics, University of Poona, Pune-411007, India. Abstract In this paper, we estimate the reliability
ESTIMATION OF SYSTEM RELIABILITY IN A TWO COMPONENT STRESS-STRENGTH MODELS DAVID D. HANAGAL Department of Statistics, University of Poona, Pune-411007, India. Abstract In this paper, we estimate the reliability
Module 5. February 14, h 0min
 Module 5 Stationary Time Series Models Part 2 AR and ARMA Models and Their Properties Class notes for Statistics 451: Applied Time Series Iowa State University Copyright 2015 W. Q. Meeker. February 14,
Module 5 Stationary Time Series Models Part 2 AR and ARMA Models and Their Properties Class notes for Statistics 451: Applied Time Series Iowa State University Copyright 2015 W. Q. Meeker. February 14,
PART ONE. Solutions to Exercises
 PART ONE Soutions to Exercises Chapter Review of Probabiity Soutions to Exercises. (a) Probabiity distribution function for Outcome = 0 = = (number of heads) probabiity 0.5 0.50 0.5 Cumuative probabiity
PART ONE Soutions to Exercises Chapter Review of Probabiity Soutions to Exercises. (a) Probabiity distribution function for Outcome = 0 = = (number of heads) probabiity 0.5 0.50 0.5 Cumuative probabiity
SOLUTIONS TO MATH38181 EXTREME VALUES AND FINANCIAL RISK EXAM
 SOLUTIONS TO MATH38181 EXTREME VALUES AND FINANCIAL RISK EXAM Solutions to Question 1 a) The cumulative distribution function of T conditional on N n is Pr T t N n) Pr max X 1,..., X N ) t N n) Pr max
SOLUTIONS TO MATH38181 EXTREME VALUES AND FINANCIAL RISK EXAM Solutions to Question 1 a) The cumulative distribution function of T conditional on N n is Pr T t N n) Pr max X 1,..., X N ) t N n) Pr max
ΔΗΜΗΤΡΙΟΣ Λ. ΑΝΤΖΟΥΛΑΚΟΣ ΑΝΑΠΛΗΡΩΤΗΣ ΚΑΘΗΓΗΤΗΣ ΠΑΝΕΠΙΣΤΗΜΙΟΥ ΠΕΙΡΑΙΩΣ ΤΜΗΜΑ ΣΤΑΤΙΣΤΙΚΗΣ ΚΑΙ ΑΣΦΑΛΙΣΤΙΚΗΣ ΕΠΙΣΤΗΜΗΣ ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ
 ΔΗΜΗΤΡΙΟΣ Λ. ΑΝΤΖΟΥΛΑΚΟΣ ΑΝΑΠΛΗΡΩΤΗΣ ΚΑΘΗΓΗΤΗΣ ΠΑΝΕΠΙΣΤΗΜΙΟΥ ΠΕΙΡΑΙΩΣ ΤΜΗΜΑ ΣΤΑΤΙΣΤΙΚΗΣ ΚΑΙ ΑΣΦΑΛΙΣΤΙΚΗΣ ΕΠΙΣΤΗΜΗΣ ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ ΣΠΟΥΔΕΣ, ΣΤΑΔΙΟΔΡΟΜΙΑ ΕΡΕΥΝΗΤΙΚΟ ΕΡΓΟ ΠΕΙΡΑΙΑΣ 2014 1. Προσωπικά στοιχεία
ΔΗΜΗΤΡΙΟΣ Λ. ΑΝΤΖΟΥΛΑΚΟΣ ΑΝΑΠΛΗΡΩΤΗΣ ΚΑΘΗΓΗΤΗΣ ΠΑΝΕΠΙΣΤΗΜΙΟΥ ΠΕΙΡΑΙΩΣ ΤΜΗΜΑ ΣΤΑΤΙΣΤΙΚΗΣ ΚΑΙ ΑΣΦΑΛΙΣΤΙΚΗΣ ΕΠΙΣΤΗΜΗΣ ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ ΣΠΟΥΔΕΣ, ΣΤΑΔΙΟΔΡΟΜΙΑ ΕΡΕΥΝΗΤΙΚΟ ΕΡΓΟ ΠΕΙΡΑΙΑΣ 2014 1. Προσωπικά στοιχεία
PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI
 155 Lampiran 6 Yayan Sumaryana, 2014 PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI Universitas Pendidikan Indonesia
155 Lampiran 6 Yayan Sumaryana, 2014 PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI Universitas Pendidikan Indonesia
Exponential Families
 Exponential Families Robert L. Wolpert Department of Statistical Science Duke University, Durham, NC, USA Surprisingly many of the distributions we use in statistics for random variables taking value in
Exponential Families Robert L. Wolpert Department of Statistical Science Duke University, Durham, NC, USA Surprisingly many of the distributions we use in statistics for random variables taking value in
Μάθημα Τεχνοοικονομική ανάλυση δικτύων
 Μάθημα Τεχνοοικονομική ανάλυση δικτύων Ανάλυση Ευαισθησίας και Ανάλυση Κινδύνων Δρ. Δημήτρης Κατσιάνης Nils Kristian Elnergaard Εθνικό και Καποδιστριακό Πανεπιστήμιο Αθηνών Τμήμα Πληροφορικής και Τηλεπικοινωνιών
Μάθημα Τεχνοοικονομική ανάλυση δικτύων Ανάλυση Ευαισθησίας και Ανάλυση Κινδύνων Δρ. Δημήτρης Κατσιάνης Nils Kristian Elnergaard Εθνικό και Καποδιστριακό Πανεπιστήμιο Αθηνών Τμήμα Πληροφορικής και Τηλεπικοινωνιών
Stationary Stochastic Processes Table of Formulas, 2016
 Stationary Stochastic Processes, 06 Stationary Stochastic Processes Table of Formulas, 06 Basics of probability theory The following is valid for probabilities: P(Ω), where Ω is all possible outcomes 0
Stationary Stochastic Processes, 06 Stationary Stochastic Processes Table of Formulas, 06 Basics of probability theory The following is valid for probabilities: P(Ω), where Ω is all possible outcomes 0
= λ 1 1 e. = λ 1 =12. has the properties e 1. e 3,V(Y
 Stat 50 Homework Solutions Spring 005. (a λ λ λ 44 (b trace( λ + λ + λ 0 (c V (e x e e λ e e λ e (λ e by definition, the eigenvector e has the properties e λ e and e e. (d λ e e + λ e e + λ e e 8 6 4 4
Stat 50 Homework Solutions Spring 005. (a λ λ λ 44 (b trace( λ + λ + λ 0 (c V (e x e e λ e e λ e (λ e by definition, the eigenvector e has the properties e λ e and e e. (d λ e e + λ e e + λ e e 8 6 4 4
Additional Results for the Pareto/NBD Model
 Additional Results for the Pareto/NBD Model Peter S. Fader www.petefader.com Bruce G. S. Hardie www.brucehardie.com January 24 Abstract This note derives expressions for i) the raw moments of the posterior
Additional Results for the Pareto/NBD Model Peter S. Fader www.petefader.com Bruce G. S. Hardie www.brucehardie.com January 24 Abstract This note derives expressions for i) the raw moments of the posterior
Chapter 1 Introduction to Observational Studies Part 2 Cross-Sectional Selection Bias Adjustment
 Contents Preface ix Part 1 Introduction Chapter 1 Introduction to Observational Studies... 3 1.1 Observational vs. Experimental Studies... 3 1.2 Issues in Observational Studies... 5 1.3 Study Design...
Contents Preface ix Part 1 Introduction Chapter 1 Introduction to Observational Studies... 3 1.1 Observational vs. Experimental Studies... 3 1.2 Issues in Observational Studies... 5 1.3 Study Design...
TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics
 TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics Exploring Data: Distributions Look for overall pattern (shape, center, spread) and deviations (outliers). Mean (use a calculator): x = x 1 + x
TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics Exploring Data: Distributions Look for overall pattern (shape, center, spread) and deviations (outliers). Mean (use a calculator): x = x 1 + x
ST5224: Advanced Statistical Theory II
 ST5224: Advanced Statistical Theory II 2014/2015: Semester II Tutorial 7 1. Let X be a sample from a population P and consider testing hypotheses H 0 : P = P 0 versus H 1 : P = P 1, where P j is a known
ST5224: Advanced Statistical Theory II 2014/2015: Semester II Tutorial 7 1. Let X be a sample from a population P and consider testing hypotheses H 0 : P = P 0 versus H 1 : P = P 1, where P j is a known
5. Choice under Uncertainty
 5. Choice under Uncertainty Daisuke Oyama Microeconomics I May 23, 2018 Formulations von Neumann-Morgenstern (1944/1947) X: Set of prizes Π: Set of probability distributions on X : Preference relation
5. Choice under Uncertainty Daisuke Oyama Microeconomics I May 23, 2018 Formulations von Neumann-Morgenstern (1944/1947) X: Set of prizes Π: Set of probability distributions on X : Preference relation
ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ. Λέκτορας στο Τμήμα Οργάνωσης και Διοίκησης Επιχειρήσεων, Πανεπιστήμιο Πειραιώς, Ιανουάριος 2012-Μάρτιος 2014.
 ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ 1. Γενικά στοιχεία Όνομα Επίθετο Θέση E-mail Πέτρος Μαραβελάκης Επίκουρος καθηγητής στο Πανεπιστήμιο Πειραιώς, Τμήμα Οργάνωσης και Διοίκησης Επιχειρήσεων με αντικείμενο «Εφαρμογές Στατιστικής
ΒΙΟΓΡΑΦΙΚΟ ΣΗΜΕΙΩΜΑ 1. Γενικά στοιχεία Όνομα Επίθετο Θέση E-mail Πέτρος Μαραβελάκης Επίκουρος καθηγητής στο Πανεπιστήμιο Πειραιώς, Τμήμα Οργάνωσης και Διοίκησης Επιχειρήσεων με αντικείμενο «Εφαρμογές Στατιστικής
3.4 SUM AND DIFFERENCE FORMULAS. NOTE: cos(α+β) cos α + cos β cos(α-β) cos α -cos β
 3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
ECE598: Information-theoretic methods in high-dimensional statistics Spring 2016
 ECE598: Information-theoretic methods in high-dimensional statistics Spring 06 Lecture 7: Information bound Lecturer: Yihong Wu Scribe: Shiyu Liang, Feb 6, 06 [Ed. Mar 9] Recall the Chi-squared divergence
ECE598: Information-theoretic methods in high-dimensional statistics Spring 06 Lecture 7: Information bound Lecturer: Yihong Wu Scribe: Shiyu Liang, Feb 6, 06 [Ed. Mar 9] Recall the Chi-squared divergence
Important Probability Distributions
 D Important Probability Distributions Development of stochastic models is facilitated by identifying a few probability distributions that seem to correspond to a variety of data-generating processes, and
D Important Probability Distributions Development of stochastic models is facilitated by identifying a few probability distributions that seem to correspond to a variety of data-generating processes, and
Machine Learning. Lecture 5: Gaussian Discriminant Analysis, Naive Bayes and EM Algorithm. Feng Li.
 Machine Learning Lecture 5: Gaussian Discriminant Analysis, Naive Bayes and EM Algorithm Feng Li fli@sdu.edu.cn https://funglee.github.io School of Computer Science and Technology Shandong University Fall
Machine Learning Lecture 5: Gaussian Discriminant Analysis, Naive Bayes and EM Algorithm Feng Li fli@sdu.edu.cn https://funglee.github.io School of Computer Science and Technology Shandong University Fall
Contents Introduction to Filter Concepts All-Pole Approximations
 Contents 1 Introduction to Filter Concepts... 1 1.1 Gain and Attenuation Functions..... 1 1.2 Ideal Transmission... 4 1.2.1 Ideal Filters... 5 1.3 Real Electronic Filters... 6 1.3.1 Realizable Lowpass
Contents 1 Introduction to Filter Concepts... 1 1.1 Gain and Attenuation Functions..... 1 1.2 Ideal Transmission... 4 1.2.1 Ideal Filters... 5 1.3 Real Electronic Filters... 6 1.3.1 Realizable Lowpass
Chapter 6: Systems of Linear Differential. be continuous functions on the interval
 Chapter 6: Systems of Linear Differential Equations Let a (t), a 2 (t),..., a nn (t), b (t), b 2 (t),..., b n (t) be continuous functions on the interval I. The system of n first-order differential equations
Chapter 6: Systems of Linear Differential Equations Let a (t), a 2 (t),..., a nn (t), b (t), b 2 (t),..., b n (t) be continuous functions on the interval I. The system of n first-order differential equations
Jesse Maassen and Mark Lundstrom Purdue University November 25, 2013
 Notes on Average Scattering imes and Hall Factors Jesse Maassen and Mar Lundstrom Purdue University November 5, 13 I. Introduction 1 II. Solution of the BE 1 III. Exercises: Woring out average scattering
Notes on Average Scattering imes and Hall Factors Jesse Maassen and Mar Lundstrom Purdue University November 5, 13 I. Introduction 1 II. Solution of the BE 1 III. Exercises: Woring out average scattering
Web-based supplementary materials for Bayesian Quantile Regression for Ordinal Longitudinal Data
 Web-based supplementary materials for Bayesian Quantile Regression for Ordinal Longitudinal Data Rahim Alhamzawi, Haithem Taha Mohammad Ali Department of Statistics, College of Administration and Economics,
Web-based supplementary materials for Bayesian Quantile Regression for Ordinal Longitudinal Data Rahim Alhamzawi, Haithem Taha Mohammad Ali Department of Statistics, College of Administration and Economics,
The k-bessel Function of the First Kind
 International Mathematical Forum, Vol. 7, 01, no. 38, 1859-186 The k-bessel Function of the First Kin Luis Guillermo Romero, Gustavo Abel Dorrego an Ruben Alejanro Cerutti Faculty of Exact Sciences National
International Mathematical Forum, Vol. 7, 01, no. 38, 1859-186 The k-bessel Function of the First Kin Luis Guillermo Romero, Gustavo Abel Dorrego an Ruben Alejanro Cerutti Faculty of Exact Sciences National
Srednicki Chapter 55
 Srednicki Chapter 55 QFT Problems & Solutions A. George August 3, 03 Srednicki 55.. Use equations 55.3-55.0 and A i, A j ] = Π i, Π j ] = 0 (at equal times) to verify equations 55.-55.3. This is our third
Srednicki Chapter 55 QFT Problems & Solutions A. George August 3, 03 Srednicki 55.. Use equations 55.3-55.0 and A i, A j ] = Π i, Π j ] = 0 (at equal times) to verify equations 55.-55.3. This is our third
Bayesian modeling of inseparable space-time variation in disease risk
 Bayesian modeling of inseparable space-time variation in disease risk Leonhard Knorr-Held Laina Mercer Department of Statistics UW May, 013 Motivation Ohio Lung Cancer Example Lung Cancer Mortality Rates
Bayesian modeling of inseparable space-time variation in disease risk Leonhard Knorr-Held Laina Mercer Department of Statistics UW May, 013 Motivation Ohio Lung Cancer Example Lung Cancer Mortality Rates
Markov chains model reduction
 Markov chains model reduction C. Landim Seminar on Stochastic Processes 216 Department of Mathematics University of Maryland, College Park, MD C. Landim Markov chains model reduction March 17, 216 1 /
Markov chains model reduction C. Landim Seminar on Stochastic Processes 216 Department of Mathematics University of Maryland, College Park, MD C. Landim Markov chains model reduction March 17, 216 1 /
Elements of Information Theory
 Elements of Information Theory Model of Digital Communications System A Logarithmic Measure for Information Mutual Information Units of Information Self-Information News... Example Information Measure
Elements of Information Theory Model of Digital Communications System A Logarithmic Measure for Information Mutual Information Units of Information Self-Information News... Example Information Measure
10/3/ revolution = 360 = 2 π radians = = x. 2π = x = 360 = : Measures of Angles and Rotations
 //.: Measures of Angles and Rotations I. Vocabulary A A. Angle the union of two rays with a common endpoint B. BA and BC C. B is the vertex. B C D. You can think of BA as the rotation of (clockwise) with
//.: Measures of Angles and Rotations I. Vocabulary A A. Angle the union of two rays with a common endpoint B. BA and BC C. B is the vertex. B C D. You can think of BA as the rotation of (clockwise) with
Βιογραφικό Σημείωμα. Διεύθυνση επικοινωνίας: Τμήμα Μαθηματικών, Πανεπιστήμιο Πατρών
 Βιογραφικό Σημείωμα Προσωπικά στοιχεία Όνομα: Σταύρος Επώνυμο: Κουρούκλης Έτος γέννησης: 1952 Τόπος γέννησης: Ληξούρι Κεφαλλονιάς Στρατιωτική θητεία: Φεβρουάριος 2002 Οκτώβριος 2003 Οικογενειακή κατάσταση:
Βιογραφικό Σημείωμα Προσωπικά στοιχεία Όνομα: Σταύρος Επώνυμο: Κουρούκλης Έτος γέννησης: 1952 Τόπος γέννησης: Ληξούρι Κεφαλλονιάς Στρατιωτική θητεία: Φεβρουάριος 2002 Οκτώβριος 2003 Οικογενειακή κατάσταση:
Contents. Preface. 4 Support Vector Machines Linearclassification SVMs separablecase... 64
 Contents Preface xi 1 Introduction 1 1.1 Applicationsandproblems... 1 1.2 Definitionsandterminology... 3 1.3 Cross-validation... 5 1.4 Learningscenarios... 7 1.5 Outline... 8 2 The PAC Learning Framework
Contents Preface xi 1 Introduction 1 1.1 Applicationsandproblems... 1 1.2 Definitionsandterminology... 3 1.3 Cross-validation... 5 1.4 Learningscenarios... 7 1.5 Outline... 8 2 The PAC Learning Framework
STAT200C: Hypothesis Testing
 STAT200C: Hypothesis Testing Zhaoxia Yu Spring 2017 Some Definitions A hypothesis is a statement about a population parameter. The two complementary hypotheses in a hypothesis testing are the null hypothesis
STAT200C: Hypothesis Testing Zhaoxia Yu Spring 2017 Some Definitions A hypothesis is a statement about a population parameter. The two complementary hypotheses in a hypothesis testing are the null hypothesis
Notations. Primary definition. Specific values. General characteristics. Traditional name. Traditional notation. Mathematica StandardForm notation
 KelvinKei Notations Traditional name Kelvin function of the second kind Traditional notation kei Mathematica StandardForm notation KelvinKei Primary definition 03.5.0.000.0 kei kei 0 Specific values Values
KelvinKei Notations Traditional name Kelvin function of the second kind Traditional notation kei Mathematica StandardForm notation KelvinKei Primary definition 03.5.0.000.0 kei kei 0 Specific values Values
Confidence Intervals for Negative Binomial Random Variables of High Dispersion
 Confidence Intervals for Negative Binomial Random Variables of High Dispersion David Shilane, Steven N. Evans, Alan E. Hubbard September 25, 2009 Abstract This paper considers the problem of constructing
Confidence Intervals for Negative Binomial Random Variables of High Dispersion David Shilane, Steven N. Evans, Alan E. Hubbard September 25, 2009 Abstract This paper considers the problem of constructing
Discrete scan statistics with windows of arbitrary shape
 Discrete scan statistics with windows of arbitrary shape Alexandru Am rioarei National Institute of Research and Development for Biological Sciences Bucharest, Romania MΘDAL TEAM - INRIA Lille Nord Europe
Discrete scan statistics with windows of arbitrary shape Alexandru Am rioarei National Institute of Research and Development for Biological Sciences Bucharest, Romania MΘDAL TEAM - INRIA Lille Nord Europe
Chapter 6: Systems of Linear Differential. be continuous functions on the interval
 Chapter 6: Systems of Linear Differential Equations Let a (t), a 2 (t),..., a nn (t), b (t), b 2 (t),..., b n (t) be continuous functions on the interval I. The system of n first-order differential equations
Chapter 6: Systems of Linear Differential Equations Let a (t), a 2 (t),..., a nn (t), b (t), b 2 (t),..., b n (t) be continuous functions on the interval I. The system of n first-order differential equations
Computable error bounds for asymptotic expansions formulas of distributions related to gamma functions
 Computable error bounds for asymptotic expansions formulas of distributions related to gamma functions Hirofumi Wakaki (Math. of Department, Hiroshima Univ.) 20.7. Hiroshima Statistical Group Meeting at
Computable error bounds for asymptotic expansions formulas of distributions related to gamma functions Hirofumi Wakaki (Math. of Department, Hiroshima Univ.) 20.7. Hiroshima Statistical Group Meeting at
ΚΑΤΑΝΟΜΈΣ. 8.1 Εισαγωγή. 8.2 Κατανομές Συχνοτήτων (Frequency Distributions) ΚΕΦΑΛΑΙΟ
 ΚΑΤΑΝΟΜΈΣ ΚΕΦΑΛΑΙΟ 8 81 Εισαγωγή Οι κατανομές διακρίνονται σε κατανομές συχνοτήτων, κατανομές πιθανοτήτων και σε δειγματοληπτικές κατανομές Στη συνέχεια θα γίνει αναλυτική περιγραφή αυτών 82 Κατανομές
ΚΑΤΑΝΟΜΈΣ ΚΕΦΑΛΑΙΟ 8 81 Εισαγωγή Οι κατανομές διακρίνονται σε κατανομές συχνοτήτων, κατανομές πιθανοτήτων και σε δειγματοληπτικές κατανομές Στη συνέχεια θα γίνει αναλυτική περιγραφή αυτών 82 Κατανομές
SOME PROPERTIES OF FUZZY REAL NUMBERS
 Sahand Communications in Mathematical Analysis (SCMA) Vol. 3 No. 1 (2016), 21-27 http://scma.maragheh.ac.ir SOME PROPERTIES OF FUZZY REAL NUMBERS BAYAZ DARABY 1 AND JAVAD JAFARI 2 Abstract. In the mathematical
Sahand Communications in Mathematical Analysis (SCMA) Vol. 3 No. 1 (2016), 21-27 http://scma.maragheh.ac.ir SOME PROPERTIES OF FUZZY REAL NUMBERS BAYAZ DARABY 1 AND JAVAD JAFARI 2 Abstract. In the mathematical
Part III - Pricing A Down-And-Out Call Option
 Part III - Pricing A Down-And-Out Call Option Gary Schurman MBE, CFA March 202 In Part I we examined the reflection principle and a scaled random walk in discrete time and then extended the reflection
Part III - Pricing A Down-And-Out Call Option Gary Schurman MBE, CFA March 202 In Part I we examined the reflection principle and a scaled random walk in discrete time and then extended the reflection
Other Test Constructions: Likelihood Ratio & Bayes Tests
 Other Test Constructions: Likelihood Ratio & Bayes Tests Side-Note: So far we have seen a few approaches for creating tests such as Neyman-Pearson Lemma ( most powerful tests of H 0 : θ = θ 0 vs H 1 :
Other Test Constructions: Likelihood Ratio & Bayes Tests Side-Note: So far we have seen a few approaches for creating tests such as Neyman-Pearson Lemma ( most powerful tests of H 0 : θ = θ 0 vs H 1 :
Εισαγωγή σε μεθόδους Monte Carlo Ενότητα 3: Δειγματοληπτικές μέθοδοι
 ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΤΙΑ ΠΑΝΕΠΙΣΤΗΜΙΟ ΚΡΗΤΗΣ Εισαγωγή σε μεθόδους Monte Carlo Ενότητα 3: Δειγματοληπτικές μέθοδοι Βαγγέλης Χαρμανδάρης Τμήμα Μαθηματικών και Εφαρμοσμένων Μαθηματικών Transformation Methods:
ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΤΙΑ ΠΑΝΕΠΙΣΤΗΜΙΟ ΚΡΗΤΗΣ Εισαγωγή σε μεθόδους Monte Carlo Ενότητα 3: Δειγματοληπτικές μέθοδοι Βαγγέλης Χαρμανδάρης Τμήμα Μαθηματικών και Εφαρμοσμένων Μαθηματικών Transformation Methods:
The Negative Neumann Eigenvalues of Second Order Differential Equation with Two Turning Points
 Applied Mathematical Sciences, Vol. 3, 009, no., 6-66 The Negative Neumann Eigenvalues of Second Order Differential Equation with Two Turning Points A. Neamaty and E. A. Sazgar Department of Mathematics,
Applied Mathematical Sciences, Vol. 3, 009, no., 6-66 The Negative Neumann Eigenvalues of Second Order Differential Equation with Two Turning Points A. Neamaty and E. A. Sazgar Department of Mathematics,
ΣΤΟΙΧΕΙΑ ΣΤΑ ΕΛΛΗΝΙΚΑ ΓΙΑ ΤΟΥΣ ΔΙΔΑΣΚΟΝΤΕΣ ΤΟΥ ΠΜΣ
 ΣΤΟΙΧΕΙΑ ΣΤΑ ΕΛΛΗΝΙΚΑ ΓΙΑ ΤΟΥΣ ΔΙΔΑΣΚΟΝΤΕΣ ΤΟΥ ΠΜΣ Ονοματεπώνυμο : Μάρκος Κούτρας Τίτλος : Καθηγητής Τμήμα : Στατιστικής & Ασφαλιστικής Επιστήμης Ίδρυμα : Πανεπιστήμιο Πειραιώς Διεύθυνση Γραφείο: Καραολή
ΣΤΟΙΧΕΙΑ ΣΤΑ ΕΛΛΗΝΙΚΑ ΓΙΑ ΤΟΥΣ ΔΙΔΑΣΚΟΝΤΕΣ ΤΟΥ ΠΜΣ Ονοματεπώνυμο : Μάρκος Κούτρας Τίτλος : Καθηγητής Τμήμα : Στατιστικής & Ασφαλιστικής Επιστήμης Ίδρυμα : Πανεπιστήμιο Πειραιώς Διεύθυνση Γραφείο: Καραολή
NOB= Dickey=Fuller Engle-Granger., P. ( ). NVAR=Engle-Granger/Dickey-Fuller. 1( ), 6. CONSTANT/NOCONST (C) Dickey-Fuller. NOCONST NVAR=1. TREND/NOTREN
 CDF(BIVNORM or CHISQ or DICKEYF or F or NORMAL or T or WTDCHI, DF=CHISQ T, DF1=F, DF2=F, NLAGS= Dickey-Fuller, NOB=, NVAR=, RHO=BIVNORM, EIGVAL=WTDCHI, LOWTAIL or UPTAIL or TWOTAIL, CONSTANT, TREND, TSQ,
CDF(BIVNORM or CHISQ or DICKEYF or F or NORMAL or T or WTDCHI, DF=CHISQ T, DF1=F, DF2=F, NLAGS= Dickey-Fuller, NOB=, NVAR=, RHO=BIVNORM, EIGVAL=WTDCHI, LOWTAIL or UPTAIL or TWOTAIL, CONSTANT, TREND, TSQ,
