Key Formulas From Larson/Farber Elementary Statistics: Picturing the World, Second Edition 2002 Prentice Hall
|
|
|
- Πρίαμ Λαμέρας
- 8 χρόνια πριν
- Προβολές:
Transcript
1 64_INS.qxd /6/0 :56 AM Page Key Formulas From Larson/Farber Elemenary Saisics: Picuring he World, Second Ediion 00 Prenice Hall CHAPTER Class Widh = round up o nex convenien number Maximum daa enry - Minimum daa enry of Number of classes Lower class limi + Upper class limi Midpoin = Class frequency Relaive Frequency = = f Sample sie n Populaion Mean: Sample Mean: Weighed Mean: Mean for Grouped Daa: Range Populaion Variance: Populaion Sandard Deviaion: Sample Variance: Sample Sandard Deviaion: Sample Sandard Deviaion for Grouped Daa: s = C gx - x f n - m = gx N x = gx n x = gx # w gw s = s = C gx - m N s = s = x = gx # f n = Maximum enry - Minimum enry gx - m gx - x n - Empirical Rule (or Rule) For daa wih a (symmeric) bell-shaped disribuion. Abou 68% of he daa lies beween m - s and m + s.. Abou 95% of he daa lies beween m - s and m + s. 3. Abou 99.7% of he daa lies beween m - 3s and m + 3s. N s = s = C gx - x n - Chebychev s Theorem The porion of any daa se lying wihin k sandard deviaions k 7 of he mean is a leas - k. Sandard Score: CHAPTER 3 Classical (or Theoreical) Probabiliy: PE = Empirical (or Saisical) Probabiliy: Frequency of even E PE = = f Toal frequency n Probabiliy of a Complemen: Probabiliy of occurrence of boh evens A and B: PA and B = PA # PBƒA PA and B = PA # PB if A and B are independen Probabiliy of occurrence of eiher A or B or boh: PA or B = PA + PB - PA and B PA or B = PA + PB if evens are muually exclusive. Permuaions of n objecs aken r a a ime: np r = Disinguishable Permuaions: n alike, n alike, Á, alike: n k n! n! # n! # n! Á n k! where n + n + n 3 + Á + n k = n. Combinaion of n objecs aken r a a ime: nc r = Number of oucomes in E Toal number of oucomes in sample space n! where r n. n - r!, n! n - r!r! = value - mean sandard deviaion = x - m s PE = - PE
2 64_INS.qxd /6/0 :56 AM Page Key Formulas From Larson/Farber Elemenary Saisics: Picuring he World, Second Ediion 00 Prenice Hall CHAPTER 4 Mean of a Discree Random Variable: Variance of a Discree Random Variable: s = gx - m Px Sandard Deviaion: Expeced Value: Binomial Probabiliy of x successes in n rials: Px = n C x p x q n - x = Populaion Parameers of a Binomial Disribuion: Mean: m = np s = s Ex = m = gxpx n! n - x!x! px q n - x Variance: s = npq m = gxpx CHAPTER 6 c-confidence Inerval for m: x - E 6 m 6 x + E s where E = c if s is known and he populaion is n s normal or n Ú 30, and E = c if he populaion is n normal, s is unknown, and n Minimum Sample Sie o Esimae : n = a cs m E b Poin Esimae for p, he populaion proporion of successes: pn = x n c-confidence Inerval for Populaion Proporion p (when np Ú 5 and nq Ú 5: pn - E 6 p 6 pn + E, where Sandard Deviaion: s = npq Geomeric Disribuion: The probabiliy ha he firs success will occur on rial number x is Px = pq x -, where q = - p. pnqn E = c B n. Minimum Sample Sie o Esimae p: n = pnqn a c E b Poisson Disribuion: The probabiliy of exacly x occurrences in an inerval is Px = mx e -m, where e L.788. x! CHAPTER 5 Sandard Score, or -Score: = value - mean sandard deviaion = x - m s c-confidence Inerval for Populaion Variance s : n - s x R n - 6 s s 6 x L c-confidence Inerval for Populaion Sandard Deviaion s: n - s n - s 6 s 6 C x R C x L Transforming a -Score o an x-value: x = m + s m x = m Cenral Limi Theorem (Mean of he Sample Means) s x = s n Cenral Limi Theorem (Sandard Error) = x - m x s x = x - m s> n Cenral Limi Theorem
3 64_INS.qxd /6/0 :56 AM Page 3 Key Formulas From Larson/Farber Elemenary Saisics: Picuring he World, Second Ediion 00 Prenice Hall CHAPTER 7 - Tes for a Mean m : = x - m for s known wih a s> n, normal populaion, or for n Ú Tes for a Mean m : = x - m for s unknown, x s> n, normally disribued, and n Tes for a Proporion p (when np Ú 5 and nq Ú 5: = Chi-Square Tes for a Variance or Sandard Deviaion: CHAPTER 8 Two-Sample -Tes for he Difference Beween Means: (Independen samples; n and n Ú 30 or normally disribued populaions) where Two-Sample -Tes for he Difference Beween Means: (Independen samples from normally disribued populaions, or n 6 30) If populaion variances are equal, d.f. = n + n - and pn - p pq>n n - x s = s n = x - x - m - m s x - x If populaion variances are no equal, d.f. is he smaller of n - or n - and d.f. = n - = x - x - m - m s x - x, s x - x = C n - s + n - s n + n - s x - x = B s n + s n. -Tes for he Difference Beween Means: (Dependen samples) d.f. = n - s x - x # = B s n + s n +. B n n = d - m d s d > n, where and d.f. = n -. Two-Sample -Tes for he Difference Beween Proporions: Noe: n p, n q, n p, and n q mus be a leas 5. = pn - pn - p - p, where CHAPTER 9 Correlaion Coefficien: r = -Tes for he Correlaion Coefficien: = B p qa + b n n r - r B n - (d.f. = n - ) Equaion of a Regression Line: ngxy - gxgy where m = and ngx - gx b = y - mx = gy n d = gd n, ngxy - gxgy ngx - gx ngy - gy - m gx n Coefficien of Deerminaion: explained variaion r = oal variaion Sandard Error of Esimae: = gyn i - y gy i - y c-predicion Inerval for y is yn - E 6 y 6 yn + E, where E = c s e C + n + nx 0 - x ngx - gx. ngd - gd s d =, C nn - p = x + x n + n. yn = mx + b s e = C gy i - yn i n - d.f. = n -
4 64_INS.qxd /6/0 :56 AM Page 4 Key Formulas From Larson/Farber Elemenary Saisics: Picuring he World, Second Ediion 00 Prenice Hall CHAPTER 0 Chi-Square: Goodness-of-Fi Tes: Tes of Independence: d.f. N = n -, and d.f. D = n - One-Way Analysis of Variance Tes: F = MS B MS W, O - E x = g E where MS B = and MS W = SS W N - k = gn i - s i. N - k ( d.f. N = k -, d.f. D = N - k) d.f. = k - d.f. = no. of rows - no. of columns - Two-Sample F-Tes for Variances: s Ú s SS B k - = gn i ax i - gx N b k - F = s s CHAPTER Tes Saisic for Sign Tes: When n 7 5, x n =, where x is he smaller number of 0.5n + or - signs and n is he oal number of + and - signs. When n 5, he es saisic is he smaller number of + or - signs. Tes Saisic for Wilcoxon Rank Sum Tes: = R - m R, s R where R = sum of he ranks for he smaller sample, m R = n n + n +, n n. Tes Saisic for he Kruskal-Wallis Tes: Given hree or more independen samples, he es saisic for he Kruskal-Wallis es is H = n n n + n + s R =, B NN + a R + R + Á + R k b - 3N +. n n n k and d.f. = k - The Spearman Rank Correlaion Coefficien: r s = - 6gd nn -
5 64_INS.qxd /6/0 :56 AM Page 5 Table 4 Sandard Normal Disribuion Area Criical Values Level of Confidence c c c = 0 c c
6 64_INS.qxd /6/0 :56 AM Page 6 Table 4 Sandard Normal Disribuion (coninued) Area
7 64_INS.qxd /6/0 :56 AM Page 7 Table 5 -Disribuion c-confidence inerval Lef-ailed es Righ-ailed es Two-ailed es Level of confidence, c One ail, d.f. Two ails, q
8 64_INS.qxd /6/0 :56 AM Page 8 Table 6 Chi-Square Disribuion χ χ χ L χ R χ Righ ail Two ails Degrees of freedom
The Student s t and F Distributions Page 1
 The Suden s and F Disribuions Page The Fundamenal Transformaion formula for wo random variables: Consider wo random variables wih join probabiliy disribuion funcion f (, ) simulaneously ake on values in
The Suden s and F Disribuions Page The Fundamenal Transformaion formula for wo random variables: Consider wo random variables wih join probabiliy disribuion funcion f (, ) simulaneously ake on values in
APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 651 APPENDIX B. BIBLIOGRAPHY 677 APPENDIX C. ANSWERS TO SELECTED EXERCISES 679
 APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 1 Table I Summary of Common Probability Distributions 2 Table II Cumulative Standard Normal Distribution Table III Percentage Points, 2 of the Chi-Squared
APPENDICES APPENDIX A. STATISTICAL TABLES AND CHARTS 1 Table I Summary of Common Probability Distributions 2 Table II Cumulative Standard Normal Distribution Table III Percentage Points, 2 of the Chi-Squared
Statistics 104: Quantitative Methods for Economics Formula and Theorem Review
 Harvard College Statistics 104: Quantitative Methods for Economics Formula and Theorem Review Tommy MacWilliam, 13 tmacwilliam@college.harvard.edu March 10, 2011 Contents 1 Introduction to Data 5 1.1 Sample
Harvard College Statistics 104: Quantitative Methods for Economics Formula and Theorem Review Tommy MacWilliam, 13 tmacwilliam@college.harvard.edu March 10, 2011 Contents 1 Introduction to Data 5 1.1 Sample
FORMULAS FOR STATISTICS 1
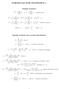 FORMULAS FOR STATISTICS 1 X = 1 n Sample statistics X i or x = 1 n x i (sample mean) S 2 = 1 n 1 s 2 = 1 n 1 (X i X) 2 = 1 n 1 (x i x) 2 = 1 n 1 Xi 2 n n 1 X 2 x 2 i n n 1 x 2 or (sample variance) E(X)
FORMULAS FOR STATISTICS 1 X = 1 n Sample statistics X i or x = 1 n x i (sample mean) S 2 = 1 n 1 s 2 = 1 n 1 (X i X) 2 = 1 n 1 (x i x) 2 = 1 n 1 Xi 2 n n 1 X 2 x 2 i n n 1 x 2 or (sample variance) E(X)
Biostatistics for Health Sciences Review Sheet
 Biostatistics for Health Sciences Review Sheet http://mathvault.ca June 1, 2017 Contents 1 Descriptive Statistics 2 1.1 Variables.............................................. 2 1.1.1 Qualitative........................................
Biostatistics for Health Sciences Review Sheet http://mathvault.ca June 1, 2017 Contents 1 Descriptive Statistics 2 1.1 Variables.............................................. 2 1.1.1 Qualitative........................................
Aquinas College. Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET
 Aquinas College Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET Pearson Edexcel Level 3 Advanced Subsidiary and Advanced GCE in Mathematics and Further Mathematics Mathematical
Aquinas College Edexcel Mathematical formulae and statistics tables DO NOT WRITE ON THIS BOOKLET Pearson Edexcel Level 3 Advanced Subsidiary and Advanced GCE in Mathematics and Further Mathematics Mathematical
TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics
 TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics Exploring Data: Distributions Look for overall pattern (shape, center, spread) and deviations (outliers). Mean (use a calculator): x = x 1 + x
TABLES AND FORMULAS FOR MOORE Basic Practice of Statistics Exploring Data: Distributions Look for overall pattern (shape, center, spread) and deviations (outliers). Mean (use a calculator): x = x 1 + x
ΣΤΑΤΙΣΤΙΚΗ ΕΠΙΧΕΙΡΗΣΕΩΝ ΕΙΔΙΚΑ ΘΕΜΑΤΑ. Κεφάλαιο 13. Συμπεράσματα για τη σύγκριση δύο πληθυσμών
 ΤΕΧΝΟΛΟΓΙΚΟ ΕΚΠΑΙΔΕΥΤΙΚΟ ΙΔΡΥΜΑ ΔΥΤΙΚΗΣ ΕΛΛΑΔΑΣ ΤΜΗΜΑ ΔΙΟΙΚΗΣΗΣ ΕΠΙΧΕΙΡΗΣΕΩΝ ΠΑΤΡΑΣ Εργαστήριο Λήψης Αποφάσεων & Επιχειρησιακού Προγραμματισμού Καθηγητής Ι. Μητρόπουλος ΣΤΑΤΙΣΤΙΚΗ ΕΠΙΧΕΙΡΗΣΕΩΝ ΕΙΔΙΚΑ ΘΕΜΑΤΑ
ΤΕΧΝΟΛΟΓΙΚΟ ΕΚΠΑΙΔΕΥΤΙΚΟ ΙΔΡΥΜΑ ΔΥΤΙΚΗΣ ΕΛΛΑΔΑΣ ΤΜΗΜΑ ΔΙΟΙΚΗΣΗΣ ΕΠΙΧΕΙΡΗΣΕΩΝ ΠΑΤΡΑΣ Εργαστήριο Λήψης Αποφάσεων & Επιχειρησιακού Προγραμματισμού Καθηγητής Ι. Μητρόπουλος ΣΤΑΤΙΣΤΙΚΗ ΕΠΙΧΕΙΡΗΣΕΩΝ ΕΙΔΙΚΑ ΘΕΜΑΤΑ
d dt S = (t)si d dt R = (t)i d dt I = (t)si (t)i
 d d S = ()SI d d I = ()SI ()I d d R = ()I d d S = ()SI μs + fi + hr d d I = + ()SI (μ + + f + ())I d d R = ()I (μ + h)r d d P(S,I,) = ()(S +1)(I 1)P(S +1, I 1, ) +()(I +1)P(S,I +1, ) (()SI + ()I)P(S,I,)
d d S = ()SI d d I = ()SI ()I d d R = ()I d d S = ()SI μs + fi + hr d d I = + ()SI (μ + + f + ())I d d R = ()I (μ + h)r d d P(S,I,) = ()(S +1)(I 1)P(S +1, I 1, ) +()(I +1)P(S,I +1, ) (()SI + ()I)P(S,I,)
5.4 The Poisson Distribution.
 The worst thing you can do about a situation is nothing. Sr. O Shea Jackson 5.4 The Poisson Distribution. Description of the Poisson Distribution Discrete probability distribution. The random variable
The worst thing you can do about a situation is nothing. Sr. O Shea Jackson 5.4 The Poisson Distribution. Description of the Poisson Distribution Discrete probability distribution. The random variable
Για να ελέγξουµε αν η κατανοµή µιας µεταβλητής είναι συµβατή µε την κανονική εφαρµόζουµε το test Kolmogorov-Smirnov.
 A. ΈΛΕΓΧΟΣ ΚΑΝΟΝΙΚΟΤΗΤΑΣ A 1. Έλεγχος κανονικότητας Kolmogorov-Smirnov. Για να ελέγξουµε αν η κατανοµή µιας µεταβλητής είναι συµβατή µε την κανονική εφαρµόζουµε το test Kolmogorov-Smirnov. Μηδενική υπόθεση:
A. ΈΛΕΓΧΟΣ ΚΑΝΟΝΙΚΟΤΗΤΑΣ A 1. Έλεγχος κανονικότητας Kolmogorov-Smirnov. Για να ελέγξουµε αν η κατανοµή µιας µεταβλητής είναι συµβατή µε την κανονική εφαρµόζουµε το test Kolmogorov-Smirnov. Μηδενική υπόθεση:
ΕΡΓΑΣΙΑ ΜΑΘΗΜΑΤΟΣ: ΘΕΩΡΙΑ ΒΕΛΤΙΣΤΟΥ ΕΛΕΓΧΟΥ ΦΙΛΤΡΟ KALMAN ΜΩΥΣΗΣ ΛΑΖΑΡΟΣ
 ΑΡΙΣΤΟΤΕΛΕΙΟ ΠΑΝΕΠΙΣΤΗΜΙΟ ΘΕΣΣΑΛΟΝΙΚΗΣ ΤΜΗΜΑ ΜΑΘΗΜΑΤΙΚΩΝ ΜΕΤΑΠΤΥΧΙΑΚΟ ΠΡΟΓΡΑΜΜΑ ΣΠΟΥΔΩΝ ΘΕΩΡΗΤΙΚΗ ΠΛΗΡΟΦΟΡΙΚΗ ΚΑΙ ΘΕΩΡΙΑ ΣΥΣΤΗΜΑΤΩΝ & ΕΛΕΓΧΟΥ ΕΡΓΑΣΙΑ ΜΑΘΗΜΑΤΟΣ: ΘΕΩΡΙΑ ΒΕΛΤΙΣΤΟΥ ΕΛΕΓΧΟΥ ΦΙΛΤΡΟ KALMAN ΜΩΥΣΗΣ
ΑΡΙΣΤΟΤΕΛΕΙΟ ΠΑΝΕΠΙΣΤΗΜΙΟ ΘΕΣΣΑΛΟΝΙΚΗΣ ΤΜΗΜΑ ΜΑΘΗΜΑΤΙΚΩΝ ΜΕΤΑΠΤΥΧΙΑΚΟ ΠΡΟΓΡΑΜΜΑ ΣΠΟΥΔΩΝ ΘΕΩΡΗΤΙΚΗ ΠΛΗΡΟΦΟΡΙΚΗ ΚΑΙ ΘΕΩΡΙΑ ΣΥΣΤΗΜΑΤΩΝ & ΕΛΕΓΧΟΥ ΕΡΓΑΣΙΑ ΜΑΘΗΜΑΤΟΣ: ΘΕΩΡΙΑ ΒΕΛΤΙΣΤΟΥ ΕΛΕΓΧΟΥ ΦΙΛΤΡΟ KALMAN ΜΩΥΣΗΣ
9.1 Introduction 9.2 Lags in the Error Term: Autocorrelation 9.3 Estimating an AR(1) Error Model 9.4 Testing for Autocorrelation 9.
 9.1 Inroducion 9.2 Lags in he Error Term: Auocorrelaion 9.3 Esimaing an AR(1) Error Model 9.4 Tesing for Auocorrelaion 9.5 An Inroducion o Forecasing: Auoregressive Models 9.6 Finie Disribued Lags 9.7
9.1 Inroducion 9.2 Lags in he Error Term: Auocorrelaion 9.3 Esimaing an AR(1) Error Model 9.4 Tesing for Auocorrelaion 9.5 An Inroducion o Forecasing: Auoregressive Models 9.6 Finie Disribued Lags 9.7
ο),,),--,ο< $ι ιι!η ι ηι ι ιι ιι t (t-test): ι ι η ι ι. $ι ι η ι ι ι 2 x s ι ι η η ιη ι η η SE x
 η &, ε ε 007!# # # ι, ι, η ιι ι ι ι ι η (.. ι, η ι η, ι & ι!ι η 50, ι ηιη 000 ι, ι, ',!,! )!η. (, ηι, ι ι ι ι "!η. #, ι "ι!η ι, ηι, ι ι ι η. ι, ι ι, ' ι ι ι η ι ι ι ι # ι ι ι ι ι 7. ο),,),--,ο< $ι ιι!η
η &, ε ε 007!# # # ι, ι, η ιι ι ι ι ι η (.. ι, η ι η, ι & ι!ι η 50, ι ηιη 000 ι, ι, ',!,! )!η. (, ηι, ι ι ι ι "!η. #, ι "ι!η ι, ηι, ι ι ι η. ι, ι ι, ' ι ι ι η ι ι ι ι # ι ι ι ι ι 7. ο),,),--,ο< $ι ιι!η
Solution Series 9. i=1 x i and i=1 x i.
 Lecturer: Prof. Dr. Mete SONER Coordinator: Yilin WANG Solution Series 9 Q1. Let α, β >, the p.d.f. of a beta distribution with parameters α and β is { Γ(α+β) Γ(α)Γ(β) f(x α, β) xα 1 (1 x) β 1 for < x
Lecturer: Prof. Dr. Mete SONER Coordinator: Yilin WANG Solution Series 9 Q1. Let α, β >, the p.d.f. of a beta distribution with parameters α and β is { Γ(α+β) Γ(α)Γ(β) f(x α, β) xα 1 (1 x) β 1 for < x
p n r.01.05.10.15.20.25.30.35.40.45.50.55.60.65.70.75.80.85.90.95
 r r Table 4 Biomial Probability Distributio C, r p q This table shows the probability of r successes i idepedet trials, each with probability of success p. p r.01.05.10.15.0.5.30.35.40.45.50.55.60.65.70.75.80.85.90.95
r r Table 4 Biomial Probability Distributio C, r p q This table shows the probability of r successes i idepedet trials, each with probability of success p. p r.01.05.10.15.0.5.30.35.40.45.50.55.60.65.70.75.80.85.90.95
Χρονοσειρές Μάθημα 3
 Χρονοσειρές Μάθημα 3 Ασυσχέτιστες (λευκός θόρυβος) και ανεξάρτητες (iid) παρατηρήσεις Chafield C., The Analysis of Time Series, An Inroducion, 6 h ediion,. 38 (Chaer 3): Some auhors refer o make he weaker
Χρονοσειρές Μάθημα 3 Ασυσχέτιστες (λευκός θόρυβος) και ανεξάρτητες (iid) παρατηρήσεις Chafield C., The Analysis of Time Series, An Inroducion, 6 h ediion,. 38 (Chaer 3): Some auhors refer o make he weaker
( ) ( ) ( ) Fourier series. ; m is an integer. r(t) is periodic (T>0), r(t+t) = r(t), t Fundamental period T 0 = smallest T. Fundamental frequency ω
 Fourier series e jm when m d when m ; m is an ineger. jm jm jm jm e d e e e jm jm jm jm r( is periodi (>, r(+ r(, Fundamenal period smalles Fundamenal frequeny r ( + r ( is periodi hen M M e j M, e j,
Fourier series e jm when m d when m ; m is an ineger. jm jm jm jm e d e e e jm jm jm jm r( is periodi (>, r(+ r(, Fundamenal period smalles Fundamenal frequeny r ( + r ( is periodi hen M M e j M, e j,
A Bonus-Malus System as a Markov Set-Chain. Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics
 A Bonus-Malus System as a Markov Set-Chain Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics Contents 1. Markov set-chain 2. Model of bonus-malus system 3. Example 4. Conclusions
A Bonus-Malus System as a Markov Set-Chain Małgorzata Niemiec Warsaw School of Economics Institute of Econometrics Contents 1. Markov set-chain 2. Model of bonus-malus system 3. Example 4. Conclusions
CHAPTER 25 SOLVING EQUATIONS BY ITERATIVE METHODS
 CHAPTER 5 SOLVING EQUATIONS BY ITERATIVE METHODS EXERCISE 104 Page 8 1. Find the positive root of the equation x + 3x 5 = 0, correct to 3 significant figures, using the method of bisection. Let f(x) =
CHAPTER 5 SOLVING EQUATIONS BY ITERATIVE METHODS EXERCISE 104 Page 8 1. Find the positive root of the equation x + 3x 5 = 0, correct to 3 significant figures, using the method of bisection. Let f(x) =
TRM +4!5"2# 6!#!-!2&'!5$27!842//22&'9&2:1*;832<
 TRM!"#$%& ' *,-./ *!#!!%!&!3,&!$-!$./!!"#$%&'*" 4!5"# 6!#!-!&'!5$7!84//&'9&:*;83< #:4
TRM!"#$%& ' *,-./ *!#!!%!&!3,&!$-!$./!!"#$%&'*" 4!5"# 6!#!-!&'!5$7!84//&'9&:*;83< #:4
Μενύχτα, Πιπερίγκου, Σαββάτης. ΒΙΟΣΤΑΤΙΣΤΙΚΗ Εργαστήριο 5 ο
 Κατανομές Στατιστικών Συναρτήσεων Δύο ανεξάρτητα δείγματα από κανονική κατανομή Έστω Χ= ( Χ, Χ,..., Χ ) τ.δ. από Ν( µ, σ ) μεγέθους n και 1 n 1 1 Y = (Y, Y,..., Y ) τ.δ. από Ν( µ, σ ) 1 n 1 Χ Y ( µ µ )
Κατανομές Στατιστικών Συναρτήσεων Δύο ανεξάρτητα δείγματα από κανονική κατανομή Έστω Χ= ( Χ, Χ,..., Χ ) τ.δ. από Ν( µ, σ ) μεγέθους n και 1 n 1 1 Y = (Y, Y,..., Y ) τ.δ. από Ν( µ, σ ) 1 n 1 Χ Y ( µ µ )
ω = radians per sec, t = 3 sec
 Secion. Linear and Angular Speed 7. From exercise, =. A= r A = ( 00 ) (. ) = 7,00 in 7. Since 7 is in quadran IV, he reference 7 8 7 angle is = =. In quadran IV, he cosine is posiive. Thus, 7 cos = cos
Secion. Linear and Angular Speed 7. From exercise, =. A= r A = ( 00 ) (. ) = 7,00 in 7. Since 7 is in quadran IV, he reference 7 8 7 angle is = =. In quadran IV, he cosine is posiive. Thus, 7 cos = cos
$ι ιι η ι ι!η ηι ι ANOVA. To ANOVA ι ι ι η η η ιη (Analysis of Variance). * ι! ι ι ι ι ι η ιη. ;, ι ι ι! η ιι ηιη ι ι!η ι η η ιη ι ι η ι η.
 η &, 7!# v # $ι ιι η ι ι!η ηι ι ANOVA. To ANOVA ι ι ι η η η ιη (Analysis of Variance). * ι! ι ι ι ι ι η ιη. ;, ι ι ι! η ιι ηιη ι ι!η ι η η ιη ι ι η ι η. - ι% ιι* ι' F ι ι ι% MS F MS between within MS MS
η &, 7!# v # $ι ιι η ι ι!η ηι ι ANOVA. To ANOVA ι ι ι η η η ιη (Analysis of Variance). * ι! ι ι ι ι ι η ιη. ;, ι ι ι! η ιι ηιη ι ι!η ι η η ιη ι ι η ι η. - ι% ιι* ι' F ι ι ι% MS F MS between within MS MS
Math 6 SL Probability Distributions Practice Test Mark Scheme
 Math 6 SL Probability Distributions Practice Test Mark Scheme. (a) Note: Award A for vertical line to right of mean, A for shading to right of their vertical line. AA N (b) evidence of recognizing symmetry
Math 6 SL Probability Distributions Practice Test Mark Scheme. (a) Note: Award A for vertical line to right of mean, A for shading to right of their vertical line. AA N (b) evidence of recognizing symmetry
Εισαγωγή στην Ανάλυση Διακύμανσης
 Εισαγωγή στην Ανάλυση Διακύμανσης 1 Η Ανάλυση Διακύμανσης Από τα πιο συχνά χρησιμοποιούμενα στατιστικά κριτήρια στην κοινωνική έρευνα Γιατί; 1. Ενώ αναφέρεται σε διαφορές μέσων όρων, όπως και το κριτήριο
Εισαγωγή στην Ανάλυση Διακύμανσης 1 Η Ανάλυση Διακύμανσης Από τα πιο συχνά χρησιμοποιούμενα στατιστικά κριτήρια στην κοινωνική έρευνα Γιατί; 1. Ενώ αναφέρεται σε διαφορές μέσων όρων, όπως και το κριτήριο
Επιστηµονική Επιµέλεια ρ. Γεώργιος Μενεξές. Εργαστήριο Γεωργίας. Viola adorata
 One-way ANOVA µε το SPSS Επιστηµονική Επιµέλεια ρ. Γεώργιος Μενεξές Τοµέας Φυτών Μεγάλης Καλλιέργειας και Οικολογίας, Εργαστήριο Γεωργίας Viola adorata To call in a statistician after the experiment is
One-way ANOVA µε το SPSS Επιστηµονική Επιµέλεια ρ. Γεώργιος Μενεξές Τοµέας Φυτών Μεγάλης Καλλιέργειας και Οικολογίας, Εργαστήριο Γεωργίας Viola adorata To call in a statistician after the experiment is
k A = [k, k]( )[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R +
[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R + k A = [k, k]( )[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R +](/thumbs/73/69566903.jpg) Chapter 3. Fuzzy Arithmetic 3- Fuzzy arithmetic: ~Addition(+) and subtraction (-): Let A = [a and B = [b, b in R If x [a and y [b, b than x+y [a +b +b Symbolically,we write A(+)B = [a (+)[b, b = [a +b
Chapter 3. Fuzzy Arithmetic 3- Fuzzy arithmetic: ~Addition(+) and subtraction (-): Let A = [a and B = [b, b in R If x [a and y [b, b than x+y [a +b +b Symbolically,we write A(+)B = [a (+)[b, b = [a +b
τατιςτική ςτην Εκπαίδευςη II
 ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΣΙΑ ΠΑΝΕΠΙΣΗΜΙΟ ΚΡΗΣΗ τατιςτική ςτην Εκπαίδευςη II Αρχείο αποτελεςμάτων Διδάσκων: Μιχάλης Λιναρδάκης ΠΑΙΔΑΓΩΓΙΚΟ ΤΜΗΜΑ ΔΗΜΟΤΙΚΗΣ ΕΚΠΑΙΔΕΥΣΗΣ ΣΧΟΛΗ ΕΠΙΣΤΗΜΩΝ ΑΓΩΓΗΣ Άδειες Χρήσης Το παρόν
ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΣΙΑ ΠΑΝΕΠΙΣΗΜΙΟ ΚΡΗΣΗ τατιςτική ςτην Εκπαίδευςη II Αρχείο αποτελεςμάτων Διδάσκων: Μιχάλης Λιναρδάκης ΠΑΙΔΑΓΩΓΙΚΟ ΤΜΗΜΑ ΔΗΜΟΤΙΚΗΣ ΕΚΠΑΙΔΕΥΣΗΣ ΣΧΟΛΗ ΕΠΙΣΤΗΜΩΝ ΑΓΩΓΗΣ Άδειες Χρήσης Το παρόν
Common Families of Distributions
 Chaper 3 Common Families of Disribuions 3 The pmf of X is f N N +, N, N +,, N Then EX N N N N + N + N N N N + N N N + N N + N N + Similarly, using he formula for N, we obain E N N +N + N N N N N + 6 VarX
Chaper 3 Common Families of Disribuions 3 The pmf of X is f N N +, N, N +,, N Then EX N N N N + N + N N N N + N N N + N N + N N + Similarly, using he formula for N, we obain E N N +N + N N N N N + 6 VarX
Answers - Worksheet A ALGEBRA PMT. 1 a = 7 b = 11 c = 1 3. e = 0.1 f = 0.3 g = 2 h = 10 i = 3 j = d = k = 3 1. = 1 or 0.5 l =
 C ALGEBRA Answers - Worksheet A a 7 b c d e 0. f 0. g h 0 i j k 6 8 or 0. l or 8 a 7 b 0 c 7 d 6 e f g 6 h 8 8 i 6 j k 6 l a 9 b c d 9 7 e 00 0 f 8 9 a b 7 7 c 6 d 9 e 6 6 f 6 8 g 9 h 0 0 i j 6 7 7 k 9
C ALGEBRA Answers - Worksheet A a 7 b c d e 0. f 0. g h 0 i j k 6 8 or 0. l or 8 a 7 b 0 c 7 d 6 e f g 6 h 8 8 i 6 j k 6 l a 9 b c d 9 7 e 00 0 f 8 9 a b 7 7 c 6 d 9 e 6 6 f 6 8 g 9 h 0 0 i j 6 7 7 k 9
ANSWERSHEET (TOPIC = DIFFERENTIAL CALCULUS) COLLECTION #2. h 0 h h 0 h h 0 ( ) g k = g 0 + g 1 + g g 2009 =?
 Teko Classes IITJEE/AIEEE Maths by SUHAAG SIR, Bhopal, Ph (0755) 3 00 000 www.tekoclasses.com ANSWERSHEET (TOPIC DIFFERENTIAL CALCULUS) COLLECTION # Question Type A.Single Correct Type Q. (A) Sol least
Teko Classes IITJEE/AIEEE Maths by SUHAAG SIR, Bhopal, Ph (0755) 3 00 000 www.tekoclasses.com ANSWERSHEET (TOPIC DIFFERENTIAL CALCULUS) COLLECTION # Question Type A.Single Correct Type Q. (A) Sol least
τατιστική στην Εκπαίδευση II
 ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΣΙΑ ΠΑΝΕΠΙΣΗΜΙΟ ΚΡΗΣΗ τατιστική στην Εκπαίδευση II Λφση επαναληπτικής άσκησης Διδάσκων: Μιχάλης Λιναρδάκης ΠΑΙΔΑΓΩΓΙΚΟ ΤΜΗΜΑ ΔΗΜΟΤΙΚΗΣ ΕΚΠΑΙΔΕΥΣΗΣ ΣΧΟΛΗ ΕΠΙΣΤΗΜΩΝ ΑΓΩΓΗΣ Άδειες Χρήσης Το
ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΣΙΑ ΠΑΝΕΠΙΣΗΜΙΟ ΚΡΗΣΗ τατιστική στην Εκπαίδευση II Λφση επαναληπτικής άσκησης Διδάσκων: Μιχάλης Λιναρδάκης ΠΑΙΔΑΓΩΓΙΚΟ ΤΜΗΜΑ ΔΗΜΟΤΙΚΗΣ ΕΚΠΑΙΔΕΥΣΗΣ ΣΧΟΛΗ ΕΠΙΣΤΗΜΩΝ ΑΓΩΓΗΣ Άδειες Χρήσης Το
PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI
 155 Lampiran 6 Yayan Sumaryana, 2014 PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI Universitas Pendidikan Indonesia
155 Lampiran 6 Yayan Sumaryana, 2014 PENGARUHKEPEMIMPINANINSTRUKSIONAL KEPALASEKOLAHDAN MOTIVASI BERPRESTASI GURU TERHADAP KINERJA MENGAJAR GURU SD NEGERI DI KOTA SUKABUMI Universitas Pendidikan Indonesia
Μαντζούνη, Πιπερίγκου, Χατζή. ΒΙΟΣΤΑΤΙΣΤΙΚΗ Εργαστήριο 5 ο
 Κατανομές Στατιστικών Συναρτήσεων Δύο δείγματα από κανονική κατανομή Έστω Χ= ( Χ, Χ,..., Χ ) τ.δ. από Ν( µ, σ ) μεγέθους n και 1 n 1 1 Y = (Y, Y,...,Y ) τ.δ. από Ν( µ, σ ) 1 n 1 Χ Y ( µ µ ) S σ Τ ( Χ,Y)
Κατανομές Στατιστικών Συναρτήσεων Δύο δείγματα από κανονική κατανομή Έστω Χ= ( Χ, Χ,..., Χ ) τ.δ. από Ν( µ, σ ) μεγέθους n και 1 n 1 1 Y = (Y, Y,...,Y ) τ.δ. από Ν( µ, σ ) 1 n 1 Χ Y ( µ µ ) S σ Τ ( Χ,Y)
t-distribution t a (ν) s N μ = where X s s x = ν 2 FD ν 1 FD a/2 a/2 t-distribution normal distribution for ν>120
 t-ditribution t X x μ = where x = ν FD ν FD t a (ν) 0 t-ditribution normal ditribution for ν>0 a/ a/ -ta ta ΒΑΘΜΟΙ ΕΛΕΥΘΕΡΙΑΣ (freedom degree) Βαθμοί ελευθερίας (ν): ο αριθμός των ανεξάρτητων μετρήσεων
t-ditribution t X x μ = where x = ν FD ν FD t a (ν) 0 t-ditribution normal ditribution for ν>0 a/ a/ -ta ta ΒΑΘΜΟΙ ΕΛΕΥΘΕΡΙΑΣ (freedom degree) Βαθμοί ελευθερίας (ν): ο αριθμός των ανεξάρτητων μετρήσεων
ΕΙΣΑΓΩΓΗ ΣΤΗ ΣΤΑΤΙΣΤΙΚΗ ΑΝΑΛΥΣΗ
 ΕΙΣΑΓΩΓΗ ΣΤΗ ΣΤΑΤΙΣΤΙΚΗ ΑΝΑΛΥΣΗ ΕΛΕΝΑ ΦΛΟΚΑ Επίκουρος Καθηγήτρια Τµήµα Φυσικής, Τοµέας Φυσικής Περιβάλλοντος- Μετεωρολογίας ΓΕΝΙΚΟΙ ΟΡΙΣΜΟΙ Πληθυσµός Σύνολο ατόµων ή αντικειµένων στα οποία αναφέρονται
ΕΙΣΑΓΩΓΗ ΣΤΗ ΣΤΑΤΙΣΤΙΚΗ ΑΝΑΛΥΣΗ ΕΛΕΝΑ ΦΛΟΚΑ Επίκουρος Καθηγήτρια Τµήµα Φυσικής, Τοµέας Φυσικής Περιβάλλοντος- Μετεωρολογίας ΓΕΝΙΚΟΙ ΟΡΙΣΜΟΙ Πληθυσµός Σύνολο ατόµων ή αντικειµένων στα οποία αναφέρονται
Statistics & Research methods. Athanasios Papaioannou University of Thessaly Dept. of PE & Sport Science
 Statistics & Research methods Athanasios Papaioannou University of Thessaly Dept. of PE & Sport Science 30 25 1,65 20 1,66 15 10 5 1,67 1,68 Κανονική 0 Height 1,69 Καμπύλη Κανονική Διακύμανση & Ζ-scores
Statistics & Research methods Athanasios Papaioannou University of Thessaly Dept. of PE & Sport Science 30 25 1,65 20 1,66 15 10 5 1,67 1,68 Κανονική 0 Height 1,69 Καμπύλη Κανονική Διακύμανση & Ζ-scores
ΜΕΤΑΣΧΗΜΑΤΙΣΜΟΙ ΣΕΙΡΩΝ
 ΜΕΤΑΣΧΗΜΑΤΙΣΜΟΙ ΣΕΙΡΩΝ Με δεδομένη μια σειρά μπορούμε να εφαρμόσουμε διάφορους μετασχηματισμούς που είναι χρήσιμοι στους στατιστικούς και οικονομετρικούς υπολογισμούς. Οι πιο συνηθισμένοι υπολογισμοί είναι
ΜΕΤΑΣΧΗΜΑΤΙΣΜΟΙ ΣΕΙΡΩΝ Με δεδομένη μια σειρά μπορούμε να εφαρμόσουμε διάφορους μετασχηματισμούς που είναι χρήσιμοι στους στατιστικούς και οικονομετρικούς υπολογισμούς. Οι πιο συνηθισμένοι υπολογισμοί είναι
CHAPTER 48 APPLICATIONS OF MATRICES AND DETERMINANTS
 CHAPTER 48 APPLICATIONS OF MATRICES AND DETERMINANTS EXERCISE 01 Page 545 1. Use matrices to solve: 3x + 4y x + 5y + 7 3x + 4y x + 5y 7 Hence, 3 4 x 0 5 y 7 The inverse of 3 4 5 is: 1 5 4 1 5 4 15 8 3
CHAPTER 48 APPLICATIONS OF MATRICES AND DETERMINANTS EXERCISE 01 Page 545 1. Use matrices to solve: 3x + 4y x + 5y + 7 3x + 4y x + 5y 7 Hence, 3 4 x 0 5 y 7 The inverse of 3 4 5 is: 1 5 4 1 5 4 15 8 3
The canonical 2nd order transfer function is expressed as. (ω n
 Second order ransfer funcions nd Order ransfer funcion - Summary of resuls The canonical nd order ransfer funcion is expressed as H(s) s + ζ s + is he naural frequency; ζ is he damping coefficien. The
Second order ransfer funcions nd Order ransfer funcion - Summary of resuls The canonical nd order ransfer funcion is expressed as H(s) s + ζ s + is he naural frequency; ζ is he damping coefficien. The
Harold s Statistics Probability Density Functions Cheat Sheet 30 May PDF Selection Tree to Describe a Single Population
 Harold s Statistics Probability Density Functions Cheat Sheet 30 May 2016 PDF Selection Tree to Describe a Single Population Qualitative Quantitative Copyright 2016 by Harold Toomey, WyzAnt Tutor 1 Discrete
Harold s Statistics Probability Density Functions Cheat Sheet 30 May 2016 PDF Selection Tree to Describe a Single Population Qualitative Quantitative Copyright 2016 by Harold Toomey, WyzAnt Tutor 1 Discrete
Δείγμα (μεγάλο) από οποιαδήποτε κατανομή
 ΒΙΟΣΤΑΤΙΣΤΙΚΗ Εργαστήριο 4ο Κατανομές Στατιστικών Συναρτήσεων Δείγμα από κανονική κατανομή Έστω Χ= Χ Χ Χ τ.δ. από Ν µσ τότε ( 1,,..., n) (, ) Τ Χ Χ Ν Τ Χ σ σ Χ Τ Χ n Χ S µ S µ 1( ) = (0,1), ( ) = ( n 1)
ΒΙΟΣΤΑΤΙΣΤΙΚΗ Εργαστήριο 4ο Κατανομές Στατιστικών Συναρτήσεων Δείγμα από κανονική κατανομή Έστω Χ= Χ Χ Χ τ.δ. από Ν µσ τότε ( 1,,..., n) (, ) Τ Χ Χ Ν Τ Χ σ σ Χ Τ Χ n Χ S µ S µ 1( ) = (0,1), ( ) = ( n 1)
2. THEORY OF EQUATIONS. PREVIOUS EAMCET Bits.
 EAMCET-. THEORY OF EQUATIONS PREVIOUS EAMCET Bits. Each of the roots of the equation x 6x + 6x 5= are increased by k so that the new transformed equation does not contain term. Then k =... - 4. - Sol.
EAMCET-. THEORY OF EQUATIONS PREVIOUS EAMCET Bits. Each of the roots of the equation x 6x + 6x 5= are increased by k so that the new transformed equation does not contain term. Then k =... - 4. - Sol.
Πρόβλημα 1: Αναζήτηση Ελάχιστης/Μέγιστης Τιμής
 Πρόβλημα 1: Αναζήτηση Ελάχιστης/Μέγιστης Τιμής Να γραφεί πρόγραμμα το οποίο δέχεται ως είσοδο μια ακολουθία S από n (n 40) ακέραιους αριθμούς και επιστρέφει ως έξοδο δύο ακολουθίες από θετικούς ακέραιους
Πρόβλημα 1: Αναζήτηση Ελάχιστης/Μέγιστης Τιμής Να γραφεί πρόγραμμα το οποίο δέχεται ως είσοδο μια ακολουθία S από n (n 40) ακέραιους αριθμούς και επιστρέφει ως έξοδο δύο ακολουθίες από θετικούς ακέραιους
Μηχανική Μάθηση Hypothesis Testing
 ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΤΙΑ ΠΑΝΕΠΙΣΤΗΜΙΟ ΚΡΗΤΗΣ Μηχανική Μάθηση Hypothesis Testing Γιώργος Μπορμπουδάκης Τμήμα Επιστήμης Υπολογιστών Procedure 1. Form the null (H 0 ) and alternative (H 1 ) hypothesis 2. Consider
ΕΛΛΗΝΙΚΗ ΔΗΜΟΚΡΑΤΙΑ ΠΑΝΕΠΙΣΤΗΜΙΟ ΚΡΗΤΗΣ Μηχανική Μάθηση Hypothesis Testing Γιώργος Μπορμπουδάκης Τμήμα Επιστήμης Υπολογιστών Procedure 1. Form the null (H 0 ) and alternative (H 1 ) hypothesis 2. Consider
Does anemia contribute to end-organ dysfunction in ICU patients Statistical Analysis
 Does anemia contribute to end-organ dysfunction in ICU patients Statistical Analysis Xue Han, MPH and Matt Shotwell, PhD Department of Biostatistics Vanderbilt University School of Medicine March 14, 2014
Does anemia contribute to end-organ dysfunction in ICU patients Statistical Analysis Xue Han, MPH and Matt Shotwell, PhD Department of Biostatistics Vanderbilt University School of Medicine March 14, 2014
( ) ( t) ( 0) ( ) dw w. = = β. Then the solution of (1.1) is easily found to. wt = t+ t. We generalize this to the following nonlinear differential
 Periodic oluion of van der Pol differenial equaion. by A. Arimoo Deparmen of Mahemaic Muahi Iniue of Technology Tokyo Japan in Seminar a Kiami Iniue of Technology January 8 9. Inroducion Le u conider a
Periodic oluion of van der Pol differenial equaion. by A. Arimoo Deparmen of Mahemaic Muahi Iniue of Technology Tokyo Japan in Seminar a Kiami Iniue of Technology January 8 9. Inroducion Le u conider a
χ 2 test ανεξαρτησίας
 χ 2 test ανεξαρτησίας Καθηγητής Ι. Κ. ΔΗΜΗΤΡΙΟΥ demetri@econ.uoa.gr 7.2 Το χ 2 Τεστ Ανεξαρτησίας Tο χ 2 τεστ ανεξαρτησίας (όπως και η παλινδρόμηση) είναι στατιστικά εργαλεία για τον εντοπισμό σχέσεων μεταξύ
χ 2 test ανεξαρτησίας Καθηγητής Ι. Κ. ΔΗΜΗΤΡΙΟΥ demetri@econ.uoa.gr 7.2 Το χ 2 Τεστ Ανεξαρτησίας Tο χ 2 τεστ ανεξαρτησίας (όπως και η παλινδρόμηση) είναι στατιστικά εργαλεία για τον εντοπισμό σχέσεων μεταξύ
Παράδειγμα: Γούργουλης Βασίλειος, Επίκουρος Καθηγητής Τ.Ε.Φ.Α.Α. Δ.Π.Θ.
 Έλεγχος ύπαρξης στατιστικά σημαντικών διαφορών μεταξύ περισσότερων από δύο ανεξάρτητων δειγμάτων, που διαχωρίζονται βάσει ενός ανεξάρτητου παράγοντα (Ανάλυση διακύμανσης για ανεξάρτητα δείγματα ως προς
Έλεγχος ύπαρξης στατιστικά σημαντικών διαφορών μεταξύ περισσότερων από δύο ανεξάρτητων δειγμάτων, που διαχωρίζονται βάσει ενός ανεξάρτητου παράγοντα (Ανάλυση διακύμανσης για ανεξάρτητα δείγματα ως προς
Γ. Κορίλη, Μοντέλα Εξυπηρέτησης
 Γ. Κορίλη, Μοντέλα Εξυπηρέτησης 2-1 hp://www.seas.upenn.edu/~com501/lecures/lecure3.pdf Καθυστερήσεις στα ίκτυα Πακέτων Εισαγωγή στη Θεωρία Ουρών Ανασκόπηση Θεωρίας Πιθανοτήτων ιαδικασία Poisson Θεώρηµα
Γ. Κορίλη, Μοντέλα Εξυπηρέτησης 2-1 hp://www.seas.upenn.edu/~com501/lecures/lecure3.pdf Καθυστερήσεις στα ίκτυα Πακέτων Εισαγωγή στη Θεωρία Ουρών Ανασκόπηση Θεωρίας Πιθανοτήτων ιαδικασία Poisson Θεώρηµα
SOLUTIONS & ANSWERS FOR KERALA ENGINEERING ENTRANCE EXAMINATION-2018 PAPER II VERSION B1
 SOLUTIONS & ANSWERS FOR KERALA ENGINEERING ENTRANCE EXAMINATION-8 PAPER II VERSION B [MATHEMATICS]. Ans: ( i) It is (cs5 isin5 ) ( i). Ans: i z. Ans: i i i The epressin ( i) ( ). Ans: cs i sin cs i sin
SOLUTIONS & ANSWERS FOR KERALA ENGINEERING ENTRANCE EXAMINATION-8 PAPER II VERSION B [MATHEMATICS]. Ans: ( i) It is (cs5 isin5 ) ( i). Ans: i z. Ans: i i i The epressin ( i) ( ). Ans: cs i sin cs i sin
Appendix. The solution begins with Eq. (2.15) from the text, which we repeat here for 1, (A.1)
 Aenix Aenix A: The equaion o he sock rice. The soluion egins wih Eq..5 rom he ex, which we reea here or convenience as Eq.A.: [ [ E E X, A. c α where X u ε, α γ, an c α y AR. Take execaions o Eq. A. as
Aenix Aenix A: The equaion o he sock rice. The soluion egins wih Eq..5 rom he ex, which we reea here or convenience as Eq.A.: [ [ E E X, A. c α where X u ε, α γ, an c α y AR. Take execaions o Eq. A. as
The conditional CAPM does not explain assetpricing. Jonathan Lewellen & Stefan Nagel. HEC School of Management, March 17, 2005
 The condiional CAPM does no explain assepricing anomalies Jonahan Lewellen & Sefan Nagel HEC School of Managemen, March 17, 005 Background Size, B/M, and momenum porfolios, 1964 001 Monhly reurns (%) Avg.
The condiional CAPM does no explain assepricing anomalies Jonahan Lewellen & Sefan Nagel HEC School of Managemen, March 17, 005 Background Size, B/M, and momenum porfolios, 1964 001 Monhly reurns (%) Avg.
Probability and Random Processes (Part II)
 Probability and Random Processes (Part II) 1. If the variance σ x of d(n) = x(n) x(n 1) is one-tenth the variance σ x of a stationary zero-mean discrete-time signal x(n), then the normalized autocorrelation
Probability and Random Processes (Part II) 1. If the variance σ x of d(n) = x(n) x(n 1) is one-tenth the variance σ x of a stationary zero-mean discrete-time signal x(n), then the normalized autocorrelation
Second Order Partial Differential Equations
 Chapter 7 Second Order Partial Differential Equations 7.1 Introduction A second order linear PDE in two independent variables (x, y Ω can be written as A(x, y u x + B(x, y u xy + C(x, y u u u + D(x, y
Chapter 7 Second Order Partial Differential Equations 7.1 Introduction A second order linear PDE in two independent variables (x, y Ω can be written as A(x, y u x + B(x, y u xy + C(x, y u u u + D(x, y
16. 17. r t te 2t i t 1. 18 19 Find the derivative of the vector function. 19. r t e t cos t i e t sin t j ln t k. 31 33 Evaluate the integral.
 SECTION.7 VECTOR FUNCTIONS AND SPACE CURVES.7 VECTOR FUNCTIONS AND SPACE CURVES A Click here for answers. S Click here for soluions. Copyrigh Cengage Learning. All righs reserved.. Find he domain of he
SECTION.7 VECTOR FUNCTIONS AND SPACE CURVES.7 VECTOR FUNCTIONS AND SPACE CURVES A Click here for answers. S Click here for soluions. Copyrigh Cengage Learning. All righs reserved.. Find he domain of he
3.4 SUM AND DIFFERENCE FORMULAS. NOTE: cos(α+β) cos α + cos β cos(α-β) cos α -cos β
 3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
List MF20. List of Formulae and Statistical Tables. Cambridge Pre-U Mathematics (9794) and Further Mathematics (9795)
 List MF0 List of Formulae and Statistical Tables Cambridge Pre-U Mathematics (979) and Further Mathematics (979) For use from 07 in all aers for the above syllabuses. CST7 Mensuration Surface area of shere
List MF0 List of Formulae and Statistical Tables Cambridge Pre-U Mathematics (979) and Further Mathematics (979) For use from 07 in all aers for the above syllabuses. CST7 Mensuration Surface area of shere
Άσκηση 10, σελ. 119. Για τη μεταβλητή x (άτυπος όγκος) έχουμε: x censored_x 1 F 3 F 3 F 4 F 10 F 13 F 13 F 16 F 16 F 24 F 26 F 27 F 28 F
 Άσκηση 0, σελ. 9 από το βιβλίο «Μοντέλα Αξιοπιστίας και Επιβίωσης» της Χ. Καρώνη (i) Αρχικά, εισάγουμε τα δεδομένα στο minitab δημιουργώντας δύο μεταβλητές: τη x για τον άτυπο όγκο και την y για τον τυπικό
Άσκηση 0, σελ. 9 από το βιβλίο «Μοντέλα Αξιοπιστίας και Επιβίωσης» της Χ. Καρώνη (i) Αρχικά, εισάγουμε τα δεδομένα στο minitab δημιουργώντας δύο μεταβλητές: τη x για τον άτυπο όγκο και την y για τον τυπικό
1. Hasil Pengukuran Kadar TNF-α. DATA PENGAMATAN ABSORBANSI STANDAR TNF α PADA PANJANG GELOMBANG 450 nm
 HASIL PENELITIAN 1. Hasil Pengukuran Kadar TNF-α DATA PENGAMATAN ABSORBANSI STANDAR TNF α PADA PANJANG GELOMBANG 450 nm NO KADAR ( pg/ml) ABSORBANSI 1. 0 0.055 2. 15.6 0.207 3. 31.5 0.368 4. 62.5 0.624
HASIL PENELITIAN 1. Hasil Pengukuran Kadar TNF-α DATA PENGAMATAN ABSORBANSI STANDAR TNF α PADA PANJANG GELOMBANG 450 nm NO KADAR ( pg/ml) ABSORBANSI 1. 0 0.055 2. 15.6 0.207 3. 31.5 0.368 4. 62.5 0.624
Quadratic Expressions
 Quadratic Expressions. The standard form of a quadratic equation is ax + bx + c = 0 where a, b, c R and a 0. The roots of ax + bx + c = 0 are b ± b a 4ac. 3. For the equation ax +bx+c = 0, sum of the roots
Quadratic Expressions. The standard form of a quadratic equation is ax + bx + c = 0 where a, b, c R and a 0. The roots of ax + bx + c = 0 are b ± b a 4ac. 3. For the equation ax +bx+c = 0, sum of the roots
1991 US Social Survey.sav
 Παραδείγµατα στατιστικής συµπερασµατολογίας µε ένα δείγµα Στα παραδείγµατα χρησιµοποιείται απλό τυχαίο δείγµα µεγέθους 1 από το αρχείο δεδοµένων 1991 US Social Survey.sav Το δείγµα λαµβάνεται µε την διαδικασία
Παραδείγµατα στατιστικής συµπερασµατολογίας µε ένα δείγµα Στα παραδείγµατα χρησιµοποιείται απλό τυχαίο δείγµα µεγέθους 1 από το αρχείο δεδοµένων 1991 US Social Survey.sav Το δείγµα λαµβάνεται µε την διαδικασία
Queensland University of Technology Transport Data Analysis and Modeling Methodologies
 Queensland University of Technology Transport Data Analysis and Modeling Methodologies Lab Session #7 Example 5.2 (with 3SLS Extensions) Seemingly Unrelated Regression Estimation and 3SLS A survey of 206
Queensland University of Technology Transport Data Analysis and Modeling Methodologies Lab Session #7 Example 5.2 (with 3SLS Extensions) Seemingly Unrelated Regression Estimation and 3SLS A survey of 206
Table A.1 Random numbers (section 1)
 A Tables Table Contents Page A.1 Random numbers 696 A.2 Orthogonal polynomial trend contrast coefficients 702 A.3 Standard normal distribution 703 A.4 Student s t-distribution 704 A.5 Chi-squared distribution
A Tables Table Contents Page A.1 Random numbers 696 A.2 Orthogonal polynomial trend contrast coefficients 702 A.3 Standard normal distribution 703 A.4 Student s t-distribution 704 A.5 Chi-squared distribution
If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2
![If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2 If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2](/thumbs/53/31933086.jpg) Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
( ) ( ) STAT 5031 Statistical Methods for Quality Improvement. Homework n = 8; x = 127 psi; σ = 2 psi (a) µ 0 = 125; α = 0.
 STAT 531 Statistical Methods for Quality Improvement Homework 3 4.8 n = 8; x = 17 psi; σ = psi (a) µ = 15; α =.5 Test H : µ = 15 vs. H 1 : µ > 15. Reject H if Z > Z α. x µ 17 15 Z = = =.88 σ n 8 Z α =
STAT 531 Statistical Methods for Quality Improvement Homework 3 4.8 n = 8; x = 17 psi; σ = psi (a) µ = 15; α =.5 Test H : µ = 15 vs. H 1 : µ > 15. Reject H if Z > Z α. x µ 17 15 Z = = =.88 σ n 8 Z α =
794 Appendix A:Tables
 Appendix A Tables A Table Contents Page A.1 Random numbers 794 A.2 Orthogonal polynomial trend contrast coefficients 800 A.3 Standard normal distribution 801 A.4 Student s t-distribution 802 A.5 Chi-squared
Appendix A Tables A Table Contents Page A.1 Random numbers 794 A.2 Orthogonal polynomial trend contrast coefficients 800 A.3 Standard normal distribution 801 A.4 Student s t-distribution 802 A.5 Chi-squared
Υπολογιστική Φυσική Στοιχειωδών Σωματιδίων
 Υπολογιστική Φυσική Στοιχειωδών Σωματιδίων Όρια Πιστότητας (Confidence Limits) 2/4/2014 Υπολογ.Φυσική ΣΣ 1 Τα όρια πιστότητας -Confidence Limits (CL) Tα όρια πιστότητας μιας μέτρησης Μπορεί να αναφέρονται
Υπολογιστική Φυσική Στοιχειωδών Σωματιδίων Όρια Πιστότητας (Confidence Limits) 2/4/2014 Υπολογ.Φυσική ΣΣ 1 Τα όρια πιστότητας -Confidence Limits (CL) Tα όρια πιστότητας μιας μέτρησης Μπορεί να αναφέρονται
Main source: "Discrete-time systems and computer control" by Α. ΣΚΟΔΡΑΣ ΨΗΦΙΑΚΟΣ ΕΛΕΓΧΟΣ ΔΙΑΛΕΞΗ 4 ΔΙΑΦΑΝΕΙΑ 1
 Main source: "Discrete-time systems and computer control" by Α. ΣΚΟΔΡΑΣ ΨΗΦΙΑΚΟΣ ΕΛΕΓΧΟΣ ΔΙΑΛΕΞΗ 4 ΔΙΑΦΑΝΕΙΑ 1 A Brief History of Sampling Research 1915 - Edmund Taylor Whittaker (1873-1956) devised a
Main source: "Discrete-time systems and computer control" by Α. ΣΚΟΔΡΑΣ ΨΗΦΙΑΚΟΣ ΕΛΕΓΧΟΣ ΔΙΑΛΕΞΗ 4 ΔΙΑΦΑΝΕΙΑ 1 A Brief History of Sampling Research 1915 - Edmund Taylor Whittaker (1873-1956) devised a
If we restrict the domain of y = sin x to [ π 2, π 2
 Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ. 5. Στατιστική συµπερασµατολογία για ποσοτικές µεταβλητές: Έλεγχοι υποθέσεων και διαστήµατα εµπιστοσύνης
 ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ 5. Στατιστική συµπερασµατολογία για ποσοτικές µεταβλητές: Έλεγχοι υποθέσεων και διαστήµατα εµπιστοσύνης ιαστήµατα εµπιστοσύνης και έλεγχοι υποθέσεων για τη µέση τιµή Για µια ποσοτική µεταβλητή
ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ 5. Στατιστική συµπερασµατολογία για ποσοτικές µεταβλητές: Έλεγχοι υποθέσεων και διαστήµατα εµπιστοσύνης ιαστήµατα εµπιστοσύνης και έλεγχοι υποθέσεων για τη µέση τιµή Για µια ποσοτική µεταβλητή
Review Test 3. MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question.
 Review Test MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question. Find the exact value of the expression. 1) sin - 11π 1 1) + - + - - ) sin 11π 1 ) ( -
Review Test MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question. Find the exact value of the expression. 1) sin - 11π 1 1) + - + - - ) sin 11π 1 ) ( -
519.22(07.07) 78 : ( ) /.. ; c (07.07) , , 2008
 .. ( ) 2008 519.22(07.07) 78 : ( ) /.. ;. : -, 2008. 38 c. ( ) STATISTICA.,. STATISTICA.,. 519.22(07.07),.., 2008.., 2008., 2008 2 ... 4 1...5...5 2...14...14 3...27...27 3 ,, -. " ", :,,,... STATISTICA.,,,.
.. ( ) 2008 519.22(07.07) 78 : ( ) /.. ;. : -, 2008. 38 c. ( ) STATISTICA.,. STATISTICA.,. 519.22(07.07),.., 2008.., 2008., 2008 2 ... 4 1...5...5 2...14...14 3...27...27 3 ,, -. " ", :,,,... STATISTICA.,,,.
6.3 Forecasting ARMA processes
 122 CHAPTER 6. ARMA MODELS 6.3 Forecasting ARMA processes The purpose of forecasting is to predict future values of a TS based on the data collected to the present. In this section we will discuss a linear
122 CHAPTER 6. ARMA MODELS 6.3 Forecasting ARMA processes The purpose of forecasting is to predict future values of a TS based on the data collected to the present. In this section we will discuss a linear
CRASH COURSE IN PRECALCULUS
 CRASH COURSE IN PRECALCULUS Shiah-Sen Wang The graphs are prepared by Chien-Lun Lai Based on : Precalculus: Mathematics for Calculus by J. Stuwart, L. Redin & S. Watson, 6th edition, 01, Brooks/Cole Chapter
CRASH COURSE IN PRECALCULUS Shiah-Sen Wang The graphs are prepared by Chien-Lun Lai Based on : Precalculus: Mathematics for Calculus by J. Stuwart, L. Redin & S. Watson, 6th edition, 01, Brooks/Cole Chapter
Homework 8 Model Solution Section
 MATH 004 Homework Solution Homework 8 Model Solution Section 14.5 14.6. 14.5. Use the Chain Rule to find dz where z cosx + 4y), x 5t 4, y 1 t. dz dx + dy y sinx + 4y)0t + 4) sinx + 4y) 1t ) 0t + 4t ) sinx
MATH 004 Homework Solution Homework 8 Model Solution Section 14.5 14.6. 14.5. Use the Chain Rule to find dz where z cosx + 4y), x 5t 4, y 1 t. dz dx + dy y sinx + 4y)0t + 4) sinx + 4y) 1t ) 0t + 4t ) sinx
Bayesian statistics. DS GA 1002 Probability and Statistics for Data Science.
 Bayesian statistics DS GA 1002 Probability and Statistics for Data Science http://www.cims.nyu.edu/~cfgranda/pages/dsga1002_fall17 Carlos Fernandez-Granda Frequentist vs Bayesian statistics In frequentist
Bayesian statistics DS GA 1002 Probability and Statistics for Data Science http://www.cims.nyu.edu/~cfgranda/pages/dsga1002_fall17 Carlos Fernandez-Granda Frequentist vs Bayesian statistics In frequentist
Έλεγχος ύπαρξης στατιστικά σημαντικών διαφορών μεταξύ δύο ανεξάρτητων δειγμάτων, που ακολουθούν την κανονική κατανομή (t-test για ανεξάρτητα δείγματα)
 Έλεγχος ύπαρξης στατιστικά σημαντικών διαφορών μεταξύ δύο ανεξάρτητων δειγμάτων, που ακολουθούν την κανονική κατανομή (t-test για ανεξάρτητα δείγματα) Όταν απαιτείται ο έλεγχος της ύπαρξης στατιστικά σημαντικών
Έλεγχος ύπαρξης στατιστικά σημαντικών διαφορών μεταξύ δύο ανεξάρτητων δειγμάτων, που ακολουθούν την κανονική κατανομή (t-test για ανεξάρτητα δείγματα) Όταν απαιτείται ο έλεγχος της ύπαρξης στατιστικά σημαντικών
Μενύχτα, Πιπερίγκου, Σαββάτης. ΒΙΟΣΤΑΤΙΣΤΙΚΗ Εργαστήριο 6 ο
 Παράδειγμα 1 Ο παρακάτω πίνακας δίνει τις πωλήσεις (ζήτηση) ενός προϊόντος Υ (σε κιλά) από το delicatessen μιας περιοχής και τις αντίστοιχες τιμές Χ του προϊόντος (σε ευρώ ανά κιλό) για μια ορισμένη χρονική
Παράδειγμα 1 Ο παρακάτω πίνακας δίνει τις πωλήσεις (ζήτηση) ενός προϊόντος Υ (σε κιλά) από το delicatessen μιας περιοχής και τις αντίστοιχες τιμές Χ του προϊόντος (σε ευρώ ανά κιλό) για μια ορισμένη χρονική
Equations. BSU Math 275 sec 002,003 Fall 2018 (Ultman) Final Exam Notes 1. du dv. FTLI : f (B) f (A) = f dr. F dr = Green s Theorem : y da
 BSU Math 275 sec 002,003 Fall 2018 (Ultman) Final Exam Notes 1 Equations r(t) = x(t) î + y(t) ĵ + z(t) k r = r (t) t s = r = r (t) t r(u, v) = x(u, v) î + y(u, v) ĵ + z(u, v) k S = ( ( ) r r u r v = u
BSU Math 275 sec 002,003 Fall 2018 (Ultman) Final Exam Notes 1 Equations r(t) = x(t) î + y(t) ĵ + z(t) k r = r (t) t s = r = r (t) t r(u, v) = x(u, v) î + y(u, v) ĵ + z(u, v) k S = ( ( ) r r u r v = u
Αν οι προϋποθέσεις αυτές δεν ισχύουν, τότε ανατρέχουµε σε µη παραµετρικό τεστ.
 ΣΤ. ΑΝΑΛΥΣΗ ΙΑΣΠΟΡΑΣ (ANALYSIS OF VARIANCE - ANOVA) ΣΤ 1. Ανάλυση ιασποράς κατά µία κατεύθυνση. Όπως έχουµε δει στη παράγραφο Β 2, όταν θέλουµε να ελέγξουµε, αν η µέση τιµή µιας ποσοτικής µεταβλητής διαφέρει
ΣΤ. ΑΝΑΛΥΣΗ ΙΑΣΠΟΡΑΣ (ANALYSIS OF VARIANCE - ANOVA) ΣΤ 1. Ανάλυση ιασποράς κατά µία κατεύθυνση. Όπως έχουµε δει στη παράγραφο Β 2, όταν θέλουµε να ελέγξουµε, αν η µέση τιµή µιας ποσοτικής µεταβλητής διαφέρει
Repeated measures Επαναληπτικές μετρήσεις
 ΠΡΟΒΛΗΜΑ Στο αρχείο δεδομένων diavitis.sav καταγράφεται η ποσότητα γλυκόζης στο αίμα 10 ασθενών στην αρχή της χορήγησης μιας θεραπείας, μετά από ένα μήνα και μετά από δύο μήνες. Μελετήστε την επίδραση
ΠΡΟΒΛΗΜΑ Στο αρχείο δεδομένων diavitis.sav καταγράφεται η ποσότητα γλυκόζης στο αίμα 10 ασθενών στην αρχή της χορήγησης μιας θεραπείας, μετά από ένα μήνα και μετά από δύο μήνες. Μελετήστε την επίδραση
ES440/ES911: CFD. Chapter 5. Solution of Linear Equation Systems
 ES440/ES911: CFD Chapter 5. Solution of Linear Equation Systems Dr Yongmann M. Chung http://www.eng.warwick.ac.uk/staff/ymc/es440.html Y.M.Chung@warwick.ac.uk School of Engineering & Centre for Scientific
ES440/ES911: CFD Chapter 5. Solution of Linear Equation Systems Dr Yongmann M. Chung http://www.eng.warwick.ac.uk/staff/ymc/es440.html Y.M.Chung@warwick.ac.uk School of Engineering & Centre for Scientific
ΠΕΡΙΕΧΟΜΕΝΑ 1 ΕΙΣΑΓΩΓΗ ΤΟ PASW ΜΕ ΜΙΑ ΜΑΤΙΑ ΠΕΡΙΓΡΑΦΙΚΗ ΣΤΑΤΙΣΤΙΚΗ: Η ΜΕΣΗ ΤΙΜΗ ΚΑΙ Η ΔΙΑΜΕΣΟΣ... 29
 ΠΕΡΙΕΧΟΜΕΝΑ 1 ΕΙΣΑΓΩΓΗ... 1 Μεταβλητές...5 Πληθυσμός, δείγμα...7 Το ευρύτερο γραμμικό μοντέλο...8 Αναφορές στη βιβλιογραφία... 11 2 ΤΟ PASW ΜΕ ΜΙΑ ΜΑΤΙΑ... 13 Περίληψη... 13 Εισαγωγή... 13 Με μια ματιά...
ΠΕΡΙΕΧΟΜΕΝΑ 1 ΕΙΣΑΓΩΓΗ... 1 Μεταβλητές...5 Πληθυσμός, δείγμα...7 Το ευρύτερο γραμμικό μοντέλο...8 Αναφορές στη βιβλιογραφία... 11 2 ΤΟ PASW ΜΕ ΜΙΑ ΜΑΤΙΑ... 13 Περίληψη... 13 Εισαγωγή... 13 Με μια ματιά...
CHAPTER 12: PERIMETER, AREA, CIRCUMFERENCE, AND 12.1 INTRODUCTION TO GEOMETRIC 12.2 PERIMETER: SQUARES, RECTANGLES,
 CHAPTER : PERIMETER, AREA, CIRCUMFERENCE, AND SIGNED FRACTIONS. INTRODUCTION TO GEOMETRIC MEASUREMENTS p. -3. PERIMETER: SQUARES, RECTANGLES, TRIANGLES p. 4-5.3 AREA: SQUARES, RECTANGLES, TRIANGLES p.
CHAPTER : PERIMETER, AREA, CIRCUMFERENCE, AND SIGNED FRACTIONS. INTRODUCTION TO GEOMETRIC MEASUREMENTS p. -3. PERIMETER: SQUARES, RECTANGLES, TRIANGLES p. 4-5.3 AREA: SQUARES, RECTANGLES, TRIANGLES p.
Λυμένες Ασκήσεις για το μάθημα:
 Λυμένες Ασκήσεις για το μάθημα: ΣΤΑΤΙΣΤΙΚΗ ΑΝΑΛΥΣΗ ΜΕ ΧΡΗΣΗ Η/Υ ΚΩΝΣΤΑΝΤΙΝΟΣ ΖΑΦΕΙΡΟΠΟΥΛΟΣ Τμήμα: ΔΙΕΘΝΩΝ ΚΑΙ ΕΥΡΩΠΑΪΚΩΝ ΣΠΟΥΔΩΝ Άδειες Χρήσης Το παρόν εκπαιδευτικό υλικό υπόκειται σε άδειες χρήσης Creative
Λυμένες Ασκήσεις για το μάθημα: ΣΤΑΤΙΣΤΙΚΗ ΑΝΑΛΥΣΗ ΜΕ ΧΡΗΣΗ Η/Υ ΚΩΝΣΤΑΝΤΙΝΟΣ ΖΑΦΕΙΡΟΠΟΥΛΟΣ Τμήμα: ΔΙΕΘΝΩΝ ΚΑΙ ΕΥΡΩΠΑΪΚΩΝ ΣΠΟΥΔΩΝ Άδειες Χρήσης Το παρόν εκπαιδευτικό υλικό υπόκειται σε άδειες χρήσης Creative
HISTOGRAMS AND PERCENTILES What is the 25 th percentile of a histogram? What is the 50 th percentile for the cigarette histogram?
 HISTOGRAMS AND PERCENTILES What is the 25 th percentile of a histogram? The point on the horizontal axis such that of the area under the histogram lies to the left of that point (and to the right) What
HISTOGRAMS AND PERCENTILES What is the 25 th percentile of a histogram? The point on the horizontal axis such that of the area under the histogram lies to the left of that point (and to the right) What
Approximation of distance between locations on earth given by latitude and longitude
 Approximation of distance between locations on earth given by latitude and longitude Jan Behrens 2012-12-31 In this paper we shall provide a method to approximate distances between two points on earth
Approximation of distance between locations on earth given by latitude and longitude Jan Behrens 2012-12-31 In this paper we shall provide a method to approximate distances between two points on earth
Congruence Classes of Invertible Matrices of Order 3 over F 2
 International Journal of Algebra, Vol. 8, 24, no. 5, 239-246 HIKARI Ltd, www.m-hikari.com http://dx.doi.org/.2988/ija.24.422 Congruence Classes of Invertible Matrices of Order 3 over F 2 Ligong An and
International Journal of Algebra, Vol. 8, 24, no. 5, 239-246 HIKARI Ltd, www.m-hikari.com http://dx.doi.org/.2988/ija.24.422 Congruence Classes of Invertible Matrices of Order 3 over F 2 Ligong An and
Reservoir modeling. Reservoir modelling Linear reservoirs. The linear reservoir, no input. Starting up reservoir modeling
 Reservoir modeling Reservoir modelling Linear reservoirs Paul Torfs Basic equaion for one reservoir:) change in sorage = sum of inflows minus ouflows = Q in,n Q ou,n n n jus an ordinary differenial equaion
Reservoir modeling Reservoir modelling Linear reservoirs Paul Torfs Basic equaion for one reservoir:) change in sorage = sum of inflows minus ouflows = Q in,n Q ou,n n n jus an ordinary differenial equaion
ΚΟΙΝΩΝΙΟΒΙΟΛΟΓΙΑ, ΝΕΥΡΟΕΠΙΣΤΗΜΕΣ ΚΑΙ ΕΚΠΑΙΔΕΥΣΗ
 A εξάμηνο 2009-2010 ΠΡΟΓΡΑΜΜΑ ΜΕΤΑΠΤΥΧΙΑΚΩΝ ΣΠΟΥΔΩΝ ΚΟΙΝΩΝΙΟΒΙΟΛΟΓΙΑ, ΝΕΥΡΟΕΠΙΣΤΗΜΕΣ ΚΑΙ ΕΚΠΑΙΔΕΥΣΗ Μεθοδολογία Έρευνας και Στατιστική ΑΝΤΩΝΙΟΣ ΧΡ. ΜΠΟΥΡΑΣ Χειμερινό Εξάμηνο 2009-2010 Ποιοτικές και Ποσοτικές
A εξάμηνο 2009-2010 ΠΡΟΓΡΑΜΜΑ ΜΕΤΑΠΤΥΧΙΑΚΩΝ ΣΠΟΥΔΩΝ ΚΟΙΝΩΝΙΟΒΙΟΛΟΓΙΑ, ΝΕΥΡΟΕΠΙΣΤΗΜΕΣ ΚΑΙ ΕΚΠΑΙΔΕΥΣΗ Μεθοδολογία Έρευνας και Στατιστική ΑΝΤΩΝΙΟΣ ΧΡ. ΜΠΟΥΡΑΣ Χειμερινό Εξάμηνο 2009-2010 Ποιοτικές και Ποσοτικές
ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ. 8. Ανάλυση διασποράς (ANOVA)
 ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ 8. Ανάλυση διασποράς (ANOVA) Γενικά Επέκταση της σύγκρισης µέσων τιµών µεταβλητής ανάµεσα σε 2 δείγµατα (οµάδες ήστάθµες): Σύγκριση πολλών δειγµάτων (K>2) µαζί Σχέση ανάµεσα σε µια ποσοτική
ΑΝΑΛΥΣΗ Ε ΟΜΕΝΩΝ 8. Ανάλυση διασποράς (ANOVA) Γενικά Επέκταση της σύγκρισης µέσων τιµών µεταβλητής ανάµεσα σε 2 δείγµατα (οµάδες ήστάθµες): Σύγκριση πολλών δειγµάτων (K>2) µαζί Σχέση ανάµεσα σε µια ποσοτική
Solutions to Exercise Sheet 5
 Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
Matrices and Determinants
 Matrices and Determinants SUBJECTIVE PROBLEMS: Q 1. For what value of k do the following system of equations possess a non-trivial (i.e., not all zero) solution over the set of rationals Q? x + ky + 3z
Matrices and Determinants SUBJECTIVE PROBLEMS: Q 1. For what value of k do the following system of equations possess a non-trivial (i.e., not all zero) solution over the set of rationals Q? x + ky + 3z
Homework 3 Solutions
 Homework 3 Solutions Igor Yanovsky (Math 151A TA) Problem 1: Compute the absolute error and relative error in approximations of p by p. (Use calculator!) a) p π, p 22/7; b) p π, p 3.141. Solution: For
Homework 3 Solutions Igor Yanovsky (Math 151A TA) Problem 1: Compute the absolute error and relative error in approximations of p by p. (Use calculator!) a) p π, p 22/7; b) p π, p 3.141. Solution: For
Fourier Series. Fourier Series
 ECE 37 Z. Aliyazicioglu Elecrical & Compuer Egieerig Dep. Cal Poly Pomoa Periodic sigal is a fucio ha repeas iself every secods. x() x( ± ) : period of a fucio, : ieger,,3, x() 3 x() x() Periodic sigal
ECE 37 Z. Aliyazicioglu Elecrical & Compuer Egieerig Dep. Cal Poly Pomoa Periodic sigal is a fucio ha repeas iself every secods. x() x( ± ) : period of a fucio, : ieger,,3, x() 3 x() x() Periodic sigal
Generalized additive models in R
 www.nr.no Generalized additive models in R Magne Aldrin, Norwegian Computing Center and the University of Oslo Sharp workshop, Copenhagen, October 2012 Generalized Linear Models - GLM y Distributed with
www.nr.no Generalized additive models in R Magne Aldrin, Norwegian Computing Center and the University of Oslo Sharp workshop, Copenhagen, October 2012 Generalized Linear Models - GLM y Distributed with
ΚΥΠΡΙΑΚΗ ΕΤΑΙΡΕΙΑ ΠΛΗΡΟΦΟΡΙΚΗΣ CYPRUS COMPUTER SOCIETY ΠΑΓΚΥΠΡΙΟΣ ΜΑΘΗΤΙΚΟΣ ΔΙΑΓΩΝΙΣΜΟΣ ΠΛΗΡΟΦΟΡΙΚΗΣ 24/3/2007
 Οδηγίες: Να απαντηθούν όλες οι ερωτήσεις. Όλοι οι αριθμοί που αναφέρονται σε όλα τα ερωτήματα μικρότεροι του 10000 εκτός αν ορίζεται διαφορετικά στη διατύπωση του προβλήματος. Αν κάπου κάνετε κάποιες υποθέσεις
Οδηγίες: Να απαντηθούν όλες οι ερωτήσεις. Όλοι οι αριθμοί που αναφέρονται σε όλα τα ερωτήματα μικρότεροι του 10000 εκτός αν ορίζεται διαφορετικά στη διατύπωση του προβλήματος. Αν κάπου κάνετε κάποιες υποθέσεις
Second Order RLC Filters
 ECEN 60 Circuits/Electronics Spring 007-0-07 P. Mathys Second Order RLC Filters RLC Lowpass Filter A passive RLC lowpass filter (LPF) circuit is shown in the following schematic. R L C v O (t) Using phasor
ECEN 60 Circuits/Electronics Spring 007-0-07 P. Mathys Second Order RLC Filters RLC Lowpass Filter A passive RLC lowpass filter (LPF) circuit is shown in the following schematic. R L C v O (t) Using phasor
