Paul Langacker. The Standard Model and Beyond, Second Edition Answers to Selected Problems
|
|
|
- Τάνις Κορομηλάς
- 6 χρόνια πριν
- Προβολές:
Transcript
1 Paul Langacker The Standard Model and Beyond, Second Edition Answers to Selected Problems
2
3 Contents Chapter Review of Perturbative Field Theory 1.1 PROBLEMS 1 Chapter 3 Lie Groups, Lie Algebras, and Symmetries PROBLEMS 5 Chapter 4 Gauge Theories PROBLEMS 9 Chapter 5 The Strong Interactions and QCD PROBLEMS 11 Chapter 6 Collider Physics PROBLEMS 15 Chapter 7 The Weak Interactions PROBLEMS 17 Chapter 8 The Standard Electroweak Theory PROBLEMS 19 Chapter 9 Neutrino Mass and Mixing PROBLEMS 5 Chapter 10 Beyond the Standard Model PROBLEMS 7 i
4
5 C H A P T E R Review of Perturbative Field Theory.1 PROBLEMS Problem.: 0.04 dσ d cos θ (fm ) cos θ σ (fm ) s (GeV) Left: dσ/d cos θ in units of fm as a function of cos θ for s = 5m, 6m, and 7m (from upper to lower). Right: σ(s) in units of fm as a function of s Problem.5: [ ] M fi = ( iκ) i i s m + 3 u m 3 dσ d cos θ = M fi 3πs, u = p (1 + cos θ), p = s 4m 1 / Problem.6: M fi = 4iσ 4 Problem.7: dγ d cos θ = αm A 8 (1 4m M A ) 3/ cos θ, Γ = αm A 1 (1 4m M A ) 3/ 1
6 The Standard Model and Beyond Problem.8: (a) ( i M fi = ( ig) s µ + i ) t µ, s 4m t = p (1 cos θ), p =, E = (b,c) dσ d cos θ = M fi +1 3πs, σ = 1 dσ d cos θ. d cos θ s, dσ d cos θ (fm ) π + π - π + π - x=4.0 x=4. x=4.4 σ (fm ) π + π - π + π cos θ x Left: dσ/d cos θ in units of fm for x = 4, 4., and 4.4. Right: σ in units of fm vs x. ( ) Problem.15: For example, P L u(+) m φ+ E E, so the rates for the wrong he- 0 licity are suppressed by m /4E. Problem.18: (a) with d σ d cos θ = 1 3πs M [ ( M = h 4 1 t µ ) ( 4m t ) ( + 1 u µ ) ( 4m u ) 1 ( ) ( ) 1 1 [(4m t µ u µ u ) ( + 4m t ) ( s 4m ) ]], where E = s s 4m, k =, t = k (1 cos θ), u = k (1 + cos θ). (b) d σ d cos θ = 3h4 3πs, σ = d cos θ d σ d cos θ = 3h4 3πs.
7 Review of Perturbative Field Theory 3 Problem.0: d σ p [ = 4πα s + (E + p cos θ) d cos θ st m µ(1 cos θ) ] Problem.1: d σ d cos θ = πα s ( 3 + cos ) θ, 1 cos θ which displays the singularity from the t-channel pole in the forward direction. Problem.: d σ d cos θ = πα s = πα s [ (1 + cos 4 θ ) sin 4 θ [ 16 sin 4 θ 8 sin θ (1 + sin4 θ ) + cos 4 θ ]. ] sin θ cos θ Problem.3: d σ d cos θ = πα βf 3 sin θ, 4s σ = πα β 3 f 3s Problem.4: d σ d cos θ = (Zα) π(1 β cos θ β p sin 4 θ ) β 1 Z α π β p sin 4 θ Problem.5: p f Γ = 8πMZ M m 0 M Z ( g 1π V + ga ). Problem.6: dγ d cos θ = p f [( gs + g P ) E p + ( g S g P ) m p 8πm Λ + Re (g P gs) p f cos θ]. Problem.7: σ(s) = 1π(s/M V ) Γ aā Γb b (s MV ) + MV, Γb b = g b M V Γ V 1π. Problem.9: M(, ) = M(+, +) = 8πα i ( 1 β π + 1 ) cos θ 1 cos θ.
8 4 The Standard Model and Beyond Problem.30: (a) eφ s (mã0( q ) ( p 1 + p ) ) A( q ) σ B( q ) [1 + F (0)] φ s1. (b) d e = ieg (0) m σ. Problem.31: τ 1 = 1 4 δ α m e, δ < Problem.3: p() H p(1) = p() d 3 x ej µ Q A µ p(1) [ = m p e φ G E (Q )Ã0( q ) G M (Q ) σ ] B( q ) m p φ 1 Problem.33: (a) (b) (c) Ṽ ( q ) = λ, V ( r ) = λδ 3 ( r ). Ṽ ( q ) = g 1g q + m, V (r) = g 1g φ 4π V (r) = + g 1g 4π (d) Sign change for vector, not for scalar. (e) V ( r ) = g πm 3 π 16πm p e M V r r. e m φr ( σ p x ) ( σ n x ) ( e x x r ).. Problem.34: Γ = e m 3 µ 8π ( A + B ).
9 C H A P T E R 3 Lie Groups, Lie Algebras, and Symmetries 3.1 PROBLEMS Problem 3.4: cos γ = cos α cos β sin α sin β ˆα ˆβ sin γ ˆγ = sin α cos β ˆα + cos α sin β ˆβ + sin α sin β ˆα ˆβ. Problem 3.13: π + p M π + p = M 3/, π p M π p = 1 3 M 3/ + 3 M 1/ π 0 n M π p = 3 M 3/ 3 M 1/, π 0 n M π 0 n = 3 M 3/ M 1/ Problem 3.14: M(Σ + π 0 ( ) p) = M3/ M 1/ 3 M(Σ + π + n) = 1 3 M 3/ + 3 M 1/ M(Σ π n) = M 3/. Problem 3.16: σ A+ p A + p = σ A 0 p A 0 p = 1 4 σ A + n A 0 p = λ 8πs (E 1 + m p), E 1 = s + m p m A s 5
10 6 The Standard Model and Beyond Problem 3.19: g K+ nσ = g π + pn + 6g K+ pλ Problem 3.0: M p = M n M N = m m β + M Ξ 0 = M Ξ M Ξ = m m β M Σ ± = M Σ 0 M Σ = m 0 + m β 3 m α 3 m α M Λ = m 0 m β 3 Problem 3.: (b) g p /g n = 3/ (exp: 1.46) (c) g p / = 3 (exp:.79); g n / = (exp: 1.91) Problem 3.3: ( ) [ gf g d M π0 p = ( ie)ū γ 5 i( k 1+ p 1 + m) (k 1 + p 1 ) m ɛ+ ɛ i( p ] 1 k + m) (p 1 k ) m γ5 u 1 ( ) [ M K + Σ 0 = gf + g d ( ie)ū γ 5 i( k ] 1+ p 1 + m) (k 1 + p 1 ) m ɛ + k i ɛ (k k 1 ) µ γ5 u 1 Problem 3.5: (a) (b) ( L i A )ab;cd = Li acδ bd + L i bdδ ac L i adδ bc L i bcδ ad, T (L A ) = m. ( L i S )ab;cd = c ab c cb [L i acδ bd + L i bdδ ac + L i adδ bc + L i bcδ ad ], T (L S ) = m +. For m =, ψ S 11, ψ S 1 and ψ S correspond to the J = 1 angular momentum representation with J 3 = +1, 0, and 1, respectively. For example, ( L 3 S )11;11 = 1 4 [4] = 1, ( L 3 S )1;1 = 1 4 [1 1] = 0, ( ) L 1 S 11;1 = 1 1 [ ] = 1. Problem 3.6: JLµ i = ψ ( ) L γ µ L i nψ L + itr φ L i n µ φ JRµ i = ψ ( R γ µ L i nψ R + itr φl i n µ φ ), and L i n I for the U(1) currents.
11 Lie Groups, Lie Algebras, and Symmetries 7 Problem 3.8: a = ν = µ /λ, b = µ / ( ) H(x) = µ4 µ x λ sech4, + H(x)dx = µ 3 3 λ Problem 3.30: (a) (b) M I = i ( mψ ν ) ū4 [ [ ( M = iλ 1 + 3m 1 1 t m + m 1 1 s + 1 )] u ] m η 1 m ψ t m + γ 5 1 γ 5 + γ 5 1 γ 5 η p 1 + p m ψ p p 3 m ψ u Problem 3.3: ψ = 1 {[ a b + a + b e iφ m ] + [ a b + a + b e iφm ] γ 5} ψ γ 5 ψ m = c + d a b, tan φ m = d c Problem 3.33: λ > 0 : φ 1 = ν b µ /λ, φ = 0, m 1 = λν b, m = λ ν b. φ φ survives. λ = 0 is similar except there is a circle of degenerate minima. For λ < 0, require λ + λ > 0. φ 1 = φ = ν c µ /(λ + λ ), m 1 = λ ν c, m = (λ + λ )ν c. φ 1 φ survives. Problem 3.34: λ 1 > 0, λ 3 > 0, µ µ 1 0, (flat for µ = µ 1 ). Problem 3.35: (a) µ I + µ II A > 0. (c) Minimum for µ I µ II A > 0; saddle point for µ I µ II A < 0. (d,e) µ A = µ I + µ II = A sin γ, 1 M Z = λν = µ I µ II tan γ tan. γ 1 (f) ( µ M Re = Z cos γ + µ A sin γ (MZ + ) µ A ) sin γ cos γ (MZ + µ A ) sin γ cos γ M Z sin γ + µ. A cos γ
12 8 The Standard Model and Beyond Problem 3.36: (b) (c) µ > 0 : m 1 = m = µ, m ψ = 0 µ < 0 : m 1 = µ, m = 0, m ψ = hν/, with ν = µ /λ µ > 0 : m 1 = µ + 3λν, m = µ + λν, m ψ = hν, with ν a/µ µ < 0 : m 1 = µ + 3 a a, m =, m ψ = h ν 0 + ɛ, ν 0 ν 0 with ν = ν 0 + ɛ, ν 0 = µ /λ, ɛ = a µ (e) µ J µ = i m L ( ψ L C ψ T L ψ T LCψ L )
13 C H A P T E R 4 Gauge Theories 4.1 PROBLEMS Problem 4.1: A µ = ( 0, nˆθ ) ( ), A ds rg = πn g. Problem 4.11: (a) gγ µ /, gγ µ /, igc µνσ ( p 3, p 3 + p 4, p 4 ) (b) M = ig v ɛ 1 ɛ+ u 1 p 1 p 3 m ψ + ig 1 s M A v [ ɛ + p 3 ɛ + ɛ + ɛ ( p 4 p 3 ) ɛ p 4 ɛ +] u 1. (c) M ig M A v p 4 u 1 ig MA v p 4 u 1 = 0 Problem 4.1: (a) A ijk = d ijk. (b) A D ijk = md ijk. One can also show that A A ijk = (m 4)d ijk and A S ijk = (m + 4)d ijk for the antisymmetric (A) and symmetric (D) products of two fundamentals. 9
14
15 C H A P T E R 5 The Strong Interactions and QCD 5.1 PROBLEMS Problem 5.1: (b) b = 1, c = 1/ 3. (c) R = 4() for HN (QCD); Drell Yan 5/9 (1/3); anomaly coefficient = 4 for both. Problem 5.: M = 4 9 gs 4 [ (s m t c ) + (u m c) + m ct ] u m c = p(e + p cos θ), t = p (1 cos θ), E = s + m c s, p = s m c s Problem 5.3: (a) where (b,c) L φ =Tr [(D µ φ) D µ φ] µ Tr (φ φ) λ 1 ( Tr (φ φ) ) λ Tr (φ φφ φ) + q L hφq R + q R h φ q L, D µ φ = µ φ + ig L G µ L L φ ig R φ G µ R L. G i = sin δ G i L + cos δ G i R, G i A = cos δ G i L sin δ G i R, where tan δ g R /g L and M GA = g L + g R v φ. L q = g s q G L q gs cot δ q L GA L q L + g s tan δ q R GA L q R, where g s g L sin δ = g R cos δ; T i = T i L + T i R. Problem 5.4: σ = 8πα s 7s β Q ( 3 β Q σ = πα sβ 3 0 7s ) with β 0 = with β Q = 1 4m Q s 1 4m 0 s. 11
16 1 The Standard Model and Beyond Problem 5.5: F r=1 σ q0 q 0 q r q r β rel = n f 4πα s 7m 0 β i, σ q0 q 0 GG β rel = 8 7 παs m. 0 Problem 5.6: (a) M /gs 4 = 4 ( ( s + u m r m s + s t + u ) + m 4 r m s(s t + u) + ms) 4 9t (b) ( M 7m /gs 4 4 7m t 7m u + 4t tu + 4u ) = 4s (m t) (m u) ( 6m 8 3m 4 t 14m 4 tu 3m 4 u + m t 3 + 7m t u + 7m tu + m u 3 t 3 u tu 3) (d) Use the expressions for t and u in (.40) for m 1, = 0, m 3,4 = m t, and the cross section formula in (.57), integrating cos θ from 1 to σ t t _ (nb) qq tt GG t t s (TeV) Problem 5.8: (a) B = 7, α(m P ) 0.019; (b) B = 3, α(m P ) Problem 5.10: charmonium bottomonium exp model difference exp model difference 1S S % % 3S % 4S %
17 The Strong Interactions and QCD 13 for m c 1.7 GeV and m b 4.61 GeV. Problem 5.17: (b)tr ( M 1 M ) [ (, Tr M 1 M )][ ( )] ( ) Tr M a M b, Tr M 1 M M 1 M (c) Tr (M[ a M b ), ψ L ψ] R + ψ R ψ L (d) itr ˆn τm 1 M.
18
19 C H A P T E R 6 Collider Physics 6.1 PROBLEMS Problem 6.3: dlij/ds dy (pb) LHC (14 TeV), s = 1 TeV uu uu dlij/ds dy (pb) LHC (14 TeV), s = 1 TeV uu Gu GG y y Problem 6.4: Cross sections in pb: p p ( TeV) pp (8 TeV) pp (14 TeV) σ q q (pb) σ GG (pb) σ tot (pb) pp tt ( TeV) pp tt ( TeV) dσ /dy (pb) total qq GG dσ/dp T (pb/gev) total qq GG y p T (GeV) 15
20 16 The Standard Model and Beyond Problem 6.5: λ GG = π 8 Γ R dl GG 130 fb, M R dŝ λ uū = 4π 9 Γ R dl uū+ūu 79 fb, M R dŝ λ d d 51 fb. Problem 6.6: 1, 1, 3
21 C H A P T E R 7 The Weak Interactions 7.1 PROBLEMS Problem 7.: (a) 1ɛ (1 ɛ) (b) ɛ [ (3 ɛ) + 6(1 ɛ) ], ρ = 3 8 (c): ν µ : ɛ (3 ɛ), ν e : 1ɛ (1 ɛ). Problem 7.10: (a), (b), (c) in text. (d): α eν = g V g A. gv +3g A Problem 7.11: dγ(πν τ ) d cos θ = M 3πm τ Γ(πν τ ) = G F cos θ c fπm 3 τ. 16π = G F cos θ c fπm 3 τ (1 + cos θ) 3π 17
22
23 C H A P T E R 8 The Standard Electroweak Theory 8.1 PROBLEMS Problem 8.4: (a) V (φ, σ) = µ σσ σ + µ φφ φ + λ σ ( σ σ ) + λφ ( φ φ ) + λφσ σ σφ φ + κ φσ σφ φ + κ φσ σ φ φ. (c) M A cos θ W g ν σ (d) Γ = p ( ) f 6πMZ g sin θ W MA + E A MA, which survives for M A 0 because of the longitudinal mode. Problem 8.5: (b) L lh = 0. L l(w,a,z) = g ( Nγ µ E W µ + + Ēγµ N Wµ ) + e Ēγ µ EA µ g [ 1 cos θ W Nγ µ N + ( 1 ) ] Ēγµ + sin θ W E Z µ. Problem 8.6: A L MA R = diag( ) for i i i A L = i i i i i A R = i i i diag(e +3.14i e +0.14i e 0.67i ) Problem 8.7: m 1 = m = 3c, ψ L = ψ 0 L = ( ψ 0 1L ψ 0 L ), ψ R = i ( ψ 0 R ψ 0 1R ) 19
24 0 The Standard Model and Beyond Problem 8.8: (a) m 1 x, m B, A R I, ( ) A 1 y L = B y. B 1 (b) J µ Z = ē Lγ µ e L y B (ēl γ µ E L + ĒLγ µ e L ) ( y b ) ĒL γ µ E L + sin θ W (ēγ µ e + Ēγµ E ). Problem 8.9: M = g4 M Z (g V + g A ) cos 4 θ W 1 (q M Z ) d σ d cos θ = 1 3πs [ p 1 p 3 + p ] 1 p 4 p 3 p 4 MZ k 3 k 1 M. k 1 = s M H s, E = s + M H s, k 3 = s M Z s, E 4 = s + M Z s q = (p 3 p 1 ) = p 1 p 3 = k 1 k 3 (1 cos θ) p 1 p 4 = k 1 (E 4 + k 3 cos θ), p 3 p 4 = s M Z. Problem 8.10: L = Γ D κσ q L φd R /M D + h.c.. Problem 8.13: (a) d σ dz = s [( ɛll + ɛ RR ) (1 + z) + ( ɛ LR + ɛ RL ) (1 z) ] 384π z z for q r q r µ µ +. (b) For y > 0 d σ dydẑ =λ Z ɛ AB = Q re g s ZD(s)ɛ A (µ)ɛ B (r). [ ( Lr r G r N (1 + ẑ) + G r F (1 ẑ) ) r +L rr ( G r N (1 ẑ) + G r F (1 + ẑ) )], (ẑ ẑ for y < 0), where gz 4 λ Z, L r r dl q r q r, 384M Z Γ Z dŝdy ŝ=m L rr dl q rq r dŝdy ŝ=m Z Z G r N = ɛ L (µ) ɛ L (r) + ɛ R (µ) ɛ R (r), G r F = ɛ L (µ) ɛ R (r) + ɛ R (µ) ɛ L (r).
25 The Standard Electroweak Theory 1 (c) A F B (y) = 3 r L r r L rr (G r N Gr F ) 4 r (L r r + L rr ) (G r N + Gr F ) A similar expression holds for A F B (y 1, y ) except the numerator and denorminator are integrated ( y y y 1 dy, or y 1 + ) y 1 y dy. For more detail, see (Han et al., 013). (d) A F B (0, ln( s/m Z )) A FB (pp μ - μ + ), s = 14 TeV, s M Z 0.08 AFB (y) Problem 8.14: M νe [ ig = ɛ 3µɛ 4ν v 3 γ ν (1 γ 5 ) ) ( i M γ = ɛ 3µɛ 4ν v 3 [ieγ α ]u 1 s [ M Z = ɛ 3µɛ ig 4ν v 3 y ] ( i( p1 p 3 ) t ) [ ] ig γ µ (1 γ 5 ) u 1 ie C µαν ( p 3, p 1 + p, p 4 ) γ ( α 1 cos θ + sin θ W 1 )] γ5 u 1 W ( ) i e s MZ i C µαν ( p 3, p 1 + p, p 4 ) tan θ W
26 The Standard Model and Beyond 40 e + e - W + W - 30 σww (pb) only ν e ν e, γ ν e, γ, Z s (GeV) Problem 8.15: (a) dγ d cos θ = ĝ Eb [ ] (1 + cos θ) + m t 4πm t MW (1 cos θ) (c) m t F 0 = MW + m t 0.70, F = 1 F 0, F + = 0 Problem 8.0: (a) σ GGF pp H = π 8 σ W W F q rq s q rq sh(ŝ) = Γ H B(H GG) dl GG M H dŝ [( α3 g 16MW 1 + M H ) ln ŝ ŝ M H M H 11 pb + M H ] ŝ σ W W F pp H = q rq s dŝ ŝ dl qrq s dŝ ŝ σ W W F q rq s q rq sh(ŝ) 1.5 pb, σ ZZF pp H 0.5 pb σ qr q s W ± H(ŝ) = V rs πα g 36 p f p i 3M W + p f (ŝ M W ) (b) σ pp W ± H = q r q s dŝ ŝ dl qr q s dŝ ŝ σ qr q s W ± H(ŝ) 0.5 pb, σ pp ZH 0.3 pb. σ NNLO (pb) σ LO (pb) N γγ N 4l GGF VBF W H ZH total
27 The Standard Electroweak Theory 3 Problem 8.1: Γ(H GG) increases by a factor 9, while Γ increases by 1.8. B(H ZZ ) B(H ZZ 1 ) SM , B(H γγ).8/ , B(H γγ) SM 1.7 The rates σ GG B change by factors of and for ZZ and γγ, respectively. Problem 8.: (b) Define V (H + ν) c n= c nh n. Then MH = c = λν + ρν 4, c 3 = M ) H (1 + 8ρν4, c 4 = M ) H (1 ν 8ν + 16ρν4 c 5 = ρν, c 6 = ρ 6. 3M H (c) κ 1 4σν and M H / λν (1 4σν ). Interactions terms: M H σ ( νh + H ) ( µ H) M H H M H ( 1 σν ) H 3 M ( H 1 4σν ) ν 8ν H 4. Problem 8.3: M = i G F MH s s MH, σ = M 16πs Problem 8.4: M = 4ĝ ( m t M W ) mt E b (1 cos θ)
28
29 C H A P T E R 9 Neutrino Mass and Mixing 9.1 PROBLEMS Problem 9.: m 1 = ɛ, ν 1L = ν µl ν τl m,3 = + ɛ (, ν,3l = ɛ ) ν el + ν µl + ν τl, Problem 9.3: m T γ µ (1 γ 5 )γ ν γ µ[ (1 γ 5 )m T + ɛ k ] γ ν + O(ɛ ) Problem 9.5: Dirac: σβ rel = G [ F π m ν ɛ L + ɛ ] R. Majorana: σβ rel = 8β 3 G [ F π m ν ɛ L + ɛ ] R. 5
30
31 C H A P T E R 10 Beyond the Standard Model 10.1 PROBLEMS Problem 10.1: (a) (b) n AF = 11C (G) = 11m n fp = 17C (G) 5C (G) + 3C (L m ) = 34m3 13m 3 < n AF α = 4πˆb 1 (m, n f ) ˆb (m, n f ) = 4π m(11m n f ) 34m 3 13m n f + 3n f 3.5 Running Coupling (m=3) g(t) n f =1, α g (M P )=0.6 n f =13, α g (M P )=0.4 n f =14, α g (M P )= t=ln Q/M Z (c) α < α c for n f > n c. α = α c for n c = 5 50m 3 33m 5m. 3 7
32 8 The Standard Model and Beyond Problem 10.3: For infinitesimal transformation M σ 0 M σ 0 β i σ i, M σ i M σ i ɛ ijk ω j σ k β i σ 0 Problem 10.4: ψ 1M ψ M = ξ 1 ξ + ξ 1 ξ, ψ1m γ 5 ψ M = ξ 1 ξ ξ 1 ξ ψ 1M γ µ ψ M = ξ 1 σ µ ξ + ξ 1 σ µ ξ, ψ1m γ µ γ 5 ψ M = ξ 1 σ µ ξ ξ 1 σ µ ξ ψ 1M σ µν ψ M = ξ 1 s µν ξ + ξ 1 s µν ξ Problem 10.10: σ(ah) = cos (β α) g4 Z kah 3 96π k i σ(zh) = sin (β α) g4 Z kzh 3 96π k i ɛ L + ɛ R (s M Z ) ɛ L + ɛ R (s MZ ) [ 1 + 3M Z k Zh ] Problem 10.13:
33 Beyond the Standard Model 9 σ(pp q G ), s = 14 TeV 1 σ(pb) m (GeV) m. TeV M Z g Problem 10.14: Typeset by (a) FoilTEX θ = Q φ g 1. M 1 Z (b) Γ Z w + w = Γ Z zh = gq M Z φ 48π. ( Problem 10.15: B 3 W h0 d h0 u S Z ) basis: m B 0 g ν d g ν u 0 0 gν 0 m d W gνu 0 0 g ν d gν d 0 λ S s λ S ν u g Q d ν d g ν u gνu λ S s 0 λ S ν d g Q u ν u 0 0 λ S ν u λ S ν d 0 g Q S s 0 0 g Q d ν d g Q u ν u g Q S s m Z Decoupling limit: m B 0 g ν d g ν u 0 0 gν 0 m d W gνu 0 0 g ν d gν d 0 µ eff 0 0 g ν u, gνu µ eff g Q S s g Q S s 0 where µ eff = λ S s. Vector, Dirac fermion, and scalar masses are all g Q S s
34 30 The Standard Model and Beyond Problem 10.17: ζ for ω = 0; ζ 0.0 for ω = π/ (1 + ζ cos ω) (6) Problem 10.18: V = 15 4 µ ν Φ (15a + 7b)ν4 Φ
Homework 8 Model Solution Section
 MATH 004 Homework Solution Homework 8 Model Solution Section 14.5 14.6. 14.5. Use the Chain Rule to find dz where z cosx + 4y), x 5t 4, y 1 t. dz dx + dy y sinx + 4y)0t + 4) sinx + 4y) 1t ) 0t + 4t ) sinx
MATH 004 Homework Solution Homework 8 Model Solution Section 14.5 14.6. 14.5. Use the Chain Rule to find dz where z cosx + 4y), x 5t 4, y 1 t. dz dx + dy y sinx + 4y)0t + 4) sinx + 4y) 1t ) 0t + 4t ) sinx
CRASH COURSE IN PRECALCULUS
 CRASH COURSE IN PRECALCULUS Shiah-Sen Wang The graphs are prepared by Chien-Lun Lai Based on : Precalculus: Mathematics for Calculus by J. Stuwart, L. Redin & S. Watson, 6th edition, 01, Brooks/Cole Chapter
CRASH COURSE IN PRECALCULUS Shiah-Sen Wang The graphs are prepared by Chien-Lun Lai Based on : Precalculus: Mathematics for Calculus by J. Stuwart, L. Redin & S. Watson, 6th edition, 01, Brooks/Cole Chapter
6.1. Dirac Equation. Hamiltonian. Dirac Eq.
 6.1. Dirac Equation Ref: M.Kaku, Quantum Field Theory, Oxford Univ Press (1993) η μν = η μν = diag(1, -1, -1, -1) p 0 = p 0 p = p i = -p i p μ p μ = p 0 p 0 + p i p i = E c 2 - p 2 = (m c) 2 H = c p 2
6.1. Dirac Equation Ref: M.Kaku, Quantum Field Theory, Oxford Univ Press (1993) η μν = η μν = diag(1, -1, -1, -1) p 0 = p 0 p = p i = -p i p μ p μ = p 0 p 0 + p i p i = E c 2 - p 2 = (m c) 2 H = c p 2
3.4 SUM AND DIFFERENCE FORMULAS. NOTE: cos(α+β) cos α + cos β cos(α-β) cos α -cos β
 3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
3.4 SUM AND DIFFERENCE FORMULAS Page Theorem cos(αβ cos α cos β -sin α cos(α-β cos α cos β sin α NOTE: cos(αβ cos α cos β cos(α-β cos α -cos β Proof of cos(α-β cos α cos β sin α Let s use a unit circle
The Standard Model. Antonio Pich. IFIC, CSIC Univ. Valencia
 http://arxiv.org/pd/0705.464 The Standard Mode Antonio Pich IFIC, CSIC Univ. Vaencia Gauge Invariance: QED, QCD Eectroweak Uniication: SU() Symmetry Breaking: Higgs Mechanism Eectroweak Phenomenoogy Favour
http://arxiv.org/pd/0705.464 The Standard Mode Antonio Pich IFIC, CSIC Univ. Vaencia Gauge Invariance: QED, QCD Eectroweak Uniication: SU() Symmetry Breaking: Higgs Mechanism Eectroweak Phenomenoogy Favour
Mock Exam 7. 1 Hong Kong Educational Publishing Company. Section A 1. Reference: HKDSE Math M Q2 (a) (1 + kx) n 1M + 1A = (1) =
 Mock Eam 7 Mock Eam 7 Section A. Reference: HKDSE Math M 0 Q (a) ( + k) n nn ( )( k) + nk ( ) + + nn ( ) k + nk + + + A nk... () nn ( ) k... () From (), k...() n Substituting () into (), nn ( ) n 76n 76n
Mock Eam 7 Mock Eam 7 Section A. Reference: HKDSE Math M 0 Q (a) ( + k) n nn ( )( k) + nk ( ) + + nn ( ) k + nk + + + A nk... () nn ( ) k... () From (), k...() n Substituting () into (), nn ( ) n 76n 76n
ECE Spring Prof. David R. Jackson ECE Dept. Notes 2
 ECE 634 Spring 6 Prof. David R. Jackson ECE Dept. Notes Fields in a Source-Free Region Example: Radiation from an aperture y PEC E t x Aperture Assume the following choice of vector potentials: A F = =
ECE 634 Spring 6 Prof. David R. Jackson ECE Dept. Notes Fields in a Source-Free Region Example: Radiation from an aperture y PEC E t x Aperture Assume the following choice of vector potentials: A F = =
Phys460.nb Solution for the t-dependent Schrodinger s equation How did we find the solution? (not required)
 Phys460.nb 81 ψ n (t) is still the (same) eigenstate of H But for tdependent H. The answer is NO. 5.5.5. Solution for the tdependent Schrodinger s equation If we assume that at time t 0, the electron starts
Phys460.nb 81 ψ n (t) is still the (same) eigenstate of H But for tdependent H. The answer is NO. 5.5.5. Solution for the tdependent Schrodinger s equation If we assume that at time t 0, the electron starts
Reminders: linear functions
 Reminders: linear functions Let U and V be vector spaces over the same field F. Definition A function f : U V is linear if for every u 1, u 2 U, f (u 1 + u 2 ) = f (u 1 ) + f (u 2 ), and for every u U
Reminders: linear functions Let U and V be vector spaces over the same field F. Definition A function f : U V is linear if for every u 1, u 2 U, f (u 1 + u 2 ) = f (u 1 ) + f (u 2 ), and for every u U
Solutions - Chapter 4
 Solutions - Chapter Kevin S. Huang Problem.1 Unitary: Ût = 1 ī hĥt Û tût = 1 Neglect t term: 1 + hĥ ī t 1 īhĥt = 1 + hĥ ī t ī hĥt = 1 Ĥ = Ĥ Problem. Ût = lim 1 ī ] n hĥ1t 1 ī ] hĥt... 1 ī ] hĥnt 1 ī ]
Solutions - Chapter Kevin S. Huang Problem.1 Unitary: Ût = 1 ī hĥt Û tût = 1 Neglect t term: 1 + hĥ ī t 1 īhĥt = 1 + hĥ ī t ī hĥt = 1 Ĥ = Ĥ Problem. Ût = lim 1 ī ] n hĥ1t 1 ī ] hĥt... 1 ī ] hĥnt 1 ī ]
Review Exercises for Chapter 7
 8 Chapter 7 Integration Techniques, L Hôpital s Rule, and Improper Integrals 8. For n, I d b For n >, I n n u n, du n n d, dv (a) d b 6 b 6 (b) (c) n d 5 d b n n b n n n d, v d 6 5 5 6 d 5 5 b d 6. b 6
8 Chapter 7 Integration Techniques, L Hôpital s Rule, and Improper Integrals 8. For n, I d b For n >, I n n u n, du n n d, dv (a) d b 6 b 6 (b) (c) n d 5 d b n n b n n n d, v d 6 5 5 6 d 5 5 b d 6. b 6
Space-Time Symmetries
 Chapter Space-Time Symmetries In classical fiel theory any continuous symmetry of the action generates a conserve current by Noether's proceure. If the Lagrangian is not invariant but only shifts by a
Chapter Space-Time Symmetries In classical fiel theory any continuous symmetry of the action generates a conserve current by Noether's proceure. If the Lagrangian is not invariant but only shifts by a
CHAPTER 25 SOLVING EQUATIONS BY ITERATIVE METHODS
 CHAPTER 5 SOLVING EQUATIONS BY ITERATIVE METHODS EXERCISE 104 Page 8 1. Find the positive root of the equation x + 3x 5 = 0, correct to 3 significant figures, using the method of bisection. Let f(x) =
CHAPTER 5 SOLVING EQUATIONS BY ITERATIVE METHODS EXERCISE 104 Page 8 1. Find the positive root of the equation x + 3x 5 = 0, correct to 3 significant figures, using the method of bisection. Let f(x) =
Œ ˆ Œ Ÿ Œˆ Ÿ ˆŸŒˆ Œˆ Ÿ ˆ œ, Ä ÞŒ Å Š ˆ ˆ Œ Œ ˆˆ
 ˆ ˆŠ Œ ˆ ˆ Œ ƒ Ÿ 018.. 49.. 4.. 907Ä917 Œ ˆ Œ Ÿ Œˆ Ÿ ˆŸŒˆ Œˆ Ÿ ˆ œ, Ä ÞŒ Å Š ˆ ˆ Œ Œ ˆˆ.. ³μ, ˆ. ˆ. Ë μ μ,.. ³ ʲ μ ± Ë ²Ó Ò Ö Ò Í É Å μ ± ÊÎ μ- ² μ É ²Ó ± É ÉÊÉ Ô± ³ É ²Ó μ Ë ±, μ, μ Ö μ ² Ìμ μé Ê Ö ±
ˆ ˆŠ Œ ˆ ˆ Œ ƒ Ÿ 018.. 49.. 4.. 907Ä917 Œ ˆ Œ Ÿ Œˆ Ÿ ˆŸŒˆ Œˆ Ÿ ˆ œ, Ä ÞŒ Å Š ˆ ˆ Œ Œ ˆˆ.. ³μ, ˆ. ˆ. Ë μ μ,.. ³ ʲ μ ± Ë ²Ó Ò Ö Ò Í É Å μ ± ÊÎ μ- ² μ É ²Ó ± É ÉÊÉ Ô± ³ É ²Ó μ Ë ±, μ, μ Ö μ ² Ìμ μé Ê Ö ±
ANSWERSHEET (TOPIC = DIFFERENTIAL CALCULUS) COLLECTION #2. h 0 h h 0 h h 0 ( ) g k = g 0 + g 1 + g g 2009 =?
 Teko Classes IITJEE/AIEEE Maths by SUHAAG SIR, Bhopal, Ph (0755) 3 00 000 www.tekoclasses.com ANSWERSHEET (TOPIC DIFFERENTIAL CALCULUS) COLLECTION # Question Type A.Single Correct Type Q. (A) Sol least
Teko Classes IITJEE/AIEEE Maths by SUHAAG SIR, Bhopal, Ph (0755) 3 00 000 www.tekoclasses.com ANSWERSHEET (TOPIC DIFFERENTIAL CALCULUS) COLLECTION # Question Type A.Single Correct Type Q. (A) Sol least
Lecture 2: Dirac notation and a review of linear algebra Read Sakurai chapter 1, Baym chatper 3
 Lecture 2: Dirac notation and a review of linear algebra Read Sakurai chapter 1, Baym chatper 3 1 State vector space and the dual space Space of wavefunctions The space of wavefunctions is the set of all
Lecture 2: Dirac notation and a review of linear algebra Read Sakurai chapter 1, Baym chatper 3 1 State vector space and the dual space Space of wavefunctions The space of wavefunctions is the set of all
1 String with massive end-points
 1 String with massive end-points Πρόβλημα 5.11:Θεωρείστε μια χορδή μήκους, τάσης T, με δύο σημειακά σωματίδια στα άκρα της, το ένα μάζας m, και το άλλο μάζας m. α) Μελετώντας την κίνηση των άκρων βρείτε
1 String with massive end-points Πρόβλημα 5.11:Θεωρείστε μια χορδή μήκους, τάσης T, με δύο σημειακά σωματίδια στα άκρα της, το ένα μάζας m, και το άλλο μάζας m. α) Μελετώντας την κίνηση των άκρων βρείτε
Geodesic Equations for the Wormhole Metric
 Geodesic Equations for the Wormhole Metric Dr R Herman Physics & Physical Oceanography, UNCW February 14, 2018 The Wormhole Metric Morris and Thorne wormhole metric: [M S Morris, K S Thorne, Wormholes
Geodesic Equations for the Wormhole Metric Dr R Herman Physics & Physical Oceanography, UNCW February 14, 2018 The Wormhole Metric Morris and Thorne wormhole metric: [M S Morris, K S Thorne, Wormholes
k A = [k, k]( )[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R +
[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R + k A = [k, k]( )[a 1, a 2 ] = [ka 1,ka 2 ] 4For the division of two intervals of confidence in R +](/thumbs/73/69566903.jpg) Chapter 3. Fuzzy Arithmetic 3- Fuzzy arithmetic: ~Addition(+) and subtraction (-): Let A = [a and B = [b, b in R If x [a and y [b, b than x+y [a +b +b Symbolically,we write A(+)B = [a (+)[b, b = [a +b
Chapter 3. Fuzzy Arithmetic 3- Fuzzy arithmetic: ~Addition(+) and subtraction (-): Let A = [a and B = [b, b in R If x [a and y [b, b than x+y [a +b +b Symbolically,we write A(+)B = [a (+)[b, b = [a +b
Answers to practice exercises
 Answers to practice exercises Chapter Exercise (Page 5). 9 kg 2. 479 mm. 66 4. 565 5. 225 6. 26 7. 07,70 8. 4 9. 487 0. 70872. $5, Exercise 2 (Page 6). (a) 468 (b) 868 2. (a) 827 (b) 458. (a) 86 kg (b)
Answers to practice exercises Chapter Exercise (Page 5). 9 kg 2. 479 mm. 66 4. 565 5. 225 6. 26 7. 07,70 8. 4 9. 487 0. 70872. $5, Exercise 2 (Page 6). (a) 468 (b) 868 2. (a) 827 (b) 458. (a) 86 kg (b)
HOMEWORK 4 = G. In order to plot the stress versus the stretch we define a normalized stretch:
 HOMEWORK 4 Problem a For the fast loading case, we want to derive the relationship between P zz and λ z. We know that the nominal stress is expressed as: P zz = ψ λ z where λ z = λ λ z. Therefore, applying
HOMEWORK 4 Problem a For the fast loading case, we want to derive the relationship between P zz and λ z. We know that the nominal stress is expressed as: P zz = ψ λ z where λ z = λ λ z. Therefore, applying
Lecture 34 Bootstrap confidence intervals
 Lecture 34 Bootstrap confidence intervals Confidence Intervals θ: an unknown parameter of interest We want to find limits θ and θ such that Gt = P nˆθ θ t If G 1 1 α is known, then P θ θ = P θ θ = 1 α
Lecture 34 Bootstrap confidence intervals Confidence Intervals θ: an unknown parameter of interest We want to find limits θ and θ such that Gt = P nˆθ θ t If G 1 1 α is known, then P θ θ = P θ θ = 1 α
If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2
![If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2 If we restrict the domain of y = sin x to [ π, π ], the restrict function. y = sin x, π 2 x π 2](/thumbs/53/31933086.jpg) Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Efectos de la cromodinámica cuántica en la física del bosón de Higgs Mazzitelli, Javier
 Efectos de la cromodinámica cuántica en la física del bosón de Higgs Mazzitelli, Javier 2016 07 22 Tesis Doctoral Facultad de Ciencias Exactas y Naturales Universidad de Buenos Aires www.digital.bl.fcen.uba.ar
Efectos de la cromodinámica cuántica en la física del bosón de Higgs Mazzitelli, Javier 2016 07 22 Tesis Doctoral Facultad de Ciencias Exactas y Naturales Universidad de Buenos Aires www.digital.bl.fcen.uba.ar
C.S. 430 Assignment 6, Sample Solutions
 C.S. 430 Assignment 6, Sample Solutions Paul Liu November 15, 2007 Note that these are sample solutions only; in many cases there were many acceptable answers. 1 Reynolds Problem 10.1 1.1 Normal-order
C.S. 430 Assignment 6, Sample Solutions Paul Liu November 15, 2007 Note that these are sample solutions only; in many cases there were many acceptable answers. 1 Reynolds Problem 10.1 1.1 Normal-order
Inverse trigonometric functions & General Solution of Trigonometric Equations. ------------------ ----------------------------- -----------------
 Inverse trigonometric functions & General Solution of Trigonometric Equations. 1. Sin ( ) = a) b) c) d) Ans b. Solution : Method 1. Ans a: 17 > 1 a) is rejected. w.k.t Sin ( sin ) = d is rejected. If sin
Inverse trigonometric functions & General Solution of Trigonometric Equations. 1. Sin ( ) = a) b) c) d) Ans b. Solution : Method 1. Ans a: 17 > 1 a) is rejected. w.k.t Sin ( sin ) = d is rejected. If sin
Jeux d inondation dans les graphes
 Jeux d inondation dans les graphes Aurélie Lagoutte To cite this version: Aurélie Lagoutte. Jeux d inondation dans les graphes. 2010. HAL Id: hal-00509488 https://hal.archives-ouvertes.fr/hal-00509488
Jeux d inondation dans les graphes Aurélie Lagoutte To cite this version: Aurélie Lagoutte. Jeux d inondation dans les graphes. 2010. HAL Id: hal-00509488 https://hal.archives-ouvertes.fr/hal-00509488
If we restrict the domain of y = sin x to [ π 2, π 2
 Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Chapter 3. Analytic Trigonometry 3.1 The inverse sine, cosine, and tangent functions 1. Review: Inverse function (1) f 1 (f(x)) = x for every x in the domain of f and f(f 1 (x)) = x for every x in the
Pg The perimeter is P = 3x The area of a triangle is. where b is the base, h is the height. In our case b = x, then the area is
 Pg. 9. The perimeter is P = The area of a triangle is A = bh where b is the base, h is the height 0 h= btan 60 = b = b In our case b =, then the area is A = = 0. By Pythagorean theorem a + a = d a a =
Pg. 9. The perimeter is P = The area of a triangle is A = bh where b is the base, h is the height 0 h= btan 60 = b = b In our case b =, then the area is A = = 0. By Pythagorean theorem a + a = d a a =
Lifting Entry (continued)
 ifting Entry (continued) Basic planar dynamics of motion, again Yet another equilibrium glide Hypersonic phugoid motion Planar state equations MARYAN 1 01 avid. Akin - All rights reserved http://spacecraft.ssl.umd.edu
ifting Entry (continued) Basic planar dynamics of motion, again Yet another equilibrium glide Hypersonic phugoid motion Planar state equations MARYAN 1 01 avid. Akin - All rights reserved http://spacecraft.ssl.umd.edu
Approximation of distance between locations on earth given by latitude and longitude
 Approximation of distance between locations on earth given by latitude and longitude Jan Behrens 2012-12-31 In this paper we shall provide a method to approximate distances between two points on earth
Approximation of distance between locations on earth given by latitude and longitude Jan Behrens 2012-12-31 In this paper we shall provide a method to approximate distances between two points on earth
Exercises 10. Find a fundamental matrix of the given system of equations. Also find the fundamental matrix Φ(t) satisfying Φ(0) = I. 1.
 Exercises 0 More exercises are available in Elementary Differential Equations. If you have a problem to solve any of them, feel free to come to office hour. Problem Find a fundamental matrix of the given
Exercises 0 More exercises are available in Elementary Differential Equations. If you have a problem to solve any of them, feel free to come to office hour. Problem Find a fundamental matrix of the given
Tridiagonal matrices. Gérard MEURANT. October, 2008
 Tridiagonal matrices Gérard MEURANT October, 2008 1 Similarity 2 Cholesy factorizations 3 Eigenvalues 4 Inverse Similarity Let α 1 ω 1 β 1 α 2 ω 2 T =......... β 2 α 1 ω 1 β 1 α and β i ω i, i = 1,...,
Tridiagonal matrices Gérard MEURANT October, 2008 1 Similarity 2 Cholesy factorizations 3 Eigenvalues 4 Inverse Similarity Let α 1 ω 1 β 1 α 2 ω 2 T =......... β 2 α 1 ω 1 β 1 α and β i ω i, i = 1,...,
D Alembert s Solution to the Wave Equation
 D Alembert s Solution to the Wave Equation MATH 467 Partial Differential Equations J. Robert Buchanan Department of Mathematics Fall 2018 Objectives In this lesson we will learn: a change of variable technique
D Alembert s Solution to the Wave Equation MATH 467 Partial Differential Equations J. Robert Buchanan Department of Mathematics Fall 2018 Objectives In this lesson we will learn: a change of variable technique
Math221: HW# 1 solutions
 Math: HW# solutions Andy Royston October, 5 7.5.7, 3 rd Ed. We have a n = b n = a = fxdx = xdx =, x cos nxdx = x sin nx n sin nxdx n = cos nx n = n n, x sin nxdx = x cos nx n + cos nxdx n cos n = + sin
Math: HW# solutions Andy Royston October, 5 7.5.7, 3 rd Ed. We have a n = b n = a = fxdx = xdx =, x cos nxdx = x sin nx n sin nxdx n = cos nx n = n n, x sin nxdx = x cos nx n + cos nxdx n cos n = + sin
Orbital angular momentum and the spherical harmonics
 Orbital angular momentum and the spherical harmonics March 8, 03 Orbital angular momentum We compare our result on representations of rotations with our previous experience of angular momentum, defined
Orbital angular momentum and the spherical harmonics March 8, 03 Orbital angular momentum We compare our result on representations of rotations with our previous experience of angular momentum, defined
Dong Liu State Key Laboratory of Particle Detection and Electronics University of Science and Technology of China
 Dong Liu State Key Laboratory of Particle Detection and Electronics University of Science and Technology of China ISSP, Erice, 7 Outline Introduction of BESIII experiment Motivation of the study Data sample
Dong Liu State Key Laboratory of Particle Detection and Electronics University of Science and Technology of China ISSP, Erice, 7 Outline Introduction of BESIII experiment Motivation of the study Data sample
4.6 Autoregressive Moving Average Model ARMA(1,1)
 84 CHAPTER 4. STATIONARY TS MODELS 4.6 Autoregressive Moving Average Model ARMA(,) This section is an introduction to a wide class of models ARMA(p,q) which we will consider in more detail later in this
84 CHAPTER 4. STATIONARY TS MODELS 4.6 Autoregressive Moving Average Model ARMA(,) This section is an introduction to a wide class of models ARMA(p,q) which we will consider in more detail later in this
Higher Derivative Gravity Theories
 Higher Derivative Gravity Theories Black Holes in AdS space-times James Mashiyane Supervisor: Prof Kevin Goldstein University of the Witwatersrand Second Mandelstam, 20 January 2018 James Mashiyane WITS)
Higher Derivative Gravity Theories Black Holes in AdS space-times James Mashiyane Supervisor: Prof Kevin Goldstein University of the Witwatersrand Second Mandelstam, 20 January 2018 James Mashiyane WITS)
Srednicki Chapter 55
 Srednicki Chapter 55 QFT Problems & Solutions A. George August 3, 03 Srednicki 55.. Use equations 55.3-55.0 and A i, A j ] = Π i, Π j ] = 0 (at equal times) to verify equations 55.-55.3. This is our third
Srednicki Chapter 55 QFT Problems & Solutions A. George August 3, 03 Srednicki 55.. Use equations 55.3-55.0 and A i, A j ] = Π i, Π j ] = 0 (at equal times) to verify equations 55.-55.3. This is our third
Solutions to Exercise Sheet 5
 Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
Solutions to Eercise Sheet 5 jacques@ucsd.edu. Let X and Y be random variables with joint pdf f(, y) = 3y( + y) where and y. Determine each of the following probabilities. Solutions. a. P (X ). b. P (X
 Problem 7.19 Ignoring reflection at the air soil boundary, if the amplitude of a 3-GHz incident wave is 10 V/m at the surface of a wet soil medium, at what depth will it be down to 1 mv/m? Wet soil is
Problem 7.19 Ignoring reflection at the air soil boundary, if the amplitude of a 3-GHz incident wave is 10 V/m at the surface of a wet soil medium, at what depth will it be down to 1 mv/m? Wet soil is
Second Order RLC Filters
 ECEN 60 Circuits/Electronics Spring 007-0-07 P. Mathys Second Order RLC Filters RLC Lowpass Filter A passive RLC lowpass filter (LPF) circuit is shown in the following schematic. R L C v O (t) Using phasor
ECEN 60 Circuits/Electronics Spring 007-0-07 P. Mathys Second Order RLC Filters RLC Lowpass Filter A passive RLC lowpass filter (LPF) circuit is shown in the following schematic. R L C v O (t) Using phasor
Review Test 3. MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question.
 Review Test MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question. Find the exact value of the expression. 1) sin - 11π 1 1) + - + - - ) sin 11π 1 ) ( -
Review Test MULTIPLE CHOICE. Choose the one alternative that best completes the statement or answers the question. Find the exact value of the expression. 1) sin - 11π 1 1) + - + - - ) sin 11π 1 ) ( -
Uniform Convergence of Fourier Series Michael Taylor
 Uniform Convergence of Fourier Series Michael Taylor Given f L 1 T 1 ), we consider the partial sums of the Fourier series of f: N 1) S N fθ) = ˆfk)e ikθ. k= N A calculation gives the Dirichlet formula
Uniform Convergence of Fourier Series Michael Taylor Given f L 1 T 1 ), we consider the partial sums of the Fourier series of f: N 1) S N fθ) = ˆfk)e ikθ. k= N A calculation gives the Dirichlet formula
Derivation of Optical-Bloch Equations
 Appendix C Derivation of Optical-Bloch Equations In this appendix the optical-bloch equations that give the populations and coherences for an idealized three-level Λ system, Fig. 3. on page 47, will be
Appendix C Derivation of Optical-Bloch Equations In this appendix the optical-bloch equations that give the populations and coherences for an idealized three-level Λ system, Fig. 3. on page 47, will be
( N m 2 /C 2 )( C)( C) J
 Electrical Energy and Capacitance Practice 8A, p. 669 Chapter 8. PE electric = 6.3 0 9 J q = q = q p + q n = ().60 0 9 C + ()(0) = 3.0 0 9 C kcqq (8.99 0 9 N /C )(3.0 0 9 C) r = = P Ee lectric 6.3 0 9
Electrical Energy and Capacitance Practice 8A, p. 669 Chapter 8. PE electric = 6.3 0 9 J q = q = q p + q n = ().60 0 9 C + ()(0) = 3.0 0 9 C kcqq (8.99 0 9 N /C )(3.0 0 9 C) r = = P Ee lectric 6.3 0 9
Non-Abelian Gauge Fields
 Chapter 5 Non-Abelian Gauge Fields The simplest example starts with two Fermions Dirac particles) ψ 1, ψ 2, degenerate in mass, and hence satisfying in the absence of interactions γ 1 i + m)ψ 1 = 0, γ
Chapter 5 Non-Abelian Gauge Fields The simplest example starts with two Fermions Dirac particles) ψ 1, ψ 2, degenerate in mass, and hence satisfying in the absence of interactions γ 1 i + m)ψ 1 = 0, γ
Lifting Entry 2. Basic planar dynamics of motion, again Yet another equilibrium glide Hypersonic phugoid motion MARYLAND U N I V E R S I T Y O F
 ifting Entry Basic planar dynamics of motion, again Yet another equilibrium glide Hypersonic phugoid motion MARYAN 1 010 avid. Akin - All rights reserved http://spacecraft.ssl.umd.edu ifting Atmospheric
ifting Entry Basic planar dynamics of motion, again Yet another equilibrium glide Hypersonic phugoid motion MARYAN 1 010 avid. Akin - All rights reserved http://spacecraft.ssl.umd.edu ifting Atmospheric
!!" #7 $39 %" (07) ..,..,.. $ 39. ) :. :, «(», «%», «%», «%» «%». & ,. ). & :..,. '.. ( () #*. );..,..'. + (# ).
 1 00 3 !!" 344#7 $39 %" 6181001 63(07) & : ' ( () #* ); ' + (# ) $ 39 ) : : 00 %" 6181001 63(07)!!" 344#7 «(» «%» «%» «%» «%» & ) 4 )&-%/0 +- «)» * «1» «1» «)» ) «(» «%» «%» + ) 30 «%» «%» )1+ / + : +3
1 00 3 !!" 344#7 $39 %" 6181001 63(07) & : ' ( () #* ); ' + (# ) $ 39 ) : : 00 %" 6181001 63(07)!!" 344#7 «(» «%» «%» «%» «%» & ) 4 )&-%/0 +- «)» * «1» «1» «)» ) «(» «%» «%» + ) 30 «%» «%» )1+ / + : +3
Areas and Lengths in Polar Coordinates
 Kiryl Tsishchanka Areas and Lengths in Polar Coordinates In this section we develop the formula for the area of a region whose boundary is given by a polar equation. We need to use the formula for the
Kiryl Tsishchanka Areas and Lengths in Polar Coordinates In this section we develop the formula for the area of a region whose boundary is given by a polar equation. We need to use the formula for the
Appendix to On the stability of a compressible axisymmetric rotating flow in a pipe. By Z. Rusak & J. H. Lee
 Appendi to On the stability of a compressible aisymmetric rotating flow in a pipe By Z. Rusak & J. H. Lee Journal of Fluid Mechanics, vol. 5 4, pp. 5 4 This material has not been copy-edited or typeset
Appendi to On the stability of a compressible aisymmetric rotating flow in a pipe By Z. Rusak & J. H. Lee Journal of Fluid Mechanics, vol. 5 4, pp. 5 4 This material has not been copy-edited or typeset
L. F avart. CLAS12 Workshop Genova th of Feb CLAS12 workshop Feb L.Favart p.1/28
 L. F avart I.I.H.E. Université Libre de Bruxelles H Collaboration HERA at DESY CLAS Workshop Genova - 4-8 th of Feb. 9 CLAS workshop Feb. 9 - L.Favart p./8 e p Integrated luminosity 96- + 3-7 (high energy)
L. F avart I.I.H.E. Université Libre de Bruxelles H Collaboration HERA at DESY CLAS Workshop Genova - 4-8 th of Feb. 9 CLAS workshop Feb. 9 - L.Favart p./8 e p Integrated luminosity 96- + 3-7 (high energy)
( ) 2 and compare to M.
 Problems and Solutions for Section 4.2 4.9 through 4.33) 4.9 Calculate the square root of the matrix 3!0 M!0 8 Hint: Let M / 2 a!b ; calculate M / 2!b c ) 2 and compare to M. Solution: Given: 3!0 M!0 8
Problems and Solutions for Section 4.2 4.9 through 4.33) 4.9 Calculate the square root of the matrix 3!0 M!0 8 Hint: Let M / 2 a!b ; calculate M / 2!b c ) 2 and compare to M. Solution: Given: 3!0 M!0 8
2 Composition. Invertible Mappings
 Arkansas Tech University MATH 4033: Elementary Modern Algebra Dr. Marcel B. Finan Composition. Invertible Mappings In this section we discuss two procedures for creating new mappings from old ones, namely,
Arkansas Tech University MATH 4033: Elementary Modern Algebra Dr. Marcel B. Finan Composition. Invertible Mappings In this section we discuss two procedures for creating new mappings from old ones, namely,
Matrices and Determinants
 Matrices and Determinants SUBJECTIVE PROBLEMS: Q 1. For what value of k do the following system of equations possess a non-trivial (i.e., not all zero) solution over the set of rationals Q? x + ky + 3z
Matrices and Determinants SUBJECTIVE PROBLEMS: Q 1. For what value of k do the following system of equations possess a non-trivial (i.e., not all zero) solution over the set of rationals Q? x + ky + 3z
EE101: Resonance in RLC circuits
 EE11: Resonance in RLC circuits M. B. Patil mbatil@ee.iitb.ac.in www.ee.iitb.ac.in/~sequel Deartment of Electrical Engineering Indian Institute of Technology Bombay I V R V L V C I = I m = R + jωl + 1/jωC
EE11: Resonance in RLC circuits M. B. Patil mbatil@ee.iitb.ac.in www.ee.iitb.ac.in/~sequel Deartment of Electrical Engineering Indian Institute of Technology Bombay I V R V L V C I = I m = R + jωl + 1/jωC
Trigonometric Formula Sheet
 Trigonometric Formula Sheet Definition of the Trig Functions Right Triangle Definition Assume that: 0 < θ < or 0 < θ < 90 Unit Circle Definition Assume θ can be any angle. y x, y hypotenuse opposite θ
Trigonometric Formula Sheet Definition of the Trig Functions Right Triangle Definition Assume that: 0 < θ < or 0 < θ < 90 Unit Circle Definition Assume θ can be any angle. y x, y hypotenuse opposite θ
Problem 3.16 Given B = ˆx(z 3y) +ŷ(2x 3z) ẑ(x+y), find a unit vector parallel. Solution: At P = (1,0, 1), ˆb = B
 Problem 3.6 Given B = ˆxz 3y) +ŷx 3z) ẑx+y), find a unit vector parallel to B at point P =,0, ). Solution: At P =,0, ), B = ˆx )+ŷ+3) ẑ) = ˆx+ŷ5 ẑ, ˆb = B B = ˆx+ŷ5 ẑ = ˆx+ŷ5 ẑ. +5+ 7 Problem 3.4 Convert
Problem 3.6 Given B = ˆxz 3y) +ŷx 3z) ẑx+y), find a unit vector parallel to B at point P =,0, ). Solution: At P =,0, ), B = ˆx )+ŷ+3) ẑ) = ˆx+ŷ5 ẑ, ˆb = B B = ˆx+ŷ5 ẑ = ˆx+ŷ5 ẑ. +5+ 7 Problem 3.4 Convert
Second Order Partial Differential Equations
 Chapter 7 Second Order Partial Differential Equations 7.1 Introduction A second order linear PDE in two independent variables (x, y Ω can be written as A(x, y u x + B(x, y u xy + C(x, y u u u + D(x, y
Chapter 7 Second Order Partial Differential Equations 7.1 Introduction A second order linear PDE in two independent variables (x, y Ω can be written as A(x, y u x + B(x, y u xy + C(x, y u u u + D(x, y
Π Ο Λ Ι Τ Ι Κ Α Κ Α Ι Σ Τ Ρ Α Τ Ι Ω Τ Ι Κ Α Γ Ε Γ Ο Ν Ο Τ Α
 Α Ρ Χ Α Ι Α Ι Σ Τ Ο Ρ Ι Α Π Ο Λ Ι Τ Ι Κ Α Κ Α Ι Σ Τ Ρ Α Τ Ι Ω Τ Ι Κ Α Γ Ε Γ Ο Ν Ο Τ Α Σ η µ ε ί ω σ η : σ υ ν ά δ ε λ φ ο ι, ν α µ ο υ σ υ γ χ ω ρ ή σ ε τ ε τ ο γ ρ ή γ ο ρ ο κ α ι α τ η µ έ λ η τ ο ύ
Α Ρ Χ Α Ι Α Ι Σ Τ Ο Ρ Ι Α Π Ο Λ Ι Τ Ι Κ Α Κ Α Ι Σ Τ Ρ Α Τ Ι Ω Τ Ι Κ Α Γ Ε Γ Ο Ν Ο Τ Α Σ η µ ε ί ω σ η : σ υ ν ά δ ε λ φ ο ι, ν α µ ο υ σ υ γ χ ω ρ ή σ ε τ ε τ ο γ ρ ή γ ο ρ ο κ α ι α τ η µ έ λ η τ ο ύ
Graded Refractive-Index
 Graded Refractive-Index Common Devices Methodologies for Graded Refractive Index Methodologies: Ray Optics WKB Multilayer Modelling Solution requires: some knowledge of index profile n 2 x Ray Optics for
Graded Refractive-Index Common Devices Methodologies for Graded Refractive Index Methodologies: Ray Optics WKB Multilayer Modelling Solution requires: some knowledge of index profile n 2 x Ray Optics for
C 1 D 1. AB = a, AD = b, AA1 = c. a, b, c : (1) AC 1 ; : (1) AB + BC + CC1, AC 1 = BC = AD, CC1 = AA 1, AC 1 = a + b + c. (2) BD 1 = BD + DD 1,
 1 1., BD 1 B 1 1 D 1, E F B 1 D 1. B = a, D = b, 1 = c. a, b, c : (1) 1 ; () BD 1 ; () F; D 1 F 1 (4) EF. : (1) B = D, D c b 1 E a B 1 1 = 1, B1 1 = B + B + 1, 1 = a + b + c. () BD 1 = BD + DD 1, BD =
1 1., BD 1 B 1 1 D 1, E F B 1 D 1. B = a, D = b, 1 = c. a, b, c : (1) 1 ; () BD 1 ; () F; D 1 F 1 (4) EF. : (1) B = D, D c b 1 E a B 1 1 = 1, B1 1 = B + B + 1, 1 = a + b + c. () BD 1 = BD + DD 1, BD =
Notes on the Open Economy
 Notes on the Open Econom Ben J. Heijdra Universit of Groningen April 24 Introduction In this note we stud the two-countr model of Table.4 in more detail. restated here for convenience. The model is Table.4.
Notes on the Open Econom Ben J. Heijdra Universit of Groningen April 24 Introduction In this note we stud the two-countr model of Table.4 in more detail. restated here for convenience. The model is Table.4.
Oscillatory integrals
 Oscilltory integrls Jordn Bell jordn.bell@gmil.com Deprtment of Mthemtics, University of Toronto August, 0 Oscilltory integrls Suppose tht Φ C R d ), ψ DR d ), nd tht Φ is rel-vlued. I : 0, ) C by Iλ)
Oscilltory integrls Jordn Bell jordn.bell@gmil.com Deprtment of Mthemtics, University of Toronto August, 0 Oscilltory integrls Suppose tht Φ C R d ), ψ DR d ), nd tht Φ is rel-vlued. I : 0, ) C by Iλ)
상대론적고에너지중이온충돌에서 제트입자와관련된제동복사 박가영 인하대학교 윤진희교수님, 권민정교수님
 상대론적고에너지중이온충돌에서 제트입자와관련된제동복사 박가영 인하대학교 윤진희교수님, 권민정교수님 Motivation Bremsstrahlung is a major rocess losing energies while jet articles get through the medium. BUT it should be quite different from low energy
상대론적고에너지중이온충돌에서 제트입자와관련된제동복사 박가영 인하대학교 윤진희교수님, 권민정교수님 Motivation Bremsstrahlung is a major rocess losing energies while jet articles get through the medium. BUT it should be quite different from low energy
Trigonometry 1.TRIGONOMETRIC RATIOS
 Trigonometry.TRIGONOMETRIC RATIOS. If a ray OP makes an angle with the positive direction of X-axis then y x i) Sin ii) cos r r iii) tan x y (x 0) iv) cot y x (y 0) y P v) sec x r (x 0) vi) cosec y r (y
Trigonometry.TRIGONOMETRIC RATIOS. If a ray OP makes an angle with the positive direction of X-axis then y x i) Sin ii) cos r r iii) tan x y (x 0) iv) cot y x (y 0) y P v) sec x r (x 0) vi) cosec y r (y
CHAPTER (2) Electric Charges, Electric Charge Densities and Electric Field Intensity
 CHAPTE () Electric Chrges, Electric Chrge Densities nd Electric Field Intensity Chrge Configurtion ) Point Chrge: The concept of the point chrge is used when the dimensions of n electric chrge distriution
CHAPTE () Electric Chrges, Electric Chrge Densities nd Electric Field Intensity Chrge Configurtion ) Point Chrge: The concept of the point chrge is used when the dimensions of n electric chrge distriution
Problem 3.1 Vector A starts at point (1, 1, 3) and ends at point (2, 1,0). Find a unit vector in the direction of A. Solution: A = 1+9 = 3.
 Problem 3.1 Vector A starts at point (1, 1, 3) and ends at point (, 1,0). Find a unit vector in the direction of A. Solution: A = ˆx( 1)+ŷ( 1 ( 1))+ẑ(0 ( 3)) = ˆx+ẑ3, A = 1+9 = 3.16, â = A A = ˆx+ẑ3 3.16
Problem 3.1 Vector A starts at point (1, 1, 3) and ends at point (, 1,0). Find a unit vector in the direction of A. Solution: A = ˆx( 1)+ŷ( 1 ( 1))+ẑ(0 ( 3)) = ˆx+ẑ3, A = 1+9 = 3.16, â = A A = ˆx+ẑ3 3.16
Ó³ Ÿ , º 7(170) Ä1091 ˆŒ ˆ. Œ. ˆ. Ò μí± 1. ˆ É ÉÊÉ É μ É Î ±μ Ô± ³ É ²Ó μ Ë ±, Œμ ±
 Ó³ Ÿ. 011.. 8, º 7(170.. 1038Ä1091 Š ˆˆ ˆˆ Š ˆŒ ˆ Œ. ˆ. Ò μí± 1 ˆ É ÉÊÉ É μ É Î ±μ Ô± ³ É ²Ó μ Ë ±, Œμ ± Î ÉÒ Ì ² ±Í ÖÌ ² É Ö É μ Ö Ô² ±É μ ² ÒÌ ³μ É. Theory of electroweak interactions is given in 4 lectures.
Ó³ Ÿ. 011.. 8, º 7(170.. 1038Ä1091 Š ˆˆ ˆˆ Š ˆŒ ˆ Œ. ˆ. Ò μí± 1 ˆ É ÉÊÉ É μ É Î ±μ Ô± ³ É ²Ó μ Ë ±, Œμ ± Î ÉÒ Ì ² ±Í ÖÌ ² É Ö É μ Ö Ô² ±É μ ² ÒÌ ³μ É. Theory of electroweak interactions is given in 4 lectures.
Chapter 6 BLM Answers
 Chapter 6 BLM Answers BLM 6 Chapter 6 Prerequisite Skills. a) i) II ii) IV iii) III i) 5 ii) 7 iii) 7. a) 0, c) 88.,.6, 59.6 d). a) 5 + 60 n; 7 + n, c). rad + n rad; 7 9,. a) 5 6 c) 69. d) 0.88 5. a) negative
Chapter 6 BLM Answers BLM 6 Chapter 6 Prerequisite Skills. a) i) II ii) IV iii) III i) 5 ii) 7 iii) 7. a) 0, c) 88.,.6, 59.6 d). a) 5 + 60 n; 7 + n, c). rad + n rad; 7 9,. a) 5 6 c) 69. d) 0.88 5. a) negative
= 0.927rad, t = 1.16ms
 P 9. [a] ω = 2πf = 800rad/s, f = ω 2π = 27.32Hz [b] T = /f = 7.85ms [c] I m = 25mA [d] i(0) = 25cos(36.87 ) = 00mA [e] φ = 36.87 ; φ = 36.87 (2π) = 0.6435 rad 360 [f] i = 0 when 800t + 36.87 = 90. Now
P 9. [a] ω = 2πf = 800rad/s, f = ω 2π = 27.32Hz [b] T = /f = 7.85ms [c] I m = 25mA [d] i(0) = 25cos(36.87 ) = 00mA [e] φ = 36.87 ; φ = 36.87 (2π) = 0.6435 rad 360 [f] i = 0 when 800t + 36.87 = 90. Now
Example Sheet 3 Solutions
 Example Sheet 3 Solutions. i Regular Sturm-Liouville. ii Singular Sturm-Liouville mixed boundary conditions. iii Not Sturm-Liouville ODE is not in Sturm-Liouville form. iv Regular Sturm-Liouville note
Example Sheet 3 Solutions. i Regular Sturm-Liouville. ii Singular Sturm-Liouville mixed boundary conditions. iii Not Sturm-Liouville ODE is not in Sturm-Liouville form. iv Regular Sturm-Liouville note
Particle Physics: Introduction to the Standard Model
 Particle Physics: Introduction to the Standard Model Electroweak theory (I) Frédéric Machefert frederic@cern.ch Laboratoire de l accélérateur linéaire (CNRS) Cours de l École Normale Supérieure 4, rue
Particle Physics: Introduction to the Standard Model Electroweak theory (I) Frédéric Machefert frederic@cern.ch Laboratoire de l accélérateur linéaire (CNRS) Cours de l École Normale Supérieure 4, rue
Areas and Lengths in Polar Coordinates
 Kiryl Tsishchanka Areas and Lengths in Polar Coordinates In this section we develop the formula for the area of a region whose boundary is given by a polar equation. We need to use the formula for the
Kiryl Tsishchanka Areas and Lengths in Polar Coordinates In this section we develop the formula for the area of a region whose boundary is given by a polar equation. We need to use the formula for the
SPECIAL FUNCTIONS and POLYNOMIALS
 SPECIAL FUNCTIONS and POLYNOMIALS Gerard t Hooft Stefan Nobbenhuis Institute for Theoretical Physics Utrecht University, Leuvenlaan 4 3584 CC Utrecht, the Netherlands and Spinoza Institute Postbox 8.195
SPECIAL FUNCTIONS and POLYNOMIALS Gerard t Hooft Stefan Nobbenhuis Institute for Theoretical Physics Utrecht University, Leuvenlaan 4 3584 CC Utrecht, the Netherlands and Spinoza Institute Postbox 8.195
MATH423 String Theory Solutions 4. = 0 τ = f(s). (1) dτ ds = dxµ dτ f (s) (2) dτ 2 [f (s)] 2 + dxµ. dτ f (s) (3)
![MATH423 String Theory Solutions 4. = 0 τ = f(s). (1) dτ ds = dxµ dτ f (s) (2) dτ 2 [f (s)] 2 + dxµ. dτ f (s) (3) MATH423 String Theory Solutions 4. = 0 τ = f(s). (1) dτ ds = dxµ dτ f (s) (2) dτ 2 [f (s)] 2 + dxµ. dτ f (s) (3)](/thumbs/81/84712331.jpg) 1. MATH43 String Theory Solutions 4 x = 0 τ = fs). 1) = = f s) ) x = x [f s)] + f s) 3) equation of motion is x = 0 if an only if f s) = 0 i.e. fs) = As + B with A, B constants. i.e. allowe reparametrisations
1. MATH43 String Theory Solutions 4 x = 0 τ = fs). 1) = = f s) ) x = x [f s)] + f s) 3) equation of motion is x = 0 if an only if f s) = 0 i.e. fs) = As + B with A, B constants. i.e. allowe reparametrisations
Differential equations
 Differential equations Differential equations: An equation inoling one dependent ariable and its deriaties w. r. t one or more independent ariables is called a differential equation. Order of differential
Differential equations Differential equations: An equation inoling one dependent ariable and its deriaties w. r. t one or more independent ariables is called a differential equation. Order of differential
Exercise 1.1. Verify that if we apply GS to the coordinate basis Gauss form ds 2 = E(u, v)du 2 + 2F (u, v)dudv + G(u, v)dv 2
 Math 209 Riemannian Geometry Jeongmin Shon Problem. Let M 2 R 3 be embedded surface. Then the induced metric on M 2 is obtained by taking the standard inner product on R 3 and restricting it to the tangent
Math 209 Riemannian Geometry Jeongmin Shon Problem. Let M 2 R 3 be embedded surface. Then the induced metric on M 2 is obtained by taking the standard inner product on R 3 and restricting it to the tangent
X t m X t Y t Z t Y t l Z t k X t h x Z t h z Z t Y t h y z X t Y t Z t E. G γ. F θ. z Θ Γ. γ F θ
 R X t m X t Y t Z t Y t l Z t k X t hxz t hzz t Y t hy z X t Y t Z t E F { f( y z; θ); θ Θ R p } θ G { g( y z; γ); γ Γ R q } γ ΘΓ z ΘΓ F θ θ γ F θ G γ G γ E [] = () h( y, z) dydz h( z) () h( y z) dydz
R X t m X t Y t Z t Y t l Z t k X t hxz t hzz t Y t hy z X t Y t Z t E F { f( y z; θ); θ Θ R p } θ G { g( y z; γ); γ Γ R q } γ ΘΓ z ΘΓ F θ θ γ F θ G γ G γ E [] = () h( y, z) dydz h( z) () h( y z) dydz
Physique des réacteurs à eau lourde ou légère en cycle thorium : étude par simulation des performances de conversion et de sûreté
 Physique des réacteurs à eau lourde ou légère en cycle thorium : étude par simulation des performances de conversion et de sûreté Alexis Nuttin To cite this version: Alexis Nuttin. Physique des réacteurs
Physique des réacteurs à eau lourde ou légère en cycle thorium : étude par simulation des performances de conversion et de sûreté Alexis Nuttin To cite this version: Alexis Nuttin. Physique des réacteurs
rs r r â t át r st tíst Ó P ã t r r r â
 rs r r â t át r st tíst P Ó P ã t r r r â ã t r r P Ó P r sã rs r s t à r çã rs r st tíst r q s t r r t çã r r st tíst r t r ú r s r ú r â rs r r â t át r çã rs r st tíst 1 r r 1 ss rt q çã st tr sã
rs r r â t át r st tíst P Ó P ã t r r r â ã t r r P Ó P r sã rs r s t à r çã rs r st tíst r q s t r r t çã r r st tíst r t r ú r s r ú r â rs r r â t át r çã rs r st tíst 1 r r 1 ss rt q çã st tr sã
Parametrized Surfaces
 Parametrized Surfaces Recall from our unit on vector-valued functions at the beginning of the semester that an R 3 -valued function c(t) in one parameter is a mapping of the form c : I R 3 where I is some
Parametrized Surfaces Recall from our unit on vector-valued functions at the beginning of the semester that an R 3 -valued function c(t) in one parameter is a mapping of the form c : I R 3 where I is some
Written Examination. Antennas and Propagation (AA ) April 26, 2017.
 Written Examination Antennas and Propagation (AA. 6-7) April 6, 7. Problem ( points) Let us consider a wire antenna as in Fig. characterized by a z-oriented linear filamentary current I(z) = I cos(kz)ẑ
Written Examination Antennas and Propagation (AA. 6-7) April 6, 7. Problem ( points) Let us consider a wire antenna as in Fig. characterized by a z-oriented linear filamentary current I(z) = I cos(kz)ẑ
PARTIAL NOTES for 6.1 Trigonometric Identities
 PARTIAL NOTES for 6.1 Trigonometric Identities tanθ = sinθ cosθ cotθ = cosθ sinθ BASIC IDENTITIES cscθ = 1 sinθ secθ = 1 cosθ cotθ = 1 tanθ PYTHAGOREAN IDENTITIES sin θ + cos θ =1 tan θ +1= sec θ 1 + cot
PARTIAL NOTES for 6.1 Trigonometric Identities tanθ = sinθ cosθ cotθ = cosθ sinθ BASIC IDENTITIES cscθ = 1 sinθ secθ = 1 cosθ cotθ = 1 tanθ PYTHAGOREAN IDENTITIES sin θ + cos θ =1 tan θ +1= sec θ 1 + cot
( )( ) ( ) ( )( ) ( )( ) β = Chapter 5 Exercise Problems EX α So 49 β 199 EX EX EX5.4 EX5.5. (a)
 hapter 5 xercise Problems X5. α β α 0.980 For α 0.980, β 49 0.980 0.995 For α 0.995, β 99 0.995 So 49 β 99 X5. O 00 O or n 3 O 40.5 β 0 X5.3 6.5 μ A 00 β ( 0)( 6.5 μa) 8 ma 5 ( 8)( 4 ) or.88 P on + 0.0065
hapter 5 xercise Problems X5. α β α 0.980 For α 0.980, β 49 0.980 0.995 For α 0.995, β 99 0.995 So 49 β 99 X5. O 00 O or n 3 O 40.5 β 0 X5.3 6.5 μ A 00 β ( 0)( 6.5 μa) 8 ma 5 ( 8)( 4 ) or.88 P on + 0.0065
ITU-R P (2009/10)
 ITU-R.45-4 (9/) % # GHz,!"# $$ # ITU-R.45-4.. (IR) (ITU-T/ITU-R/ISO/IEC).ITU-R http://www.tu.t/itu-r/go/patets/e. (http://www.tu.t/publ/r-rec/e ) () ( ) BO BR BS BT F M RA S RS SA SF SM SNG TF V.ITU-R
ITU-R.45-4 (9/) % # GHz,!"# $$ # ITU-R.45-4.. (IR) (ITU-T/ITU-R/ISO/IEC).ITU-R http://www.tu.t/itu-r/go/patets/e. (http://www.tu.t/publ/r-rec/e ) () ( ) BO BR BS BT F M RA S RS SA SF SM SNG TF V.ITU-R
Errata 18/05/2018. Chapter 1. Chapter 2
 Errata 8/05/08 Fundamentals of Neutrino Physics and Astrophysics C. Giunti and C.W. Kim Oxford University Press publication date: 5 March 007; 78 pages ± Lines are calculated before or after + the Anchor.
Errata 8/05/08 Fundamentals of Neutrino Physics and Astrophysics C. Giunti and C.W. Kim Oxford University Press publication date: 5 March 007; 78 pages ± Lines are calculated before or after + the Anchor.
Α Ρ Ι Θ Μ Ο Σ : 6.913
 Α Ρ Ι Θ Μ Ο Σ : 6.913 ΠΡΑΞΗ ΚΑΤΑΘΕΣΗΣ ΟΡΩΝ ΔΙΑΓΩΝΙΣΜΟΥ Σ τ η ν Π ά τ ρ α σ ή μ ε ρ α σ τ ι ς δ ε κ α τ έ σ σ ε ρ ι ς ( 1 4 ) τ ο υ μ ή ν α Ο κ τ ω β ρ ί ο υ, η μ έ ρ α Τ ε τ ά ρ τ η, τ ο υ έ τ ο υ ς δ
Α Ρ Ι Θ Μ Ο Σ : 6.913 ΠΡΑΞΗ ΚΑΤΑΘΕΣΗΣ ΟΡΩΝ ΔΙΑΓΩΝΙΣΜΟΥ Σ τ η ν Π ά τ ρ α σ ή μ ε ρ α σ τ ι ς δ ε κ α τ έ σ σ ε ρ ι ς ( 1 4 ) τ ο υ μ ή ν α Ο κ τ ω β ρ ί ο υ, η μ έ ρ α Τ ε τ ά ρ τ η, τ ο υ έ τ ο υ ς δ
General 2 2 PT -Symmetric Matrices and Jordan Blocks 1
 General 2 2 PT -Symmetric Matrices and Jordan Blocks 1 Qing-hai Wang National University of Singapore Quantum Physics with Non-Hermitian Operators Max-Planck-Institut für Physik komplexer Systeme Dresden,
General 2 2 PT -Symmetric Matrices and Jordan Blocks 1 Qing-hai Wang National University of Singapore Quantum Physics with Non-Hermitian Operators Max-Planck-Institut für Physik komplexer Systeme Dresden,
Figure A.2: MPC and MPCP Age Profiles (estimating ρ, ρ = 2, φ = 0.03)..
 Supplemental Material (not for publication) Persistent vs. Permanent Income Shocks in the Buffer-Stock Model Jeppe Druedahl Thomas H. Jørgensen May, A Additional Figures and Tables Figure A.: Wealth and
Supplemental Material (not for publication) Persistent vs. Permanent Income Shocks in the Buffer-Stock Model Jeppe Druedahl Thomas H. Jørgensen May, A Additional Figures and Tables Figure A.: Wealth and
r r t r r t t r t P s r t r P s r s r r rs tr t r r t s ss r P s s t r t t tr r r t t r t r r t t s r t rr t Ü rs t 3 r r r 3 rträ 3 röÿ r t
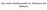 r t t r t ts r3 s r r t r r t t r t P s r t r P s r s r P s r 1 s r rs tr t r r t s ss r P s s t r t t tr r 2s s r t t r t r r t t s r t rr t Ü rs t 3 r t r 3 s3 Ü rs t 3 r r r 3 rträ 3 röÿ r t r r r rs
r t t r t ts r3 s r r t r r t t r t P s r t r P s r s r P s r 1 s r rs tr t r r t s ss r P s s t r t t tr r 2s s r t t r t r r t t s r t rr t Ü rs t 3 r t r 3 s3 Ü rs t 3 r r r 3 rträ 3 röÿ r t r r r rs
Problem Set 3: Solutions
 CMPSCI 69GG Applied Information Theory Fall 006 Problem Set 3: Solutions. [Cover and Thomas 7.] a Define the following notation, C I p xx; Y max X; Y C I p xx; Ỹ max I X; Ỹ We would like to show that C
CMPSCI 69GG Applied Information Theory Fall 006 Problem Set 3: Solutions. [Cover and Thomas 7.] a Define the following notation, C I p xx; Y max X; Y C I p xx; Ỹ max I X; Ỹ We would like to show that C
Section 9: Quantum Electrodynamics
 Physics 8.33 Section 9: Quantum Electrodynamics May c W. Taylor 8.33 Section 9: QED / 6 9. Feynman rules for QED Field content: A µ(x) gauge field, ψ(x) Dirac spinor Action Z» S = d x ψ(iγ µ D µ m)ψ Z
Physics 8.33 Section 9: Quantum Electrodynamics May c W. Taylor 8.33 Section 9: QED / 6 9. Feynman rules for QED Field content: A µ(x) gauge field, ψ(x) Dirac spinor Action Z» S = d x ψ(iγ µ D µ m)ψ Z
Durbin-Levinson recursive method
 Durbin-Levinson recursive method A recursive method for computing ϕ n is useful because it avoids inverting large matrices; when new data are acquired, one can update predictions, instead of starting again
Durbin-Levinson recursive method A recursive method for computing ϕ n is useful because it avoids inverting large matrices; when new data are acquired, one can update predictions, instead of starting again
Section 7.6 Double and Half Angle Formulas
 09 Section 7. Double and Half Angle Fmulas To derive the double-angles fmulas, we will use the sum of two angles fmulas that we developed in the last section. We will let α θ and β θ: cos(θ) cos(θ + θ)
09 Section 7. Double and Half Angle Fmulas To derive the double-angles fmulas, we will use the sum of two angles fmulas that we developed in the last section. We will let α θ and β θ: cos(θ) cos(θ + θ)
ω ω ω ω ω ω+2 ω ω+2 + ω ω ω ω+2 + ω ω+1 ω ω+2 2 ω ω ω ω ω ω ω ω+1 ω ω2 ω ω2 + ω ω ω2 + ω ω ω ω2 + ω ω+1 ω ω2 + ω ω+1 + ω ω ω ω2 + ω
 0 1 2 3 4 5 6 ω ω + 1 ω + 2 ω + 3 ω + 4 ω2 ω2 + 1 ω2 + 2 ω2 + 3 ω3 ω3 + 1 ω3 + 2 ω4 ω4 + 1 ω5 ω 2 ω 2 + 1 ω 2 + 2 ω 2 + ω ω 2 + ω + 1 ω 2 + ω2 ω 2 2 ω 2 2 + 1 ω 2 2 + ω ω 2 3 ω 3 ω 3 + 1 ω 3 + ω ω 3 +
0 1 2 3 4 5 6 ω ω + 1 ω + 2 ω + 3 ω + 4 ω2 ω2 + 1 ω2 + 2 ω2 + 3 ω3 ω3 + 1 ω3 + 2 ω4 ω4 + 1 ω5 ω 2 ω 2 + 1 ω 2 + 2 ω 2 + ω ω 2 + ω + 1 ω 2 + ω2 ω 2 2 ω 2 2 + 1 ω 2 2 + ω ω 2 3 ω 3 ω 3 + 1 ω 3 + ω ω 3 +
Jesse Maassen and Mark Lundstrom Purdue University November 25, 2013
 Notes on Average Scattering imes and Hall Factors Jesse Maassen and Mar Lundstrom Purdue University November 5, 13 I. Introduction 1 II. Solution of the BE 1 III. Exercises: Woring out average scattering
Notes on Average Scattering imes and Hall Factors Jesse Maassen and Mar Lundstrom Purdue University November 5, 13 I. Introduction 1 II. Solution of the BE 1 III. Exercises: Woring out average scattering
Απόκριση σε Μοναδιαία Ωστική Δύναμη (Unit Impulse) Απόκριση σε Δυνάμεις Αυθαίρετα Μεταβαλλόμενες με το Χρόνο. Απόστολος Σ.
 Απόκριση σε Δυνάμεις Αυθαίρετα Μεταβαλλόμενες με το Χρόνο The time integral of a force is referred to as impulse, is determined by and is obtained from: Newton s 2 nd Law of motion states that the action
Απόκριση σε Δυνάμεις Αυθαίρετα Μεταβαλλόμενες με το Χρόνο The time integral of a force is referred to as impulse, is determined by and is obtained from: Newton s 2 nd Law of motion states that the action
10.0 C N = = = electrons C/electron C/electron. ( N m 2 /C 2 )( C) 2 (0.050 m) 2.
 Electric Forces and Fields Section Review, p. 633 Givens Chapter 17 3. q 10.0 C q 10.0 C N 6.5 10 19 electrons 1.60 10 19 C/electron 1.60 10 19 C/electron Practice 17A, p. 636 1. q 1 8.0 C q 8.0 C r 5.0
Electric Forces and Fields Section Review, p. 633 Givens Chapter 17 3. q 10.0 C q 10.0 C N 6.5 10 19 electrons 1.60 10 19 C/electron 1.60 10 19 C/electron Practice 17A, p. 636 1. q 1 8.0 C q 8.0 C r 5.0
